Welcome to the Math Challenge Board

[Albert1]

HI : good to see you everybody ,I am a new comer on this board
Being a math teacher ,I always trained my students with various difficult problems
from now on I am going to post a sequential challenging questions for people to share
most of them I know the answer and the solution of it , maybe your solution is quicker and smarter (Yes)
please try it many thanks

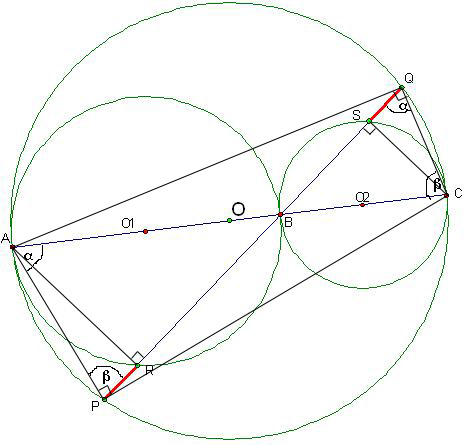
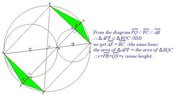
here comes the problem :

​

 

[Sudharaka]

Re: Prove PR=QS

Hi Albert, :)

The second sentence in your question isn't clear to me.

a line passing through point B and intersects two points \(P,\,Q\) with \(O\) and intersects \(R,\, S\) with \(O_1\) and \(O_2\), respectively prove: \(\overline{PR} = \overline{QS}\)

There are infinitely many lines passing though \(B\) and not all of them will have \(PR = QS\). Can you please elaborate a bit more on what you mean by this sentence.

Kind Regards,
Sudharaka.

 

[Albert1]

Re: Prove PR=QS

Can you plot a line passing through point B ,
satisfying the restriction and show that PR and QS are not equal ?

 

[Sudharaka]

Re: Prove PR=QS

Can you plot a line passing through point B ,
satisfying the restriction and show that PR and QS are not equal ?

I might be missing something here but what is meant by "the restriction"? Do you mean that \(O\) lies on the perpendicular bisector of \(PQ\) ?

 

[Albert1]

Re: Prove PR=QS

I might be missing something here but what is meant by "the restriction"? Do you mean that \(O\) lies on the perpendicular bisector of \(PQ\) ?

yes, that must be

 

[Sudharaka]

Re: Prove PR=QS

yes, that must be

But in that case there are infinitely many lines as I have claimed above, since for every chord of a circle the perpendicular bisector passes through the center of the circle. Refer >>this<<.

 

[Albert1]

Re: Prove PR=QS

the line passing through point B must also intersect with those of two small circles :
O1,and O2, at point R and S respectively as mentioned before

 

[Sudharaka]

Re: Prove PR=QS

the line passing through point B must also intersect with those of two small circles :
O1,and O2, at point R and S respectively as mentioned before

I think we are misunderstanding each other. To clarify further I have created this diagram using http://www.geogebra.org/cms/ and drawn two possible lines for \(PQ\).

 

[Albert1]

Re: Prove PR=QS

where is your points R1,S1 and R2,S2
we want to prove P1R1=Q1S1 , and P2R2=Q2S2

 

[Sudharaka]

Re: Prove PR=QS

I understand the question now. It seems that for all lines drawn through \(B\), \(PR=QS\). Sorry for the confusion. :)

I haven't found the proof yet, but I am trying...

 

[Opalg]

Re: Prove PR=QS

In the diagram, all the angles marked as right angles are angles in a semicircle (and therefore are indeed right angles). The two angles marked $\alpha$ are equal, as are the two angles marked $\beta$ (angles in the same segment). From the triangle $APC$, $AP = AC\cos\alpha$. From the triangle $ARP$, $PR = AP\cos\beta$. So $PR = AC\cos\alpha\cos\beta.$

In the same way, $CQ = AC\cos\beta$ (from triangle $AQC$) and $SQ = CQ\cos\alpha$ (from triangle $CSQ$), and so $SQ = AC\cos\alpha\cos\beta = PR.$

 

[Albert1]

Re: Prove PR=QS

Opalg:very good solution (Yes)

 

[Albert1]

Re: Prove PR=QS

here is my solution :
View attachment 699