What are the key parameters affecting heat transfer in thin tubes?

[MSM]

TL;DR Summary

Trying to understand how to approximate the time it takes for a fluid to reach the heated tube temperature

Hi,

I am trying to understand how I can estimate the time it takes for a fluid at room temperature flowing through a thin capillary glass tube (2 mm ID) connected to an oven to reach the equilibrium temperature (oven temperature). Assuming the oven is preheated and the tube inside the oven is equilibrated to the oven temperature (300 C)

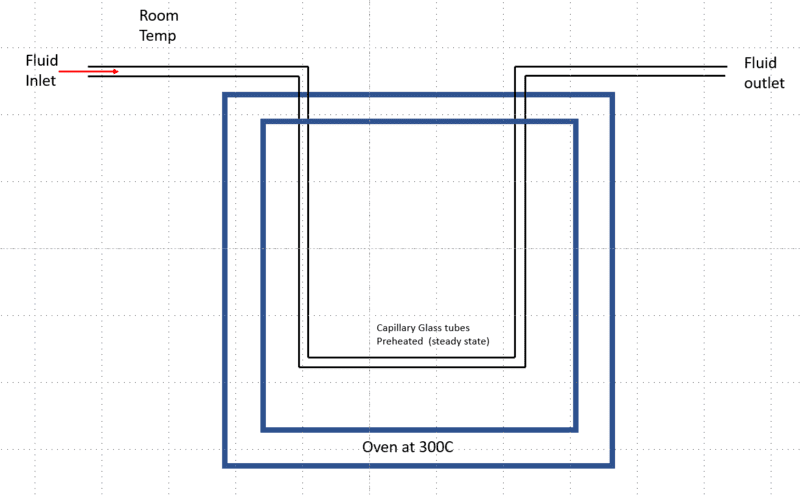
What I did was I assumed lumped capacitance model approximation and calculated the time. My understanding this would be a transient heat conduction problem, and this would be considered a constant flux since the tube inside the oven is already equilibrated to oven temperature before the fluid entering. Since this is a millimeter scale tube, I expected a millisecond time scale for fluid to reach oven temperature, but I got something in the 20+ second range What am I missing? I am only interested in an approximation, not exact numbers.

 

[Chestermiller]

Let's see your analysis.

 

[Dullard]

I may be belaboring the obvious, but:
The instantaneous rate of heat transfer from the oven (air) to the gas is proportional to the difference in those temperatures. The wall of the glass tube will 'restrict' the flow of heat by a factor dependent of the material properties and physical dimensions. Kinetics at the interior and exterior tube boundary is also a factor. Getting the gas temp to actually 'reach' oven temp takes (theoretically) forever. If you treat your arrangement as a number of discrete 'stages,' you'll note that more heat is transferred near the gas inlet and less near the outlet (this 'diminishing returns' situation is your basic problem). The nice thing about your setup is that you can vary the gas flow, measure the resulting temps, and characterize your system. Changes in gas density/velocity (due to probably-significant temp-related volume changes) will create non-idealities. I'm not sure why you would expect 'milliseconds' - glass is a lousy thermal conductor. A 'Lumped Capacitance Model' requires an assumption which would account for the conductivity of the glass - you were probably just way too optimistic.

 

[russ_watters]

If you treat your arrangement as a number of discrete 'stages,' you'll note that more heat is transferred near the gas inlet and less near the outlet (this 'diminishing returns' situation is your basic problem). The nice thing about your setup is that you can vary the gas flow, measure the resulting temps, and characterize your system. Changes in gas density/velocity (due to probably-significant temp-related volume changes) will create non-idealities. I'm not sure why you would expect 'milliseconds' - glass is a lousy thermal conductor. A 'Lumped Capacitance Model' requires an assumption which would account for the conductivity of the glass - you were probably just way too optimistic.

Yeah, as an engineer who hates math, I'd definitely do this with a spreadsheet where it is easy to do a hundred or thousand steps. And yeah, while that makes the calculation itself easy, figuring out the coefficients is near impossible. Better if it can be tested to calibrate the model.

 

[MSM]

Let's see your analysis.

I tried the lumped system analysis, but my understanding is that for this method to be valid, Biot Number (Bi) has to be less than 0.1 (Bi<0.1).

Now consider a liquid fluid with thermal conductivity k=0.14 W/m K, and heat capacity Cp= 2500 J/kg K, and density of 780 kg/m3.
Now for long pipes, Nu=3.68, and with a tube internal diameter of 2 mm, I get a heat transfer coefficient of 257 W/m2 K. This gives us a Bi=0.9. (low but not lower than 0.1)

Now with this information , I get a thermal time constant of ~3 s^-1. and t~17 seconds to reach final temperature. Does this seem reasonable? because I expected few seconds or lower

 

[Chestermiller]

Are you asking how long the tube has to be for the average fluid temperature to closely approach the oven temperature? Also, where did you get the 3.68 from? Also, when you refer to the Biot number, you are referring to the external heat transfer resistance (on the outside of the tube), right?

 

[MSM]

Are you asking how long the tube has to be for the average fluid temperature to closely approach the oven temperature? Also, where did you get the 3.68 from? Also, when you refer to the Biot number, you are referring to the external heat transfer resistance (on the outside of the tube), right?

Maybe the schematic I drew is not really a good representation, but the tube is long (coiled) and is about few meters. What I want to know is the time required for the fluid in the tubes to reach the oven temperature once it enters the oven. Basically, it is a chemical reagent that reacts once reaching the set temperature, and I want to estimate how long it takes (time) to reach that temperature once it enters.
The Nusselt number I got is for convection with uniform temperature for circular tubes (Nu=3.68), and the calculated Bi to assume a lumped system. This is only way I found to calculate the heat transfer coefficient. Maybe my approach is not correct, but that's where I am at right now

 

[Chestermiller]

I don't think you approaching this quite properly. You appear to be concerned with the temperature variation of the fluid along the tube when the system is operating at steady state. The equation to be used would be $$WC_p\frac{dT}{dx}=U(\pi D)(T_0-T)$$where U is the overall heat transfer coefficient (including convective resistance outside the tube), D is the tube diameter, W is the mass flow rate, T is the cross section average temperature, To is the bulk gas temperature in the oven, and x is the distance along the tube. In terms of mean residence time in the tube, this becomes: $$\frac{dT}{dt}=\frac{T_0-T}{\tau}$$where the characteristic time $\tau$ is given by: $$\tau=\frac{\rho C_p D}{4U}$$with v being equal to the cross sectional average axial velocity of the fluid in the tube. Neglecting the conductive resistance of the tube wall, the overall heat transfer coefficient U is essentially given by $$\frac{1}{U}=\frac{1}{h_{in}}+\frac{1}{h_{out}}$$where $h_{in}$ is the heat transfer coefficient for the fluid inside the tube and $h_{out}$ is the gas side heat transfer coefficient from the tube wall to the bulk oven temperature. Bird, Stewart, and Lightfoot give typical values of $h_{out}$ in the range 3-20 W/m^2K.

For laminar flow inside the tube at a constant wall temperature, Bird, Stewart, and Lightfoot give the asymptotic Nussult number as 3.66, rather than 3.68. But, for the more accurate case (in this situation) of a constant wall heat flux, they give an asymptotic Nu of 4.36. Still I think you are going to find that the limiting resistance to heat transfer in your system resides on the outside of the tube.

Thoughts so far?

 

[MSM]

I am not looking for the temperature profile along the tube. Although, in reality there should be a variation of temperature along the the tube. I am assuming a homogenous temperature distribution in the fluid and just need an approximate time frame for the fluid to reach the final temperature and plot T vs time.

I will go over your calculations and see how much it is different if I consider the overall heat transfer coefficient

 

[Chestermiller]

I am not looking for the temperature profile along the tube. Although, in reality there should be a variation of temperature along the the tube. I am assuming a homogenous temperature distribution in the fluid and just need an approximate time frame for the fluid to reach the final temperature and plot T vs time.

I will go over your calculations and see how much it is different if I consider the overall heat transfer coefficient

So you are assuming that all the fluid is at the lower starting temperature, and you are just inserting the tube into the oven (without the fluid flowing), and looking at the transient heating of the cylinder of fluid?

 

[MSM]

So you are assuming that all the fluid is at the lower starting temperature, and you are just inserting the tube into the oven (without the fluid flowing), and looking at the transient heating of the cylinder of fluid?

No, the tubes are connected to a pump outside the oven and then the fluid flows inside. I am aware that as the fluid flows you will have a temperature distribution along the tube inside. However, I am only interested in an approximation of the thermal time scale (the approximate time that the flowing fluid will reach the maximum temperature on average once entering) Do you think this is reasonable or I need more thorough transient analysis with all factors?

 

[Chestermiller]

No, the tubes are connected to a pump outside the oven and then the fluid flows inside. I am aware that as the fluid flows you will have a temperature distribution along the tube inside. However, I am only interested in an approximation of the thermal time scale (the approximate time that the flowing fluid will reach the maximum temperature on average once entering) Do you think this is reasonable or I need more thorough transient analysis with all factors?

With respect, I think the analysts I presented should be closer to what you are looking for.

 

[MSM]

With respect, I think the analysts I presented should be closer to what you are looking for.

What if you treat this problem as a flow in a tube with constant surface heat flux. and set Nu=4.36, calculate h, and then solve for dT/dt. How would this be different ? and are you really going to have order of magnitudes error if you solve it this way?
I appreciate your input on all of this

 

[Chestermiller]

What if you treat this problem as a flow in a tube with constant surface heat flux. and set Nu=4.36, calculate h, and then solve for dT/dt. How would this be different ? and are you really going to have order of magnitudes error if you solve it this way?
I appreciate your input on all of this

The dominant heat transfer resistance is going to be on the outside of the tube, and I would assume an overall heat transfer coefficient of 20 W/m^2K. Based on the equations I gave in my analysis, that would correspond to a characteristic time of about 50 seconds. So that would require a mean residence time of about 4(50)= 200 sec, or on the order of about 3 minutes. What volumetric flow rate do you envision using, and how long is the tube?

 

[MSM]

The dominant heat transfer resistance is going to be on the outside of the tube, and I would assume an overall heat transfer coefficient of 20 W/m^2K. Based on the equations I gave in my analysis, that would correspond to a characteristic time of about 50 seconds. So that would require a mean residence time of about 4(50)= 200 sec, or on the order of about 3 minutes. What volumetric flow rate do you envision using, and how long is the tube?

I did the calculations and I got a characteristic time of about 49 seconds as well, which translates to 244s to reach 99% of the final temperature However, that doesn't agree with what is happening in practice. The fluid is basically a reactant that reacts once exposed to high temperature. In this case, the reactant at room temperature enters the oven and we have the oven temperature set at approx. 300 C. The reaction initiates at around 250C, which when observed happens very quickly after the reactant enters. (few seconds)

But the calculation shows that the fluid reaches 250C after about ~80 seconds, which is for sure not the case. Otherwise, the reaction wouldn't initiate after few seconds of the fluid entering. I am not sure what is missing here.

I might have not noted this but the tubes have an inner diameter of about 2 mm, and a wall thickness of 0.7 mm, where the tube's thermal conductivity is ~1.4 W/m K (glass)

 

[Chestermiller]

I did the calculations and I got a characteristic time of about 49 seconds as well, which translates to 244s to reach 99% of the final temperature However, that doesn't agree with what is happening in practice. The fluid is basically a reactant that reacts once exposed to high temperature. In this case, the reactant at room temperature enters the oven and we have the oven temperature set at approx. 300 C. The reaction initiates at around 250C, which when observed happens very quickly after the reactant enters. (few seconds)

But the calculation shows that the fluid reaches 250C after about ~80 seconds, which is for sure not the case. Otherwise, the reaction wouldn't initiate after few seconds of the fluid entering. I am not sure what is missing here.

I might have not noted this but the tubes have an inner diameter of about 2 mm, and a wall thickness of 0.7 mm, where the tube's thermal conductivity is ~1.4 W/m K (glass)

I'm trying to help you and I keep asking for information, but you don't seem forthcoming, and I'm getting a little frustrated. Please help me help you.

What is the length of the tubing?
What is the flow rate of the fluid (mass flow rate)?
Is that 2 mm the inside diameter?
Is there a fan in the oven to agitate the air, or is the oven air static?

What benchmarking have you done on the heat transfer, such as flowing a non-reacting fluid through the tubing, and measuring the outlet temperature as a function of the mass flow rate?

What do you know quantitatively about the kinetics of the reaction, besides that it starts reacting at 250 C? Are there any kinetics data on the reaction rate as a function of concentration? What is the actual chemical reaction occurring?