- #1
not_famous_user
- 1
- 0
Hello, does anyone can help me with these tasks:
I have no idea how to solve it
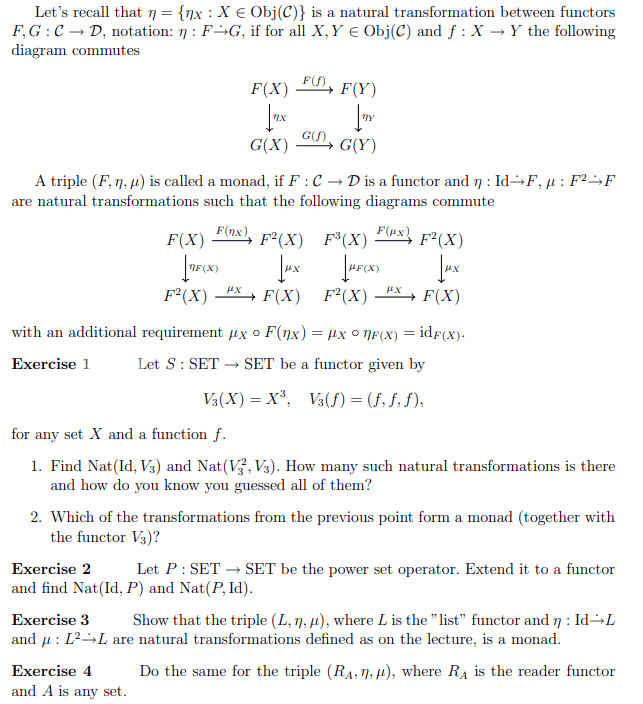
I have no idea how to solve it
A functor is a mathematical concept used in category theory to describe the relationship between two categories. It is a mapping between objects and morphisms of one category to objects and morphisms of another category, while preserving the structure and composition of the morphisms. Functors are used to analyze and compare different categories, and are essential in understanding the relationships between them.
A covariant functor preserves the direction of morphisms between categories, while a contravariant functor reverses the direction of morphisms. In other words, a covariant functor maps objects and morphisms from one category to another in the same direction, while a contravariant functor maps them in the opposite direction.
Functors are important in category theory because they allow us to study and compare different categories, which can help us understand the underlying structure and relationships between them. They also provide a way to translate concepts and ideas from one category to another, making it easier to apply knowledge from one area to another.
One example of a functor is the forgetful functor, which maps objects and morphisms from a category to a subcategory by "forgetting" some of the structure or properties of the original category. For example, the forgetful functor from the category of groups to the category of sets maps each group to its underlying set and each group homomorphism to its underlying function.
A natural transformation is a mapping between two functors that preserves the structure and composition of morphisms. In other words, it is a way to transform one functor into another while maintaining the relationships between objects and morphisms. Natural transformations play an important role in category theory, as they allow us to compare and relate different functors and categories.