barryj
- 856
- 51
What geometry theorem is used to be able to state that 8/4 = x/6 ??
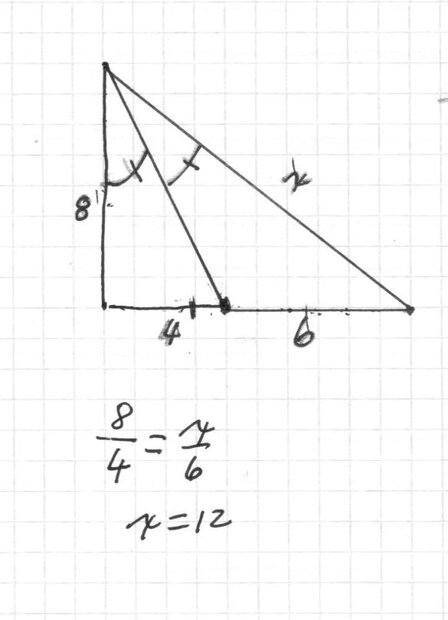
What exactly have you shown? That there exists some triangle that satisfies that ratio?barryj said:It is not a right triangle. I worked backwards assuming x is 12 and then calculated the angles that are equal and they are, 27.8858 deg (using law of cosines). The angle that looks like a right angle is in fact 82.8192 deg. I know there is some geometry theorem that stats what I have shown but I hve not found it. It is some sort of triangle bisected vertex theorem? The numbers 8,4,6, and 12 are correct.
I was told that the answer was 12. I just wanted to see if this was true. The theorem is called the triangle angle bisector theorem.Office_Shredder said:What exactly have you shown? That there exists some triangle that satisfies that ratio?
"So the areas of the triangles also have ratios as those sides i.e. x/8. Thus 6/4=x/8." It is not obvious to me that this is true.martinbn said:https://en.wikipedia.org/wiki/Angle_bisector_theorem
I think the the prove I wrote is more natural, but then it may seem that way to me because that's what came to mind first.
I think the proof of this is probably the same or closely related to the proof of the angle bisector theorem. I looked at those proofs in the link. If any of those proofs are obvious to you, then you are a smarter man than me. ;-)barryj said:"So the areas of the triangles also have ratios as those sides i.e. x/8. Thus 6/4=x/8." It is not obvious to me that this is true.
My geometry book does have the proof of the triangle angle bisector theorem but it is a bit complicated. I may, I repeat, may try to understand it at a later time. Right now I will just remember the theorem.FactChecker said:I think the proof of this is probably the same or closely related to the proof of the angle bisector theorem. I looked at those proofs in the link. If any of those proofs are obvious to you, then you are a smarter man than me. ;-)
You are wrong. The drawing is correct. You should NEVER assume things. If the drawing does not say it is a right triangle, then do not assume it is.Vanadium 50 said:The drawing is very, very poor. You're very lucky that people were able to figure out your question.
- You should not draw a right triangle if the triangle is not supposed to be right.,
- As I understand it, the line from the top vertex to the base divides divides the base into 4 and 6. The tick mark you drew to the right of the 4 and to the left of the angle bisector is irrelevant.
If you want to do well in geometry, you need to be more careful in your drawings. If you want help - not just in geometry, but in life - you need to make it easy for people to help you. Making them work hard because you didn't spend the time to express yourself clearly is ineffective. (Some might even say disrespectful.)
Good luck in life with that attitude.barryj said:You are wrong. The drawing is correct. You should NEVER assume things. If the drawing does not say it is a right triangle, then do not assume it is.
Well, when this problem was presented to my student ( I am a tutor) he worked it immediately. I did not recall the theorem he was using and this is why I posted on this forum. In fact, it took me a while to find the theorem in my geometry book as I did not know the proper name for it. I am surprised that the people on this forum had an issue with my question. I would have expected an answer like, "this is the triangle angle bisector theorem" but did not get it. Anyway, my attitude3 has been a benefit to me for many years.phinds said:Good luck in life with that attitude.
Then you understand the need for an accurate drawing and a well-stated question.barryj said:I am a tutor)
Well, the drawing must be accurate because my 15 year old student worked the problem in about 15 seconds. Go figure.Vanadium 50 said:Then you understand the need for an accurate drawing and a well-stated question.
No, he depends on lucky guesses by some students. Obviously you are just not intuitive enoughVanadium 50 said:Then you understand the need for an accurate drawing and a well-stated question.
The drawing was accurate. As it turns out, what looks to be a right angle is in fact about 83 degrees.phinds said:No, he depends on lucky guesses by some students. Obviously you are just not intuitive enough
Not so much.barryj said:The drawing was accurate.
So, you are saying that when solving a geometry problem I should get out my calipers and draw the diagram with precision?? No way. A general diagram is all that is necessary.Vanadium 50 said:Not so much.
Another reason to draw an accurate picture is that it's the first step in solving the problem. You learn about the elements and relationships when trying to draw it. This angle needs to be acute, that angle needs to be obtuse, this line segment has to be shorter than that line segment, etc.
While I agree "these lines look congruent" is not a proof, but that does not mean that any random grouping of lines makes a good drawing.
The point is that from the begining you don't know what that angle is, so why draw it so close to a right angle!barryj said:So, you are saying that when solving a geometry problem I should get out my calipers and draw the diagram with precision?? No way. A general diagram is all that is necessary.
If you knew the theorem then it would not matter if the angle was close to a right angle or not. The surprising fact is that the "experts" here did not know the theorem otherwise they would have merely refreshed my memory of it.martinbn said:The point is that from the begining you don't know what that angle is, so why draw it so close to a right angle!
Well, yes, it certainly is true that if you know the answer in advance then a correctly drawn figure has less relevance, it's just an annoyance.barryj said:If you knew the theorem then it would not matter if the angle was close to a right angle or not.
The answer was not known in advance. One had to use the knowledge of the theorem to get the answer. The angle could be drawn as a right angle, an acute angle, or an obtuse angle. It would not make any difference in the solution.phinds said:Well, yes, it certainly is true that if you know the answer in advance then a correctly drawn figure has less relevance, it's just an annoyance.
Don't put words in my mouth. It';s the cheapest form of debate.barryj said:So, you are saying
Actually, it was not my diagram. I copied it out of a geometry textbook.Vanadium 50 said:Don't put words in my mouth. It';s the cheapest form of debate.
My major pointy is that if you want help, you should make it easy for them to help you. Careless diagrams don't do that. My minor point is that a good drawing is the starting point of a good solution. You may sneer at this, but a) that doesn't make it any less true, and b) you are the one asking for help after all.
Ok.barryj said:You are wrong. The drawing is correct. You should NEVER assume things. If the drawing does not say it is a right triangle, then do not assume it is.
No, there is a 120 degree angle at the 4/6 intersection and the lines do not meet at the same point. They are separated by 2 inches. This thread is getting silly. I am gone!FactChecker said:Ok.
Should we assume that the baseline is one straight line or is there an angle at the vertex between the 4 and 6 length lines?
Should we assume that all those lines to the top meet at the same point? It looks a little like the line farthest to the right comes in at a different point.
Of course, I was being sarcastic, but I actually thought it was a right angle until I did the calculation and didn't get 12.barryj said:No, there is a 120 degree angle at the 4/6 intersection and the lines do not meet at the same point. They are separated by 2 inches. This thread is getting silly. I am gone!
You had best get another calculator.FactChecker said:Of course, I was being sarcastic, but I actually thought it was a right angle until I did the calculation and didn't get 12.
My calculator is fine. If that was a right angle, the length of ##x## would be 12.8062484748657.barryj said:You had best get another calculator.