What Happens When the Ladder Operator Hits the Identity Operator?

[binbagsss]

Homework Statement

below

Relevant Equations

below

I'm just trying to follow the below

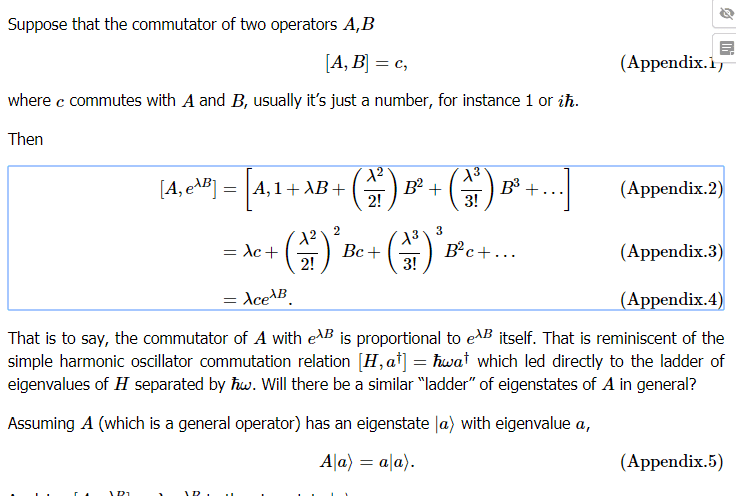
And I understand all, I think, except what's happened to the term when A hits 1: [A,1] ?
If I'm correct basically we're just hitting on the first operator so reducing the power by one each time of the operator in the right hand bracket

thanks

 

[tnich]

If I understand this correctly, $1$ is the identity operator. So what would be the value of $[A,1]$?

 

[Abhishek11235]

[A,1]=0 since 1 is pure number!

 

[binbagsss]

[A,1]=0 since 1 is pure number!

omg i think i need some sleeep ! thank you haha :)

 

[Abhishek11235]

omg i think i need some sleeep ! thank you haha :)

A typo! 1 here refers to identity operator as @tnich says. Anyways,final result is same