What is considered the "System" here? (conservation of energy problem)

[simphys]

Homework Statement

see picture please

Relevant Equations

nothing

So in this picture friction is also assumed, but if we assume friction as an energy don't we need to consider the system to be earth, roller-coaster car, roller-coaster itself(due to friction force)? I actually still had this question from a previous coverage of this material lol...

I just understood that the difference between W-E principle and conservation of energy is that we simply consider the work done by the 'external' forces (if solely the object is considered) from $W_net=changeof K$ in terms of energies itself (if system is considered as a whole) divided into NC and C forces of course.
i.e. two different points of view for the problem. #howamazingisthat :P
I always looked at it as it being a different way to solve the problem but not that it's an acutal substitute of the work done into a form of energy.

anyways... here is the question

 

[BvU]

Homework Statement:: see picture please

It's pretty useless. Don't be lazy: type the problem statement and your attempt at solution.

And read back what you post.

$\$

 

[simphys]

It's pretty useless. Don't be lazy: type the problem statement and your attempt at solution.

And read back what you post.

$\$

What do you mean about lazy?? it's really a simple question that doesn't need any calculation? :)
My question is why don't we considered the roller-coaster (for the friction force) as well to be compliant with the law of conservation of energy. Instead only the roller-coaster car and the Earth is considered as being the system

 

[haruspex]

Homework Statement:: see picture please
Relevant Equations:: nothing

don't we need to consider the system to be earth, roller-coaster car, roller-coaster itself(due to friction force)?

You can consider the friction on the car as an external force on the car+earth system.

Homework Statement:: see picture please
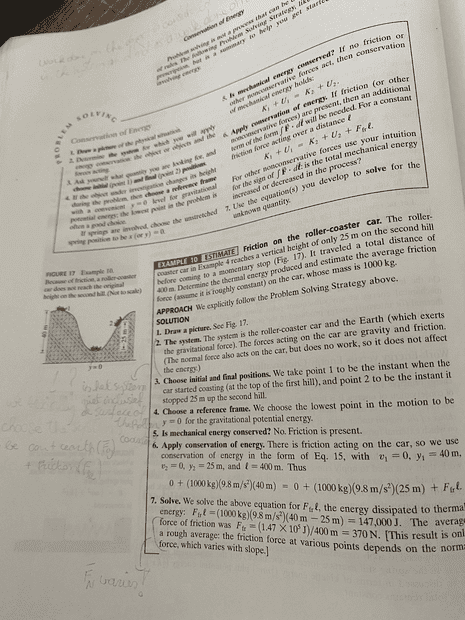
Relevant Equations:: nothing

the difference between W-E principle and conservation of energy is that we simply consider the work done by the 'external' forces (if solely the object is considered) from Wnet=changeofK in terms of energies itself (if system is considered as a whole)

I've read that ten times and still don’t get what it means. Work done = change in KE applies to rigid bodies, not the car+earth system.

 

[simphys]

You can consider the friction on the car as an external force on the car+earth system.

I've read that ten times and still don’t get what it means. Work done = change in KE applies to rigid bodies, not the car+earth system.

and what if I said that it is a thermal energy, would it be included into the system? (obviously not practical) but just wondeirng.
edit: clarification. Let me rephrase:
What if I said that the work done by the friction force is a thermal energy, do I consider the roller-coaster also to be included in the (car+earth) system?

Haha, yeah nvm I'll delete it, hahaha indeed the object itself is what I meant, not the system. What I meant was that I was always looking at the conservation of energy as a totally different approach to solve the problem, but it is a change of work done by forces (from the work done = change in KE) into either potential energy for conservative forces or work done(/thermal energy) for nonconservative forces and then equate that to KE which gives us the law basically.

Because of not understanding this I was kind of struggling on getting electric PE on an intuitive level. It all comes down to the fact that the coulomb force (or lorentz force more generally) is seen as a conservative force

 

[haruspex]

What if I said that the work done by the friction force is a thermal energy, do I consider the roller-coaster also to be included in the (car+earth) system?

No, by definition, the car+earth system does not include the roller coaster structure. And the work done by the friction on the system is negative KE.

 

[simphys]

No, by definition, the car+earth system does not include the roller coaster structure. And the work done by the friction on the system is negative KE.

Could you please tell me why by definition? Because it just said it like this potential energy is a property of the system chosen (system = collection of objects that we choose to study) But nothing really on how or why it can't be chosen. and oh yeah due to the movement of the particles internally got it.

 

[hmmm27]

If all you're interested in is the PE and KE of the car in relation to the Earth there's no point in including the trackwork.

If you order a delivery pizza, which company advertises "30 min or free", your interest is in the elapsed time, not the traffic, nor competence of the driver, nor anything else that can affect it.

@OP I noticed in your scribbles that you question why the Normal force changes as the car rolls along... has that been cleared up ?

 

[Orodruin]

What do you mean about lazy?? it's really a simple question that doesn't need any calculation? :)

It is not about being an easy question or not, it is about attaching an image of the problem instead of typing it out. Even if it was a high quality image that was easy to read (it is not) there are several issues with this in terms of quoting text in replies etc (and it is also not in line with the homework guidelines). If you expect people to spend time to help you for free, the least you can do is to not make it harder for them to do so by being lazy.

 

[vela]

What if I said that the work done by the friction force is a thermal energy, do I consider the roller-coaster also to be included in the (car+earth) system?

Yes, because friction will heat up both the car and track. The conservation of energy equation would be
$$K_{\rm i, car} + U_{\rm i, car} = K_{\rm f, car} + U_{\rm f, car} + \Delta E_{\rm th, car} + \Delta E_{\rm th, track}.$$ Friction would be the mechanism by which mechanical energy is turned into thermal energy.

If all you really care about is that the energy dissipated by friction reduces the mechanical energy of the car, you can leave the track out of it, ignore the increases in thermal energy, and just talk about the work done by friction. That's how the book solved the problem.

 

[haruspex]

Could you please tell me why by definition?

Because that is what "car+earth system" means. You could choose a car+earth+structure system, but the author has chosen just car+earth. You cannot dispute someone else's choice of system. You can dispute whether it is the best choice, and you can dispute the equations they write concerning it.

Now, strictly speaking, there is some tiny gravitational attraction between the car and the structure, but we can safely ignore that. So the variation in potential energy is in the relative positions of car and earth. The author wishes to make that internal energy, so takes car+earth as the system. But she wants the friction to be an external force, so leaves out the structure.

 

[simphys]

It is not about being an easy question or not, it is about attaching an image of the problem instead of typing it out. Even if it was a high quality image that was easy to read (it is not) there are several issues with this in terms of quoting text in replies etc (and it is also not in line with the homework guidelines). If you expect people to spend time to help you for free, the least you can do is to not make it harder for them to do so by being lazy.

right. It has nothing to do with laziness, just my evaluation of the situation deciding to put up the picture as it'd be easier to reference. And on which I didn't notice that the image was compressed causing the lower quality. I will do that next time.

May I ask what you mean with the quoting text part please? if you meant the W-E principle eq. I didn't know how to put up those supscripts indeed.

 

[simphys]

Yes thank you. It was from the previous time around.

If all you're interested in is the PE and KE of the car in relation to the Earth there's no point in including the trackwork.

If you order a delivery pizza, which company advertises "30 min or free", your interest is in the elapsed time, not the traffic, nor competence of the driver, nor anything else that can affect it.

@OP I noticed in your scribbles that you question why the Normal force changes as the car rolls along... has that been cleared up ?

Yes thank you. It was from the previous time around.

Yes, because friction will heat up both the car and track. The conservation of energy equation would be
$$K_{\rm i, car} + U_{\rm i, car} = K_{\rm f, car} + U_{\rm f, car} + \Delta E_{\rm th, car} + \Delta E_{\rm th, track}.$$ Friction would be the mechanism by which mechanical energy is turned into thermal energy.

If all you really care about is that the energy dissipated by friction reduces the mechanical energy of the car, you can leave the track out of it, ignore the increases in thermal energy, and just talk about the work done by friction. That's how the book solved the problem.

Thank you, that was unfortunately not really mentioned and caused my confusion previous time around..

It'd be more suiteable then to only incoorporate it as an energy if you can actually reutilize it afterwards I presume (more complex processes)

 

[simphys]

Because that is what "car+earth system" means. You could choose a car+earth+structure system, but the author has chosen just car+earth. You cannot dispute someone else's choice of system. You can dispute whether it is the best choice, and you can dispute the equations they write concerning it.

Now, strictly speaking, there is some tiny gravitational attraction between the car and the structure, but we can safely ignore that. So the variation in potential energy is in the relative positions of car and earth. The author wishes to make that internal energy, so takes car+earth as the system. But she wants the friction to be an external force, so leaves out the structure.

thank you, it comes down to personal judgement for such situation, got it thanks.
And now that you mentioned the second part.
Let's say I have a mass and a spring right. Taking the mass-spring system, and assume that the spring is massless.
Which energies are we then able to neglect (from the spring)?

• KE because of no mass alright(mentioned in the book). But, if it was considered what would its speed be the same as the mass or not?
• and for Potential energy of the spring (not mentioned in the book that's why I'm double checking), will also be ignored due to no mass.

 

[Orodruin]

It has nothing to do with laziness, just my evaluation of the situation deciding to put up the picture as it'd be easier to reference.

This is exactly the point. It is not easier to reference as we cannot quote particular parts of the text. Just as I am doing now.

I didn't know how to put up those supscripts indeed.

https://www.physicsforums.com/help/latexhelp/

 

[simphys]

This is exactly the point. It is not easier to reference as we cannot quote particular parts of the text. Just as I am doing now.https://www.physicsforums.com/help/latexhelp/

yep, got it thank you. Will do that next time :)
And thank you I will check that it.

 

[haruspex]

if it was considered what would its speed be the same as the mass or not?

You have not completely specified the arrangement. Are you starting with a free standing spring on the ground, with a mass dropped on top?

 

[simphys]

sorry I was talking sideways in the x-direction because otherwise there would be grav PE

 

[haruspex]

sorry I was talking sideways in the x-direction because otherwise there would be grav PE

A spring is not a rigid body. You would have to consider how the mass elements of the spring move. It could even happen that the spring does not expand uniformly, leading to waves propagating along it.

 

[haruspex]

and for Potential energy of the spring (not mentioned in the book that's why I'm double checking), will also be ignored due to no mass.

Which potential energy? If it is sideways then presumably you do not mean GPE, but you obviously can't ignore EPE (elastic PE).

 

[simphys]

Which potential energy? If it is sideways then presumably you do not mean GPE, but you obviously can't ignore EPE (elastic PE).

I thin grav PE(for the spring) was assumed because I was talking about it in the y-dir not the x-dir. as stated, but anyways doesn't really matter my apologies. it was more the KE I was not so sure about how it would be involved once taken into acount.

A spring is not a rigid body. You would have to consider how the mass elements of the spring move. It could even happen that the spring does not expand uniformly, leading to waves propagating along it.

Oh okay, so that's basically a more advanced treatment hence why we ignore the mass of the spring.