J O Linton
- 76
- 11
- TL;DR Summary
- A formula is derived for the force associated with cosmological expansion and for the size of the sphere inside which gravity prevails
In considering the excellent answers given to my previous query about a photon in a box, I was led to consider what force was needed to hold the walls of the box stationary (w.r.t. an inertial observer inside the box.).
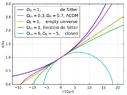
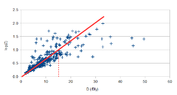
If you place a mass m on the end of a very long string of inextensible length D, I believe the tension in the string will be ## 2mD/T_0^2 ## where ##T_0## is the age of the universe (this is assuming that the expansion of the universe has been linear throughout). If we compare this force to the force of gravity exerted on the mass by a galaxy of mass M then we find that the distance at which the two are equal (and opposite) is given by the expression ## D = \sqrt[3]{GMT_0^2/2} ##. Putting in the appropriate figure for the Milky Way, this gives a distance of 2.8 million light years - a fugure which is consistent with the fact that the Andromeda galaxy is 2.5 million light years distant and is part of our Local Group.
Can someone confirm my analysis - and is there an official name for the distance at which gravity and the cosmological force are equal?
If you place a mass m on the end of a very long string of inextensible length D, I believe the tension in the string will be ## 2mD/T_0^2 ## where ##T_0## is the age of the universe (this is assuming that the expansion of the universe has been linear throughout). If we compare this force to the force of gravity exerted on the mass by a galaxy of mass M then we find that the distance at which the two are equal (and opposite) is given by the expression ## D = \sqrt[3]{GMT_0^2/2} ##. Putting in the appropriate figure for the Milky Way, this gives a distance of 2.8 million light years - a fugure which is consistent with the fact that the Andromeda galaxy is 2.5 million light years distant and is part of our Local Group.
Can someone confirm my analysis - and is there an official name for the distance at which gravity and the cosmological force are equal?