Lambda96
- 233
- 77
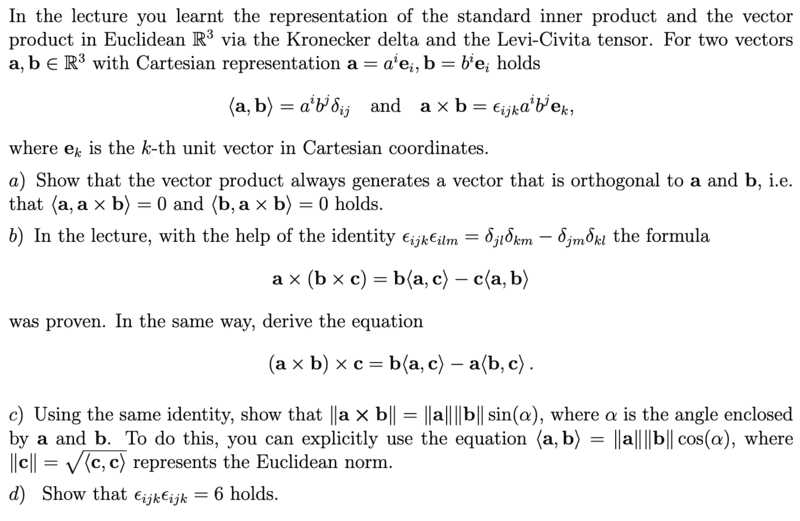
- Homework Statement
- Use the identity ##\epsilon_{ijk} \epsilon_{ilm} = \delta_{jl} \delta_{km} - \delta_{jm} \delta_{kj}## to proof ##||\vec{a} \times \vec{b}||=||\vec{a}|| \cdot ||\vec{b}|| \sin{\alpha}##
- Relevant Equations
- ##\epsilon_{ijk} \epsilon_{ilm} = \delta_{jl} \delta_{km} - \delta_{jm} \delta_{kj}##
Hi everyone,
I'm having problems with task c
In the task, the norm has already been defined, i.e. ##||\vec{c}||=\sqrt{\langle \vec{c}, \vec{c} \rangle }## I therefore first wanted to calculate the scalar product of the cross product, i.e. ##\langle \vec{a} \times \vec{b} , \vec{a} \times \vec{b} \rangle## first
$$\langle \vec{a} \times \vec{b} , \vec{a} \times \vec{b} \rangle = \epsilon_{ijk}a^{i}b^{j} \vec{e}_k \cdot \epsilon_{ijk}a^{i}b^{j} \vec{e}_k$$
$$\langle \vec{a} \times \vec{b} , \vec{a} \times \vec{b} \rangle = \epsilon_{ijk}a^{i}b^{j} \cdot \epsilon_{ijk}a^{i}b^{j}$$
$$\langle \vec{a} \times \vec{b} , \vec{a} \times \vec{b} \rangle = \epsilon_{ijk} \epsilon_{ijk} ||a^{i}||^2 ||b^{j}||^2 $$
If I look at my calculation now, I've definitely made a mistake, but I don't know how else to arrive at the desired result.
I know I didn't use the identity ##\epsilon_{ijk} \epsilon_{ilm} = \delta_{jl} \delta_{km} - \delta_{jm} \delta_{kj}##, but if I take the scalar product with itself, the epsilon would be ##\epsilon_{ijk} \epsilon_{ijk}## and not ##\epsilon_{ijk} \epsilon_{ilm}##, or not?
I'm having problems with task c
In the task, the norm has already been defined, i.e. ##||\vec{c}||=\sqrt{\langle \vec{c}, \vec{c} \rangle }## I therefore first wanted to calculate the scalar product of the cross product, i.e. ##\langle \vec{a} \times \vec{b} , \vec{a} \times \vec{b} \rangle## first
$$\langle \vec{a} \times \vec{b} , \vec{a} \times \vec{b} \rangle = \epsilon_{ijk}a^{i}b^{j} \vec{e}_k \cdot \epsilon_{ijk}a^{i}b^{j} \vec{e}_k$$
$$\langle \vec{a} \times \vec{b} , \vec{a} \times \vec{b} \rangle = \epsilon_{ijk}a^{i}b^{j} \cdot \epsilon_{ijk}a^{i}b^{j}$$
$$\langle \vec{a} \times \vec{b} , \vec{a} \times \vec{b} \rangle = \epsilon_{ijk} \epsilon_{ijk} ||a^{i}||^2 ||b^{j}||^2 $$
If I look at my calculation now, I've definitely made a mistake, but I don't know how else to arrive at the desired result.
I know I didn't use the identity ##\epsilon_{ijk} \epsilon_{ilm} = \delta_{jl} \delta_{km} - \delta_{jm} \delta_{kj}##, but if I take the scalar product with itself, the epsilon would be ##\epsilon_{ijk} \epsilon_{ijk}## and not ##\epsilon_{ijk} \epsilon_{ilm}##, or not?