- #1
DivergentSpectrum
- 149
- 15
so i was messing around and found that
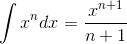
is always true.
taking the integrand as x-1
at first we have
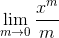
but the limit is 2 sided(as m approaches the positive side of zero i get a positive value, while as m approaches the negative side of zero, i get a negative value.)
So i decided to find the average of both sides of the limit:
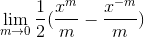
i graphed this with m=a really small (positive) number and found that it converges almost exactly to ln(x)
anyway, i just thought it was pretty cool how the power property always holds so i figured id share it. most of the sites i see just say its ln(x), period.
is always true.
taking the integrand as x-1
at first we have
but the limit is 2 sided(as m approaches the positive side of zero i get a positive value, while as m approaches the negative side of zero, i get a negative value.)
So i decided to find the average of both sides of the limit:
i graphed this with m=a really small (positive) number and found that it converges almost exactly to ln(x)
anyway, i just thought it was pretty cool how the power property always holds so i figured id share it. most of the sites i see just say its ln(x), period.
Last edited:




