nycmathguy
- Homework Statement
- Write a linear equation that gives the dollar value V of the product in terms of the year t.
- Relevant Equations
- y = mx + b
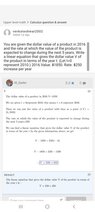
You are given the dollar value of a product in 2016 and the rate at which the value of the product is expected to change during
the next 5 years. Use this information to write a linear equation that gives the dollar value V of the product in terms of the year t. (Let t = 16 represent 2016.)1. 2016 Value = $3000
Rate = $150 decrease per year
Solution:
V(t) = dollar value V of the product in terms of the year t.
$150 decrease per year = -150t
So far, I have V(t) = -150t.
Stuck here...
2. 2016 Value = $200
Rate = $6.50 increase per year
V(t) = dollar value V of the product in terms of the year t.
$6.50 increase per year means 6.50t
So far, I have V(t) = 6.50t.
Stuck here...
the next 5 years. Use this information to write a linear equation that gives the dollar value V of the product in terms of the year t. (Let t = 16 represent 2016.)1. 2016 Value = $3000
Rate = $150 decrease per year
Solution:
V(t) = dollar value V of the product in terms of the year t.
$150 decrease per year = -150t
So far, I have V(t) = -150t.
Stuck here...
2. 2016 Value = $200
Rate = $6.50 increase per year
V(t) = dollar value V of the product in terms of the year t.
$6.50 increase per year means 6.50t
So far, I have V(t) = 6.50t.
Stuck here...