What is the maximum area of a rectangle inscribed in a circle?

[The_Prime_Number]

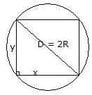
The problem asks me to show that the maximum possible area for a rectangle
inscribed in a circle of radius R is 2R^2. It also gives a hint saying that I should first maximize the square of the area.

I set the problem up as xy = 2R^2. I decided to work on the left side and wrote it as x(2R). But, I don't know where to go from here. Thanks.

 

[Pyrrhus]

You need to start up with a relationship such like

x^2 + y^2 = (2R)^2

and like you noted

xy = Area

The use the concept of derivate to maximize for the area.

The standard procedure is to find a or more relations F(x,y) and then turn it into a function F(x), so you can use the concept of derivative.

 

[Vivek Sharma]

If You know some trigonometry i.e. 2 sinA cosA = sin2A.

Solution : Since the rectangle is inscribed in a circle hence, its diagonal,D, is equal to the diamter,2R, of the given circle. i.e. D = 2R.
Now consider the triangle formed by the diagonal and two adjacent sides of our rectangle. It is a right triangle. Hence its sides can be expressed as D(diagonal the hypotenuse) , D*sinA and D*cosA(the perpendicular and base ).
Note : they also satisfy the pythagoras theorem D^2 = (D*sinA)^2 + (D*cosA)^2.

hence area of rectangle = product of adjacent sides
= DsinA * DcosA
= D*D* (sinA*cosA)
= 2R*2R*(sinA cosA)
= 2(R^2)*(sin 2A )
now R is fixed for a given circle. and A may vary.
for maximum area, sin2A must be maximum. which is 1
hence maximum area is 2R^2.
Note : sin 2A is 1 only if A =45 degree
which means that sinA = cos A
hence adjacent sides of the rectangle D*sinA and D*cosA become equal.
This justifies that in order to maximise its area the rectangle must be made into a square.

 

[dextercioby]

I like the geometrical solution proposed in post #3,but,to carry on with the idea in post #2,i'd say the simplest & most elegant way is to use a Lagrange multiplier.

Daniel.

 

[mathwonk]

this is a trivial calculus problem, to maximize A= xy when x^2 + y^2 = 4R^2, as x runs from 0 to 2R.

If we take the hint and maximize A^2 = x^2 y^2 = x^2 [ 4R^2 - x^2]

= 4R^2 x^2 - x^4, the derivative is 8R^2 x - 4x^3, which is zero when x = 0 and x = sqrt(2)R.

the max is obviously not at x= 0 hence occurs at x = sqrt(2)R (since on a closed interval there must be a max and it must occur at a critical point.

 

[mathwonk]

the solution is also obvious geometrically as follows: picture the level curves of the function xy, i.e. the hyperbolas xy = c. if you know what these look like, it is obvious that they are symmetrical about the line x=y, and the point on each hyperbola which is nearest the origin lies on this line.

Hence if we assume also that (x,y) lies on a circle of given radius, then the hyperbola xy = c which meets the circle and has largest value of c, is the one tangent to the circle at the point (sqrtc,sqrtc).