What is the Minimum Velocity to Throw a Ball Over a Pitched Roof?

[theultimate6]

Homework Statement

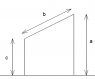
What is the minimum velocity required to throw a ball over a house with a pitched roof ( See attachment for the figure) You can choose the throwing point as needed

The Attempt at a Solution

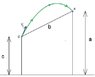
(Choose throwing point standing near $$a$$ and facing left) Assuming b is the hypotenuse of a right triangle and a-c is one side we can find the other side (let's call it d) using the Pythagorean theorem $$b^2 -(a-c)^2 = d$$

$$= -a^2 +2ac +b^2 -c^2$$

a is the maximum height of the projectile

$$a=(v^2sin^2θ)/2g$$

assuming d is the range

$$d=v^2sin(2θ)/g$$

I tried creating an equation for$$v$$from these equations , but it turned out to be too massive to even make a sensible answer.


Please help.

 

[haruspex]

$$b^2 -(a-c)^2 = d$$

d²

a is the maximum height of the projectile

Not necessarily. You should only assume the trajectory passes through the top of each vertical (a and c).

assuming d is the range

Different d?
Let x be the distance of the launch point from the first vertical, v the launch velocity and θ the angle. Write down the equations of motion and find what the requirement of just clearing the two verticals tells you. Then use calculus to find the least v. If you're familiar with them, could be a job for Lagrange multipliers.

 

[gneill]

If the ball just clears "a" and lands on the roof, but subsequently rolls down the pitch and off at side c, does that count as throwing the ball over the house? Or must it clear the house entirely?

 

[TSny]

You might first consider a related problem. What is the minimum speed V_C require to toss a ball from point C to point A in the attached figure? (Or, if you prefer, what's the minimum speed V_A required to toss a ball from point A to point C?)

If you could solve that, I think you could use the result to find the answer to the original question. I don't believe you need to worry about what point on the ground the ball should be thrown from.

I'm getting a pretty amazing answer for the original question. But, I've been wrong many times.

 

[ehild]

If the ball just clears "a" and lands on the roof, but subsequently rolls down the pitch and off at side c, does that count as throwing the ball over the house? Or must it clear the house entirely?

I think the ball can roll on the roof, and that means the smallest kinetic energy required, so the smallest initial speed.

ehild

 

[haruspex]

If the ball just clears "a" and lands on the roof, but subsequently rolls down the pitch and off at side c, does that count as throwing the ball over the house? Or must it clear the house entirely?

If you allow it to roll down, and you throw from the peak side of the roof, the answer will clearly be to stand very close and throw almost straight up at speed just over √(2ga).

 

[theultimate6]

Sorry for the late reply, I contacted my professor and he said no rolling is allowed, the ball should aim not to touch the roof at all if only extremely lightly.

d²

Not necessarily. You should only assume the trajectory passes through the top of each vertical (a and c).

Different d?
Let x be the distance of the launch point from the first vertical, v the launch velocity and θ the angle. Write down the equations of motion and find what the requirement of just clearing the two verticals tells you. Then use calculus to find the least v. If you're familiar with them, could be a job for Lagrange multipliers.

[tex]R=v_ocosθ/g∗(v_osinθ+√(v_o^2sin^2θ)) [tex]

$$-a^2 +2ac +b^2 -c^2 +x = v_ocosθ/g∗(v_osinθ+√(v_o^2sin^2θ))$$

Actually i have no idea about calculus, :)

 

[theultimate6]

If you could solve that, I think you could use the result to find the answer to the original question. I don't believe you need to worry about what point on the ground the ball should be thrown from.

I'm getting a pretty amazing answer for the original question. But, I've been wrong many times.

You're right our professor just gave us that hint.

PS: I'm really lost in this problem, i posted a solution but nobody has replied yet, I'm thinking about solving for θ from the maximum height equation then substituting it in the equation i posted, then use calculus (which i have no idea about).

 

[ehild]

Sorry for the late reply, I contacted my professor and he said no rolling is allowed, the ball should aim not to touch the roof at all if only extremely lightly.



$$R=v_ocosθ/g∗(v_osinθ+√(v_o^2sin^2θ))$$

$$-a^2 +2ac +b^2 -c^2 +x = v_ocosθ/g∗(v_osinθ+√(v_o^2sin^2θ))$$

Actually i have no idea about calculus, :)

I do not understand what you wrote. What are R and x?

ehild

 

[TSny]

I'm thinking about solving for θ from the maximum height equation then substituting it in the equation i posted, then use calculus (which i have no idea about).

For a projectile, fixing the speed at one height will automatically determine the speed at every other height. So, suppose you find the minimum speed the projectile can have at point C, say, such that it barely makes it to point A. That speed at C will fix the speed that the projectile needs to be launched from the ground.

To find the speed at C, you don't need to find the maximum height. Imagine launching the projectile at C at some angle θ_C. Can you find an expression for the launch speed V_C as a function of θ_C that will just get the projectile to A. Then, as you said, you can use calculus to minimize that function to determine the minimum speed at C that will get the ball to A. Then you can find the launch speed from the ground.

 

[theultimate6]

I do not understand what you wrote. What are R and x?

ehild

R is the range and x is the distance of the launch point from the first vertical

For a projectile, fixing the speed at one height will automatically determine the speed at every other height. So, suppose you find the minimum speed the projectile can have at point C, say, such that it barely makes it to point A. That speed at C will fix the speed that the projectile needs to be launched from the ground.

To find the speed at C, you don't need to find the maximum height. Imagine launching the projectile at C at some angle θ_C. Can you find an expression for the launch speed V_C as a function of θ_C that will just get the projectile to A. Then, as you said, you can use calculus to minimize that function to determine the minimum speed at C that will get the ball to A. Then you can find the launch speed from the ground.

I'm not sure if this is correct because time is against me but i'll try.

let y=c

$$c=V_csinθt-0.5gt^2 ?$$

OR

Edit:

$$Sinθ_c = c/v_c$$

$$v_c= c/sinθ_c$$

 

[theultimate6]

Can anybody please type anything my deadline is very near

 

[theultimate6]

$$sinθ=c/v_c$$
$$v_c = c/sinθ$$

$$c=c*sinθ/sinθ*t -0.5gt^2$$

?

 

[theultimate6]

my deadline is in 26 minutes

min = (c^2 csc^2 (theta) sin^2 (alpha))/2g

Please Help

 

[ehild]

From C to A, the projectile moves along the curve
y=c+xtan(θ)-(g/2) x²/(V_ccos(θ))².
It has to touch the pitch at hight y=a and x=d. You can find V_c² in terms of theta, and find its minimum.

ehild

 

[TSny]

my deadline is in 26 minutes

min = (c^2 csc^2 (theta) sin^2 (alpha))/2g

Please Help

Sorry, theultimate6.

You mentioned not being familiar with using calculus. I was trying to see if there is a way to solve it without calculus. I don't see another way.

 

[haruspex]

You mentioned not being familiar with using calculus. I was trying to see if there is a way to solve it without calculus. I don't see another way.

When optimising quadratics, there's often a non-calculus way that consists of expressing the part that becomes zero as a square.
As you said earlier, it reduces to lobbing the ball from one end of the roof just fast enough to clear the far end. Writing down the two equations of motion for a ball thrown at angle theta, speed v, then eliminating time, we get the parabola:
y =x tan(θ) - g x² sec²(θ)/2v²
To clear the far end:
a-c = d tan(θ) - g d² sec²(θ)/2v²
where d is the horizontal distance to the far end. Whence
2v²/(gd) = sec²(θ)/(tan(θ) - (a-c)/d)
= (1+t²)/(t - h), where t = tan(θ), h = (a-c)/d
By Pythagoras, 1+h² = (b/d)²
Whence 1+t² = 2(h + b/d)(t-h)+(t-h-b/d)²
So 2v²/(gd) = 2(h + b/d)+(t-h-b/d)²/(t-h)
This is minimised wrt t when t-h-b/d = 0, leaving v²/(gd) = h + b/d
v² = g(a - c + b)
To get the velocity needed from ground level, just need to add the energy to get it up to height c.

 

[TSny]

When optimising quadratics, there's often a non-calculus way that consists of expressing the part that becomes zero as a square.
As you said earlier, it reduces to lobbing the ball from one end of the roof just fast enough to clear the far end. Writing down the two equations of motion for a ball thrown at angle theta, speed v, then eliminating time, we get the parabola:
y =x tan(θ) - g x² sec²(θ)/2v²
To clear the far end:
a-c = d tan(θ) - g d² sec²(θ)/2v²
where d is the horizontal distance to the far end. Whence
2v²/(gd) = sec²(θ)/(tan(θ) - (a-c)/d)
= (1+t²)/(t - h), where t = tan(θ), h = (a-c)/d
By Pythagoras, 1+h² = (b/d)²

So far, similar to what I did.

Whence 1+t² = 2(h + b/d)(t-h)+(t-h-b/d)²

Did not see that trick at all!

So 2v²/(gd) = 2(h + b/d)+(t-h-b/d)²/(t-h)
This is minimised wrt t when t-h-b/d = 0, leaving v²/(gd) = h + b/d
v² = g(a - c + b)
To get the velocity needed from ground level, just need to add the energy to get it up to height c.

Yes, that gets it without calculus. Nice. Did you work out the final answer? I was a little amazed at the form it takes.

 

[haruspex]

Did not see that trick at all!

Well, of course I solved it with calculus first, then figured that if I subtracted the answer from the non-optimised expression I should get a squared term that vanished at optimum. Wouldn't have found it otherwise.

Did you work out the final answer? I was a little amazed at the form it takes.

Yes, it's astonishing. Makes one think there's a much easier way.