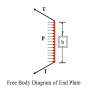
- #36
- 23,583
- 5,819
I really can't answer these questions, because they are outside my math knowledge base. If you like, I can ask a Mentor specializing in math to help.skrat said:Is it also possible to look at the whole problem as an isoperimetric problem from the maths? Concretely "Dido's problem" leads to a circular shape - which is also the case here.
That is if I assume that the area under the membrane will be maximized. I think this is a good argument too, but I don't have a good physical argument why the area would be maximized. Do you?
Is it due to the pressure difference? The air wants to expand as much as possible, therefore increase the area? :/
Chet