What Is the Oscillation Period of a Pendulum Suspended by an Inclined Wall?

[squareroot]

Homework Statement

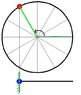
A pendulum with the length L is suspended by a inclined wall at an β angle.The pendulum is deviated with an angle of 2*β and then set free.Determine the oscillation period(T) of the pendulum considering that all the clashes are perfectly elastic.

Homework Equations

Here are some relevant equations
T=2$\pi$*$\sqrt{\frac{L}{g}}$
T=2$\pi$*$\sqrt{\frac{m}{k}}$
and all the quations from mechanical oscillations

The Attempt at a Solution

Nothing relevant yet.

 

[squareroot]

anyone?

 

[squareroot]

Amost forgot , a picture for you.

 

[bayan]

since it is 11th grade problem I assume the angle is small such that sin θ=θ. With this being the case T mainly depends on L.

You are told that the collision with the wall is elastic which means it rebounds at same speed, this is same as if the wall was not there.

The only effect the wall has is it changes the oscillation period to 3/4 ( just pic one oscillation as 8 parts, you are missing 2 from wall to left side max height)

Find the oscillation period if the wall was not there and take the effect of wall into account.

Hope this helps

 

[squareroot]

Thank you.I've initially thought of what you said, it's observable , but my problem stands in proving that mathematically.

 

[TSny]

Hi, squareroot. Have you studied the mathematical expression for the position of the pendulum as a function of time? That would be the most accurate way to determine the time to go from release to hitting the wall. Taking 3/8 of the period of the unblocked pendulum will not be very accurate. That's because it does not take the pendulum the same amount of time to swing through each eighth of a cycle.

If you've studied the relationship between simple harmonic motion and uniform circular motion, then you might see if you can use that relationship to get the answer.

 

[squareroot]

I've been fussing with this one for 1 hour , still coudn't figure it out and i need it for tomorrow.Ahhh!

 

[TSny]

If you go to this link, you will see how simple harmonic motion is a "projection" of uniform circular motion.
http://www.physics.uoguelph.ca/tutorials/shm/phase0.html

Think of the blue ball as moving back and forth in simple harmonic motion similar to a pendulum swinging back and forth. The red ball travels at a constant speed around the circle. The interesting thing here is that the blue ball, which does not move with constant speed, is always directly under the red ball.

Imagine that when the blue ball is at the far right, that corresponds to the release of the pendulum. Where would you locate a wall on the line of motion of the blue ball so that it would correspond to the wall in your problem?

How far around the circle has the red ball traveled when it is over the wall?

 

[squareroot]

Yes , i understood that , as I said it's intuitive, but i can't prove it mathematically.It is there where my problems are.

 

[TSny]

What answer did you get using your intuition?

When you say you are trying to prove it mathematically, you will need to know more than just the formula for the period of a pendulum. What other mathematical equations have you studied that relate to pendulum motion?

 

[squareroot]

well , we can find out how much the red ball has traveled around the portion of the circle "behind" the wall with the formula i attached below , where r is the length of the string(L) and n I THINK(but i m not sure) is alpha.but from here on , I'm lost.I also have the answer sheet and it says the answer is $\frac{4}{3}$*$\pi$*$\sqrt[]{\frac{l}{g}}$

Here are some more formulas that i know related to oscillation

v=ωA*cos(ωt+θ)
y=Asin(ωt+θ)
a=-ω$^{2}$Asin(ωt+θ)

where v= velocity , y=elongation , A-amplitude(maximum elongation) and ω-angular speed , θ-initial phase

T=2$\pi$$\sqrt{\frac{m}{k}}$
ω=2$\pi$*$\nu$ , $\nu$=$\frac{1}{T}$
k=m*ω*ω
T=2$\pi$$\sqrt{\frac{l}{g}}$
Kinetic energy=m*v*v/2 , potential energy=k*y*y/2

 

[TSny]

y=Asin(ωt+θ)

OK. So, here's an equation that will tell you the position, y, at any time, t. For a pendulum you may think of y as replaced by the angle, $\phi$, that the string makes with the vertical. So,

$\phi$ = Asin(ωt+θ).

Think about what you would use for the amplitude A in this equation.

Think about the value of $\phi$ at t = 0. This will help you determine the phase angle θ.

Finally, think about the value of $\phi$ at the time the pendulum hits the wall. That will help you find the time at which the pendulum hits the wall.

 

[TSny]

If you want to use the graphical approach, study the picture attached. What fraction of a circle has the red ball traveled by the time the blue ball hits the green wall?

 

[squareroot]

So, from the 2 relation that you've given me, for i finding A i thought of this , sin(2alpha)=A/l , but it is specified that 2alpha<6 degrees which means that 2alpha=A/l and A is 2alpha*l so by replaing this in the equation 2alpha=Asin(ωt+θ) at t=0 that means that

2alpha=2alpha*l*sinθ so θ=1/l (thinking that sinθ≈θ)
moving to the second relation where θ=alpha(when tha ball hits the wall)

alpha=Asin(ωt+θ) so that alpha=2alpha*l* sin(ωt+1/l) so that t=$\frac{-1}{2lω}$

But how do i get from this to the period?

 

[TSny]

In the equation $\phi$ = A sin(ωt+θ), A is the amplitude or the maximum amount that $\phi$ would have during the motion. So, how does A relate to the angle $\alpha$ shown in the figure of the pendulum that you posted?

 

[squareroot]

Well the maximum angle is 2α in the picture of the problem , so at t=0 , when you release the ball the angle is 2α, i figured that ϕ is your notation for α.And when the ball hits the wall the angle is α.

A is the maximum distance from the point of equilibrum so the max distance is reached when the object is at the angle of 2α and we (in class)take the distance from the point of equillibrum to the ball as a straight line(for simplicity) so sin2α=A/l, where l is the hypotenuse and A is the adjacent.

 

[TSny]

Well the maximum angle is 2α in the picture of the problem , so at t=0 , when you release the ball the angle is 2α, i figured that ϕ is your notation for α.And when the ball hits the wall the angle is α.

Right, the amplitude A is 2α. Also, $\phi$ = 2α at t = 0. Try to use this information to figure out $\theta$.

[Got a doctor's appointment, so I need to skip out for now.]

 

[squareroot]

Oh , man , i need it until tomorrow and its 9.30 PM in my country.Boy am I dead...

 

[squareroot]

I managed to get another day , i have until tomorrow.I l start working as soon as i get home.

 

[TSny]

As the pendulum swings, think of y = A sin (ωt+θ) as describing the horizontal motion of the pendulum. So, y represents the horizontal position relative to the equilibrium position.

At the moment the pendulum is released, what is the value of y? You won’t be able to give a numerical value, but how would you express y in terms of A at the moment of release?

What would the value of t be at the moment of release?

Substitute these values of y and t into the equation and see what you discover.