What is the purpose of the vector α in the rotational work integral?

[pherytic]

This brief worked example from a textbook section on the method of images is confusing me.

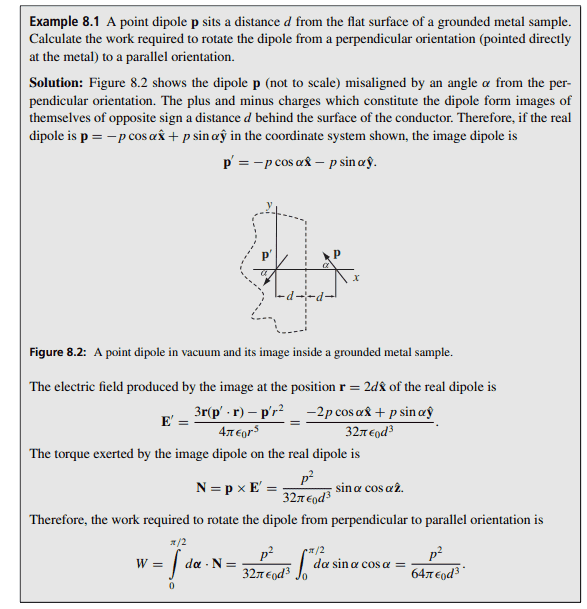
Specifically I am confused about the vector α in the integral on the last line.

When α (or θ) is an angle, I've only ever seen the vector quantity α (or θ) as a polar vector in the plane. But here, that can't be right because the dot product of the unit vectors α ⋅ z = 1, so they are clearly parallel.

What is the correct understanding of the vector α?

It seems like maybe it is supposed to be an axial pseudovector whose magnitude gives a sort of angular position in the plane normal to the vector. But I don't think I have ever seen a vector used this way and I am not sure it makes sense.

 

[vanhees71]

It's not a vector but the angle of your real dipole with the negative direction of the $x$-axis, as written in the solution. The virtual "image dipole" is the reflection of the dipole on the $yz$ plane.

 

[Ibix]

It's not a vector but the angle of your real dipole

I agree about $\alpha$, but in the last equation there is a $d\mathbf{\alpha}$ that appears in $d\mathbf{\alpha}\cdot\mathbf{N}$, which certainly looks like a vector.

I must say I don't recall seeing an angle represented that way, but since angular velocity is a pseudovector presumably $\mathbf{\omega}\Delta t$ is one too.

Note: the alpha in $d\mathbf{\alpha}$ is supposed to be a mathbf alpha, but at least for me it doesn't render noticeably different from a regular alpha. Ditto the omega.

 

[vanhees71]

Sorry, I've not read the solution carefully enough. It's of course the axial vector $\alpha \hat{z}$, describing the rotation of the dipole around the $z$-axis.