What is the right formula to use in this context? (momentum conservation)

[El foolish Phenomeno]

Homework Statement

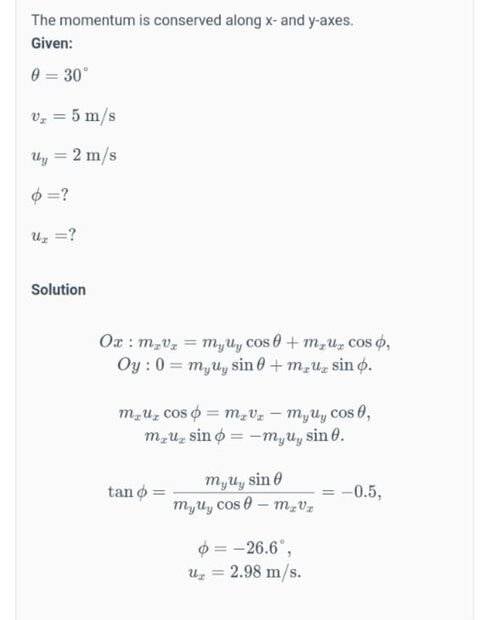
A pool ball X of mass 0.3 kg moving with velocity 5 m/ s hit a stationary ball Y of mass 0.4 kg . Y moves off with a velocity of 2 m/ s at 30 ° to the initial direction of X . Find the X and it's direction after hitting it.

Relevant Equations

momentum formulas

First i think the correct solution to the problem is

But my friends argue that it is not what i did , i am confused we didn't see the whole chapter on momentum in class, (Youtube thank you)

here is what my friends say :

(0.3×5) + 0 = (V×0.3)+(0.4×2)
and they get they a final speed of 2.33 m/s , with negative direction.

 

[BvU]

Hi,

I think you are correct. Can you guess what kind of collision your friends' equation describes ?

$\$

 

[BvU]

@kuruman : ball Y moves off with x component velocity in the positive direction ...

$\$

 

[kuruman]

@kuruman : ball Y moves off with x component velocity in the positive direction ...

$\$

Oops! I misread the problem. I deleted my post to avoid confusion. Thanks for the heads up.

 

[El foolish Phenomeno]

Sin

Hi,

I think you are correct. Can you guess what kind of collision your friends' equation describes ?

$\$

since there is no mention of conservation of energy in that equation , i'd say inelastic collision.

 

[BvU]

Consider that it is momentum conservation in one single direction: a central collision. And elastic (check !)

$\$

 

[El foolish Phenomeno]

Consider that it is momentum conservation in one single direction: a central collision. And elastic (check !)

$\$

Lol. In an elastic collision , both Energy and Momentum are conserved. I was distracted .

Consider that it is momentum conservation in one single direction: a central collision. And elastic (check !)

$\$

 

[haruspex]

Lol. In an elastic collision , both Energy and Momentum are conserved. I was distracted .

You may be missing the point of @BvU's question.
You asked how come your friends did not need energy conservation. The reason is that they treated it as a head-on collision. On that basis they did not need to consider energy; there was enough information to find X's final velocity (and to calculate the energy change).
As a 2D collision, we have one more unknown, so a second equation is needed.

 

[BvU]

I'm coming back to post #1, checking the math and such

here is what my friends say :

(0.3×5) + 0 = (V×0.3)+(0.4×2)
and they get they a final speed of 2.33 m/s , with negative direction.

Doing the math gets me 2.33 m/s in the positive direction....

the correct solution to the problem ...

I get -26.36$^\circ$ and 3.00 m/s. Nitpicking ?

And elastic (check !)

Check: $T_{in} = {1\over 2}m_x v_x^2$ = 3.75 J and
$T_{out} = {1\over 2}m_x \; 2.33^2 + {1\over 2}m_y u_y^2$ = 1.62 J, so not elastic (my bad suggesting that in post #6 ).

and for the correct result $T_{out} =$ 2.15 J, not elastic either.

If you want to get some practice, determine $u_y$ for the case of an elastic collision with $\theta = 30^\circ$
$\$

 

[haruspex]

I get -26.36$^\circ$

Yes. The mistake in the given answer was in rounding 0.49555.. to 0.5 in the preceding line.

 

[BvU]

So it's nitpicking allright. Given data are only one decimal ...

However, my rule is not to round off intermediate results unnecessarily.

$\$

 

[haruspex]

So it's nitpicking allright. Given data are only one decimal ...

However, my rule is not to round off intermediate results unnecessarily.

$\$

Not nitpicking at all. The answer should have been given as either 26° or 26.4°, not 26.6°.