- #1
hb20007
- 18
- 0
"sinh" in Snell's Law?
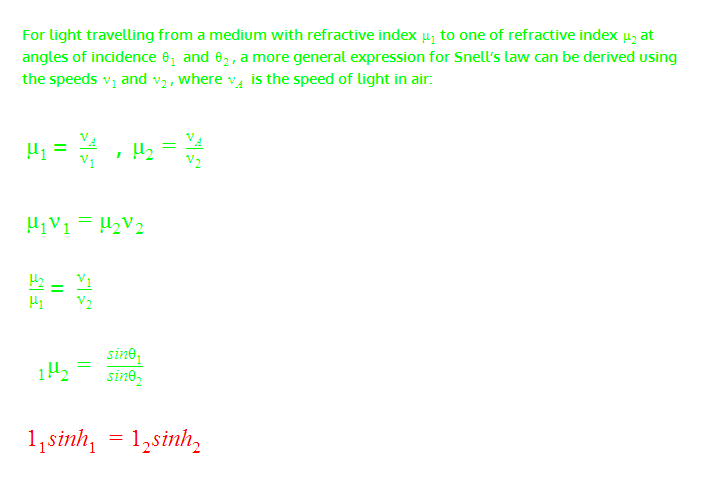
I'm trying to understand some equations in my AS level textbook about Snell's Law
The equations are in the image below
I tried reading up about sinh but I don't fully understand what it is. Also I'm not sure what the term beside sinh is. It can't be a "1" and to seems like a small letter L which comes out of nowhere?
Homework Statement
I'm trying to understand some equations in my AS level textbook about Snell's Law
Homework Equations
The equations are in the image below
The Attempt at a Solution
I tried reading up about sinh but I don't fully understand what it is. Also I'm not sure what the term beside sinh is. It can't be a "1" and to seems like a small letter L which comes out of nowhere?
 .
.