- #1
cersepn
- 1
- 0
After much trouble with using the in-built engine to display all the mathematical formula, i thought scanning the question was the only way i could get my point across
For (1) is C also defined by the parametric representation of [tex]\widetilde{}C[/tex] which is why the direction of C is negative?
Then for (3), i don't get where the '2t dt' term comes from
Help would be much appreciated.. I've been searching online for some good green theorem lessons but I'm still kinda confused after reading them all.. thanks
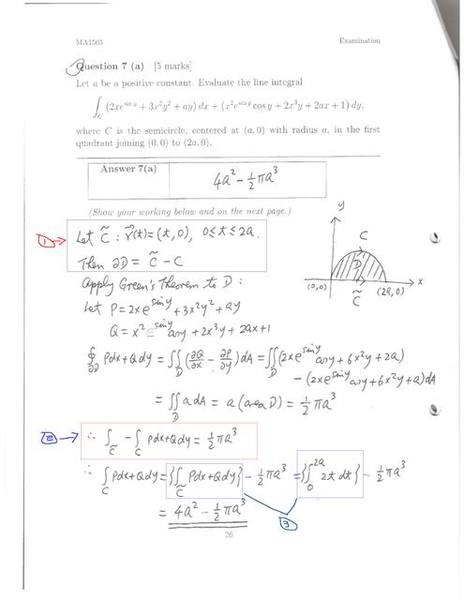
For (1) is C also defined by the parametric representation of [tex]\widetilde{}C[/tex] which is why the direction of C is negative?
Then for (3), i don't get where the '2t dt' term comes from
Help would be much appreciated.. I've been searching online for some good green theorem lessons but I'm still kinda confused after reading them all.. thanks
Last edited: