What kx/ky Values Are Used for Graphene Band Structure Plots?

[Boccard]

TL;DR

Band structure of graphene and its energy dispersion.

Hello,

https://www.researchgate.net/figure/Top-Graphene-nanoribbons-with-zigzag-left-and-armchair-right-edges-with-disordered_fig1_258105786

With what kx/ky values are these band structures plotted?

The energy dispersion of graphene is given by this

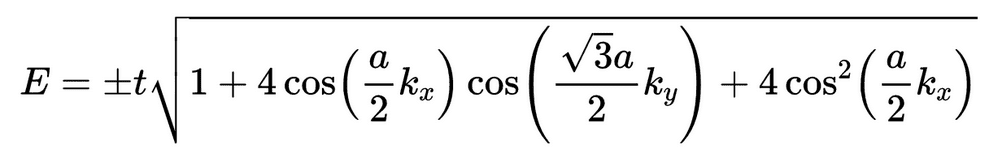
, yet I am confused to what kx/ky value choices I make to plot the graphs depicted in the link.

 

[TeethWhitener]

The band structure of graphene is not the same as the band structure of graphene nanoribbons. Graphene is a two-dimensional system, whereas GNRs are quasi-one dimensional, so $k_x$ and $k_y$ don’t make sense for GNRs.

Also the equation you provide is the tight binding energy of the valence and conduction bands of graphene, whereas the link gives a plot of the complete band structure for nanoribbons.

 

[Boccard]

This does not make sense to me.

Using that equation I can reproduce the left plot if I plot kx for some values of ky (with the exception of the lowest energy mode; where does this come from?).

 

[TeethWhitener]

I’m not sure what you mean. There is no two dimensional band structure in a one dimensional system.

 

[Boccard]

The band structure plotted on the left graph is simply the 2D energy dispersion function of the graphene, but for specific k_y values that depend on the width of the system. The discretized bands of the system are different k_y values.

My question is, what are these values``?

 

[TeethWhitener]

The band structure plotted on the left graph is simply the 2D energy dispersion function of the graphene, but for specific k_y values that depend on the width of the system. The discretized bands of the system are different k_y values.

My question is, what are these values``?

Maybe we aren’t talking about the same thing. In the link you provided, the image at the left is the band structure for a zigzag graphene nanoribbon. This is a 1D structure. Hence the wave vector being referred to simply as “k.” A zigzag graphene nanoribbon is not graphene. Draw the unit cell and this will be obvious.

 

[Boccard]

Yes, I was indeed confusing the structures. Thank you for the help.