- #1
mathmari
Gold Member
MHB
- 5,049
- 7
Hey! :giggle:
An aeroplane flies over a tower of height $h> 0$ at height $H> h$. At what distance $x$ is the angle $\alpha$ at which the tower is seen from the aeroplane, maximum?
(You can use elementary geometry and that $\arctan'(x)=\frac{1}{1+x^2}$.)
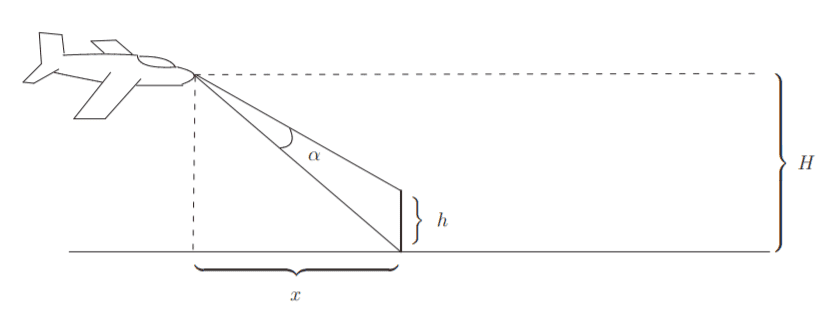 From Pythagorean Theorem for the larger triangle we have that $H^2+x^2=:y^2$.
From Pythagorean Theorem for the larger triangle we have that $H^2+x^2=:y^2$.
Do we apply for the smaller triangle law of cosine? But the upper side of that triangle is not known,and not related to the other triangle.
From the hint... Do we have to use somehow $\tan(\alpha)$ ?
:unsure:
An aeroplane flies over a tower of height $h> 0$ at height $H> h$. At what distance $x$ is the angle $\alpha$ at which the tower is seen from the aeroplane, maximum?
(You can use elementary geometry and that $\arctan'(x)=\frac{1}{1+x^2}$.)
Do we apply for the smaller triangle law of cosine? But the upper side of that triangle is not known,and not related to the other triangle.
From the hint... Do we have to use somehow $\tan(\alpha)$ ?
:unsure: