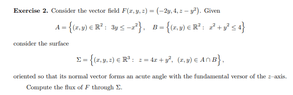When to use the Jacobian in spherical coordinates?

[Amaelle]

Homework Statement:

look at the image

Relevant Equations:

jacobian is r^2 sinv

Greetings!

here is the solution which I undertand very well:

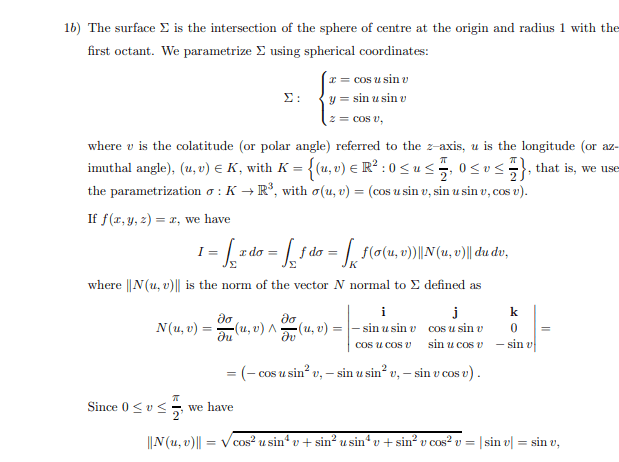
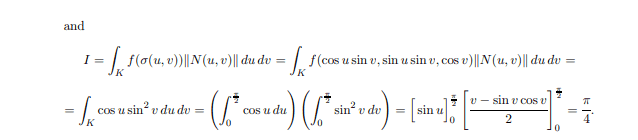
my question is:
if we go the spherical coordinates shouldn't we use the jacobian r^2*sinv?

thank you!

 

[ergospherical]

It's sort of already baked in. The surface element comes from a little parallelogram with sides $d\boldsymbol{l}_u = \frac{\partial \boldsymbol{\sigma}}{\partial u} du$ and $d\boldsymbol{l}_v = \frac{\partial \boldsymbol{\sigma}}{\partial v} dv$ and therefore area
\begin{align*}
dS = |d\boldsymbol{l}_u \times d\boldsymbol{l}_v| = \left| \frac{\partial \boldsymbol{\sigma}}{\partial u} \times \frac{\partial \boldsymbol{\sigma}}{\partial v} \right| du dv
\end{align*}The part $\frac{\partial \boldsymbol{\sigma}}{\partial u} \times \frac{\partial \boldsymbol{\sigma}}{\partial v}$ is what your solutions denoted by the vector $\mathbf{N}$.

 

[Amaelle]

thank you so much!