paulimerci
- 287
- 47
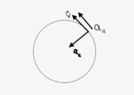
I understand that the velocity vector and acceleration vector are perpendicular to each other at every location on the circle in uniform circular motion at a constant speed because the acceleration vector points in the direction of the circle's center and the velocity is always tangent to the circle. Where should I draw "another acceleration vector" that you have pointed out in the above question?Lnewqban said:What happens during circular movement?
Could you have another acceleration vector that could be combined with the vector of centripetal acceleration in such a way that the resultant acceleration vector forms a constant angle with the velocity vector of the ball?