- #1
PeteWheatstraw
- 6
- 0
instead of typing it out, here is the problem
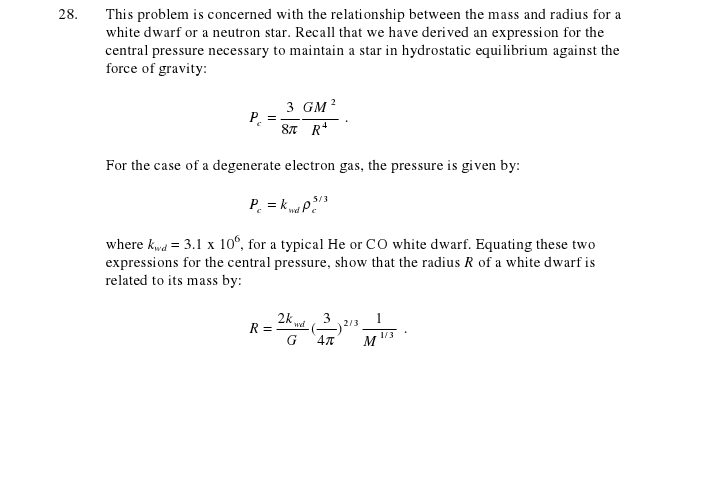
I know what to do, my math just isn't good enough to combine the equations properly. Been at it a few hours, brain hurts, please help!
I know what to do, my math just isn't good enough to combine the equations properly. Been at it a few hours, brain hurts, please help!