Why are the accelerations not equal?

[annamal]

TL;DR Summary

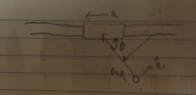
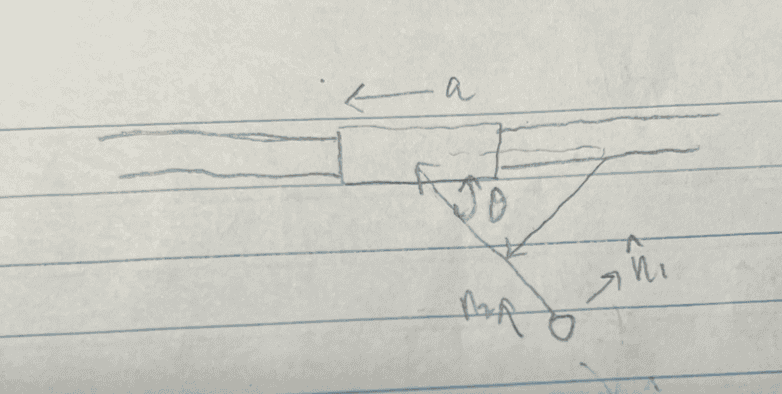
I have a slider moving with a pendulum. I take the derivative of the velocity vector. Then I get the acceleration but just using a for acceleration. How come the accelerations from equations (1) and (2) [both ways of getting the acceleration] are not equal?

$\vec n_2$ points to the diagonal top left.

The velocity v is a function of t. So for example $2t^2$ and a = dv/dt.
Putting the velocity vector into $\vec n_1$ and $\vec n_2$ terms.
$$\vec v = v sin\theta \vec n_1 + v cos\theta \vec n_2$$
$$\vec v = -v \vec i$$
$$\vec a = \frac{d\vec v}{dt} = -a \vec i = -asin\theta \vec n_1 + acos\theta \vec n_2\ (1)$$
$$\vec a = \frac{d(v sin\theta \vec n_1 + v cos\theta \vec n_2)}{dt} = (asin\theta - 2v cos\theta \dot{\theta})\vec n_1 + (-2vsin\theta \dot{\theta} + acos\theta)\vec n_2\ (2)$$
How come $\vec a \neq -asin\theta \vec n_1 + acos\theta \vec n_2\ (1) \neq (2)$?

 

[Ibix]

Are $\vec n_1$ and $\vec n_2$ constant, and it's only coincidence that they happen to point along your pendulum rod when you draw the diagram? If so, $\vec v=v\sin\theta\vec n_1+v\cos\theta\vec n_2$ is not generally true. If not, then where are the $\dot{\vec n}_i$ terms in your expression for $\vec a$?

 

[annamal]

Are $\vec n_1$ and $\vec n_2$ constant, and it's only coincidence that they happen to point along your pendulum rod when you draw the diagram? If so, $\vec v=v\sin\theta\vec n_1+v\cos\theta\vec n_2$ is not generally true. If not, then where are the $\dot{\vec n}_i$ terms in your expression for $\vec a$?

Yes $\vec n_2$ points along my pendulum rod as it moves and $\vec n_1$ points perpendicular to that constantly.

 

[Ibix]

Then they are functions of time also, and you need their derivatives also.

 

[annamal]

Then they are functions of time also, and you need their derivatives also.

I already included and simplified their derivatives in equation (2)

 

[Ibix]

I already included and simplified their derivatives in equation (2)

Ah, I see. My mistake - I missed the factors of 2 somehow. Just to note, for those of us reading on small screens it's much easier to follow maths if you break it across lines a bit using the eqnarray or eqnarray* environments in LaTeX.

Are you sure your signs are consistent? In the case $\theta=\pi/2$ your un-numbered expression for $\vec v$ becomes $\vec v=-v\vec i=v\vec n_1$, implying $\vec i$ and $\vec n_1$ are anti-parallel, but (1) becomes $\vec a=-a\vec i=-a\vec n_1$, implying they are parallel.

 

[annamal]

Ah, I see. My mistake - I missed the factors of 2 somehow. Just to note, for those of us reading on small screens it's much easier to follow maths if you break it across lines a bit using the eqnarray or eqnarray* environments in LaTeX.

Are you sure your signs are consistent? In the case $\theta=\pi/2$ your un-numbered expression for $\vec v$ becomes $\vec v=-v\vec i=v\vec n_1$, implying $\vec i$ and $\vec n_1$ are anti-parallel, but (1) becomes $\vec a=-a\vec i=-a\vec n_1$, implying they are parallel.

Above I forgot to add a negative to $\vec n_1$ so $\vec v = -v \vec i = -v sin\theta \vec n_1 + v cos\theta \vec n_2$ not just $v\vec n_1$. But the equations (1) and (2) should be correct.

 

[Ibix]

Not quite. Like this:$$\begin{eqnarray*}
\vec v &=& -v\vec i \\
&=&v\sin\theta\vec n_1 + v\cos\theta \vec n_2
\end{eqnarray*}$$Note the & symbols each side of the thing I want to align (= in this case) and the \\\\ marking the end of each line except the last.

 

[annamal]

Not quite. Like this:$$\begin{eqnarray*}
\vec v &=& -v\vec i \\
&=&v\sin\theta\vec n_1 + v\cos\theta \vec n_2
\end{eqnarray*}$$Note the & symbols each side of the thing I want to align (= in this case) and the \\ marking the end of each line except the last.

I forgot to add the negative sign to $vsin\theta$ but everything else is the same

 

[Ibix]

I forgot to add the negative sign to $vsin\theta$ but everything else is the same

Looks like you edited while I was replying. Ignore my last post, and I'll read your revised post.

 

[annamal]

Looks like you edited while I was replying. Ignore my last post, and I'll read your revised post.

$$\vec a = \frac{d(-v sin\theta \vec n_1 + v cos\theta \vec n_2)}{dt} = (-asin\theta - 2v cos\theta \dot{\theta})\vec n_1 + (-2vsin\theta \dot{\theta} + acos\theta)\vec n_2\ (2)$$
$$\vec a \neq -asin\theta \vec n_1 + acos\theta \vec n_2\ (1) \neq (2)$$

 

[Ibix]

I think your algebra's gone wrong somewhere. Defining $\vec i$ pointing to the left right and $\vec j$ pointing up the page, we have:$$\begin{eqnarray}
\vec n_1 &=&\sin\theta \vec i+\cos\theta \vec j\\
\vec n_2&=&-\cos\theta \vec i+\sin\theta\vec j\\
\dot{\vec n}_1&=&-\vec n_2\dot\theta\\
\dot{\vec n}_2&=&\vec n_1\dot\theta
\end{eqnarray}$$Then, taking your definition $\vec v = -v\vec i$ and noting that $\vec i=\vec n_1\sin\theta-\vec n_2\cos\theta$ we can write:$$\begin{eqnarray}
\vec v&=&-v\vec i\\
&=&-v\vec n_1\sin\theta+v\vec n_2\cos\theta\\
\frac{d}{dt}\vec v&=&\frac{d}{dt}\left(-v\vec n_1\sin\theta+v\vec n_2\cos\theta\right)\\
&=&-a\left(\vec n_1\sin\theta-\vec n_2\sin\theta\right)\nonumber\\
&&+v\left(-\dot{\vec n}_1\sin\theta+\dot{\vec n}_2\cos\theta\right)\nonumber\\
&&-v\left(\vec n_1\cos\theta\dot\theta+\vec n_2\sin\theta\dot\theta\right)
\end{eqnarray}$$Substituting in the definitions (3 and 4) of the two $\dot{\vec n}$s, the last two brackets cancel.

They must cancel, of course, because you are simply writing $\vec a=-\frac{dv}{dt}\vec i-v\frac{d\vec i}{dt}$ and expanding $\frac{d\vec i}{dt}$ in terms of your normal vectors.

 

[annamal]

I think your algebra's gone wrong somewhere. Defining $\vec i$ pointing to the left and $\vec j$ pointing up the page, we have:$$\begin{eqnarray}
\vec n_1 &=&\sin\theta \vec i+\cos\theta \vec j\\
\vec n_2&=&-\cos\theta \vec i+\sin\theta\vec j\\
\dot{\vec n}_1&=&-\vec n_2\dot\theta\\
\dot{\vec n}_2&=&\vec n_1\dot\theta
\end{eqnarray}$$Then, taking your definition $\vec v = -v\vec i$ and noting that $\vec i=\vec n_1\sin\theta-\vec n_2\cos\theta$ we can write:$$\begin{eqnarray}
\vec v&=&-v\vec i\\
&=&-v\vec n_1\sin\theta+v\vec n_2\cos\theta\\
\frac{d}{dt}\vec v&=&\frac{d}{dt}\left(-v\vec n_1\sin\theta+v\vec n_2\cos\theta\right)\\
&=&-a\left(\vec n_1\sin\theta-\vec n_2\sin\theta\right)\nonumber\\
&&+v\left(-\dot{\vec n}_1\sin\theta+\dot{\vec n}_2\cos\theta\right)\nonumber\\
&&-v\left(\vec n_1\cos\theta\dot\theta+\vec n_2\sin\theta\dot\theta\right)
\end{eqnarray}$$Substituting in the definitions (3 and 4) of the two $\dot{\vec n}$s, the last two brackets cancel.

They must cancel, of course, because you are simply writing $\vec a=-\frac{dv}{dt}\vec i-v\frac{d\vec i}{dt}$ and expanding $\frac{d\vec i}{dt}$ in terms of your normal vectors.

For this $\dot{\vec n_1}$ and $\dot{\vec n_2}$ I have them negated.
$\dot{\vec n_1} = \dot{\theta}\vec k \times \vec n_1 = \dot{\theta}\vec n_2$ where $\vec k$ is pointing out of the screen and $\vec n_1 \times \vec n_2 = \vec k$.
$\dot{\vec n_2} = \dot{\theta}\vec k \times \vec n_2 = -\dot{\theta}\vec n_1$

 

[Ibix]

I did it by direct calculation$$\begin{eqnarray*}
\vec n_1&=&\sin\theta\vec i+\cos\theta\vec j\\
\dot{\vec n}_1&=&\cos\theta\dot\theta\vec i-\sin\theta\dot\theta\vec j\\
&=&-\vec n_2\dot\theta
\end{eqnarray*}$$and similarly for the other one.

 

[annamal]

I think your algebra's gone wrong somewhere. Defining $\vec i$ pointing to the left and $\vec j$ pointing up the page, we have:$$\begin{eqnarray}
\vec n_1 &=&\sin\theta \vec i+\cos\theta \vec j\\
\vec n_2&=&-\cos\theta \vec i+\sin\theta\vec j\\
\dot{\vec n}_1&=&-\vec n_2\dot\theta\\
\dot{\vec n}_2&=&\vec n_1\dot\theta
\end{eqnarray}$$Then, taking your definition $\vec v = -v\vec i$ and noting that $\vec i=\vec n_1\sin\theta-\vec n_2\cos\theta$ we can write:$$\begin{eqnarray}
\vec v&=&-v\vec i\\
&=&-v\vec n_1\sin\theta+v\vec n_2\cos\theta\\
\frac{d}{dt}\vec v&=&\frac{d}{dt}\left(-v\vec n_1\sin\theta+v\vec n_2\cos\theta\right)\\
&=&-a\left(\vec n_1\sin\theta-\vec n_2\sin\theta\right)\nonumber\\
&&+v\left(-\dot{\vec n}_1\sin\theta+\dot{\vec n}_2\cos\theta\right)\nonumber\\
&&-v\left(\vec n_1\cos\theta\dot\theta+\vec n_2\sin\theta\dot\theta\right)
\end{eqnarray}$$Substituting in the definitions (3 and 4) of the two $\dot{\vec n}$s, the last two brackets cancel.

They must cancel, of course, because you are simply writing $\vec a=-\frac{dv}{dt}\vec i-v\frac{d\vec i}{dt}$ and expanding $\frac{d\vec i}{dt}$ in terms of your normal vectors.

I think you meant to say $\vec i$ has to be pointing to the right and $\vec j$ has to be pointing up.

That is interesting that the $\frac{dn_1}{dt}$ and $\frac{dn_2}{dt}$ ended up that way. In order for my way of calculating them to be true, we would have to be rotating clockwise so $-\dot{\theta}$ by default instead of just $\dot{\theta}$. Then $\frac{dn_1}{dt} = \vec \omega \times \vec r = -\dot{\theta}\vec n_3 \times \vec n_1 = -\dot{\theta}\vec n_2$. I don't know why we would have to use a negative theta though. Perhaps because if we move in the positive $\vec i$ direction we have clockwise rotation?

 

[Ibix]

I think you meant to say $\vec i$ has to be pointing to the right and $\vec j$ has to be pointing up.

I did. I've corrected my post - thank you.

It's quite likely that something you've done implies clockwise instead of anti clockwise, yes. I suspect the fundamental issue is all the different conventions you are using. Notably, the way you've defined $\theta$, an increasing value means clockwise rotation if your $\vec n$ vectors, and I rather suspect your cross products assume the opposite - I haven't checked, though.

Honestly, I'd just start again, defining $\theta$ so that it is zero when $\vec n_1=\vec i$ and increases under anticlockwise rotation and defining $\vec v=v\vec i$. Competing conventions are always a mess - you can make them work, but you have to be constantly on guard for clashes. Much simpler to use standard choices.

 

[annamal]

Notably, the way you've defined $\theta$, an increasing value means clockwise rotation if your $\vec n$ vectors, and I rather suspect your cross products assume the opposite - I haven't checked, though.

So you think the way I have defined $\theta$ is clockwise b/c we are measuring the angle from the horizontal to the pendulum string correct? How come we cannot measure the angle from the pendulum string to the horizontal and say that $\theta$ is defined counterclockwise (the way I have the angle arrow drawn in my first post)?

 

[erobz]

So you think the way I have defined $\theta$ is clockwise b/c we are measuring the angle from the horizontal to the pendulum string correct? How come we cannot measure the angle from the pendulum string to the horizontal and say that $\theta$ is defined counterclockwise (the way I have the angle arrow drawn in my first post)?

I think ( and I believe @Ibix is saying) you have to be careful about the negative $\dot \theta$. imagine the slider still; If the angle is decreasing the angular velocity is negative, but by your assumed convention the bobs velocity is positive. That doesn’t seem to be mathematically consistent.