- #1
JD_PM
- 1,131
- 158
I am studying the deuterium's nucleus.
As we know, there are just two eigenstates for a spin 1/2 particle: either spin up or spin down.
Thus, over the whole nucleus, you get 4 possible combinations:
1) Spin up-spin up
2) Spin up-spin down
3) Spin down-spin up
4) Spin down-spin down
If you add the spins up, the four cases, you get +1, 0, 0, -1 respectively.
S = 1 means there are 3 possible values for the total nuclear spin of the nucleus, while s = 0 means there is just 1 possible value.
You can watch the enlightening Dr. Physics video on nuclear spin for further details:
But I have been told there are just two possible cases instead: Spin up-spin up and Spin up-spin down.
I asked why and the following table was used to argue it:
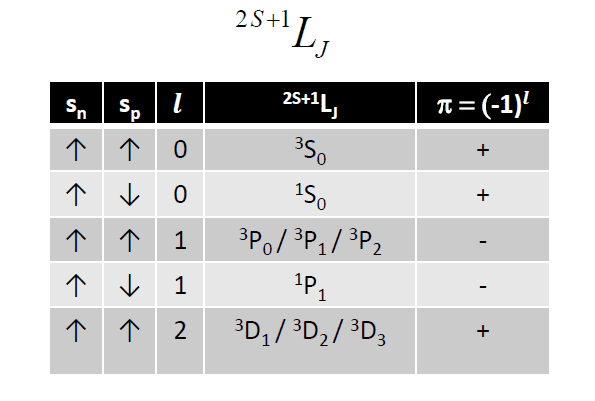
Note that here the cases are not as expected; the eigenstate of the neutron never is spin-down...
May you shed some light on why I am wrong assuming four possible eigenstates for the coupled proton-neutron case and on how to interpret the attached table?
Note: what I think it is going on here is that the general state of deuterium is just the normalized linear combination of both spin-up and spin-down, but I want to go further than that.
Thanks
As we know, there are just two eigenstates for a spin 1/2 particle: either spin up or spin down.
Thus, over the whole nucleus, you get 4 possible combinations:
1) Spin up-spin up
2) Spin up-spin down
3) Spin down-spin up
4) Spin down-spin down
If you add the spins up, the four cases, you get +1, 0, 0, -1 respectively.
S = 1 means there are 3 possible values for the total nuclear spin of the nucleus, while s = 0 means there is just 1 possible value.
You can watch the enlightening Dr. Physics video on nuclear spin for further details:
But I have been told there are just two possible cases instead: Spin up-spin up and Spin up-spin down.
I asked why and the following table was used to argue it:
Note that here the cases are not as expected; the eigenstate of the neutron never is spin-down...
May you shed some light on why I am wrong assuming four possible eigenstates for the coupled proton-neutron case and on how to interpret the attached table?
Note: what I think it is going on here is that the general state of deuterium is just the normalized linear combination of both spin-up and spin-down, but I want to go further than that.
Thanks