- #1
xAly
- 4
- 0
- Homework Statement
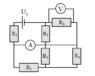
- We have to determine the readings on the ammeter and voltmeter (see picture of circuit), given that ##\text{R}_1 = 4 \Omega, \text{R}_2 = 10 \Omega, \text{R}_3 = 7 \Omega, \text{R}_4 = 8 \Omega, \text{R}_5 = 6 \Omega, \text{R}_6 = 3 \Omega##
- Relevant Equations
- ## \text{R} = \frac{\text{V}}{\text{I}} ##, Resistance in parallel and series.
I get really confused with these types of problems. I don't know how to break down the circuit properly. I tried to go anticlockwise and ignored the ammeter and the voltmeter as circuit components, which gave me R1 and R2 in series while R3 and R5 (in series) are in parallel with R4 and R6 (also in series). This gave me a final equivalent resistance of ##\frac{479}{24}##. But after checking my intermediate solution, it seems this approach is wrong. The solution starts with stating that we can ignore the lower half of the circuit and can only consider the resistors R1, R5 and R6 (so the problem becomes a lot easier). However I don't see why this is true and how one would spot this in similar problems.