ztdep
- 11
- 0
I am evaluating this formula, it's real value is 0. but maple can't further simplify it?
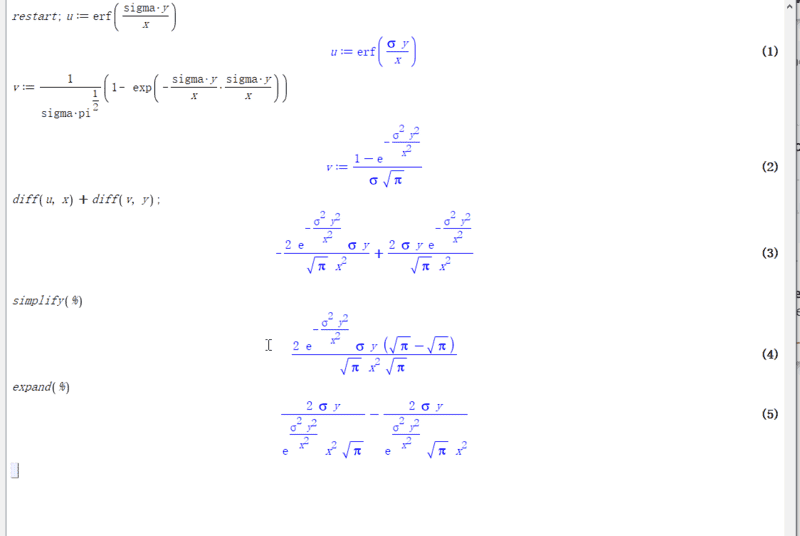
DrClaude said:How can (√π - √π) not be zero?
phyzguy said:Probably because (√π - √π ) isn't necessarily zero. It depends which of the two square roots you take.
To elaborate on @DrClaude's comment, the square root of a positive real number is by convention the principal square root of that number. i.e., the positive square root.DrClaude said:How can (√π - √π) not be zero? We are not solving a quadratic equation here, so ##\sqrt π>0##.
Try enteringztdep said:I am evaluating this formula, it's real value is 0. but maple can't further simplify it?
Pi instead of pi. Conventions are one thing, but what's coded up in the Maple code is another. What's your explanation for why it didn't replace (√π - √π ) in equation 4 of the OP with 0?Mark44 said:To elaborate on @DrClaude's comment, the square root of a positive real number is by convention the principal square root of that number. i.e., the positive square root.
Although the equation ##x^2 - 4 = 0## has two solutions -- x = 2 or x = -2, it is an error to say that ##\sqrt 4 = \pm 2##.
##\sqrt \pi## in Maple must be a positive number. I'd be very surprised if something as basic as that was coded wrongly.phyzguy said:Conventions are one thing, but what's coded up in the Maple code is another.
No idea, but I'm also puzzled why Maple added two expressions whose denominators were both ##\sqrt \pi x^2## to get a single expression with a denominator of ##\sqrt \pi x^2 \sqrt \pi##.phyzguy said:Conventions are one thing, but what's coded up in the Maple code is another. What's your explanation for why it didn't replace (√π - √π ) in equation 4 of the OP with 0?
I agree that's worth a try. @ztdep , can you try replacing pi^(1/2) with Pi^(1/2) in your definition of v and see if that fixes it?Mark44 said:However, I think @S.G. Janssens has hit the nail on the head with his advice to use Pi rather than pi. The Maple documentation backs up this advice - https://www.maplesoft.com/support/help/maple/view.aspx?path=initialconstants.