Why Define Internal Energy Using Average Mechanical Energy?

[member 731016]

Homework Statement

Please see below

Relevant Equations

Please see below

For this,

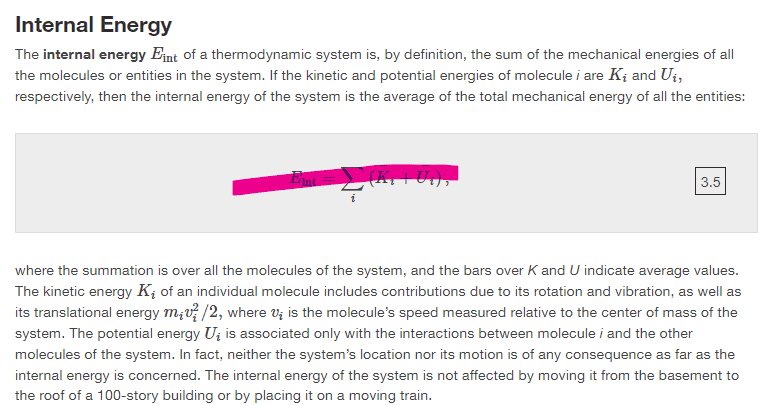
They say internal energy is the sum of the all the mechanical energies of each particle in within the thermodynamic system, however, they then define internal energy differently using the average mechanical energy for all particles within the system (Pink equation). Does someone please know why they did that?

Many thanks!

 

[pbuk]

Does someone please know why they did that?

No, nor why they talk about the "bars over K and U" when there clearly aren't any. You have two choices:

1. find a better book; or
2. use your common sense to eliminate mistakes in trivial definitions.

Posting here to ask us why an unknown author makes mistakes won't help anyone.

 

[member 731016]

No, nor why they talk about the "bars over K and U" when there clearly aren't any. You have two choices:

1. find a better book; or
2. use your common sense to eliminate mistakes in trivial definitions.

Posting here to ask us why an unknown author makes mistakes won't help anyone.

Thank you for your reply @pbuk!

There is actually bars,

I think the highlighter might have made them hard to see.

Sorry I don't have any common sense since I am do not have any other experience with an equation of internal energy. What internal energy equation do you use?

Many thanks!

 

[haruspex]

It looks to me like a conflation of two expressions: $E_{int}=\Sigma_{i=1}^N(K_i+U_i)=N(\bar K+\bar U)$.
But the opening sentence is wrong, it is not the sum of individual kinetic and potential energies.
For the KE, as is later clarified, the sum is over the KEs in the frame of reference of the common mass centre.
For potential energy, the sum over i makes no sense since individual molecules do not have PE. Internal PE resides in the forces between the molecules and the potential of those forces to do work. Further, this is distinct from PE involving external forces.

What is the book?

 

[member 731016]

It looks to me like a conflation of two expressions: $E_{int}=\Sigma_{i=1}^N(K_i+U_i)=N(\bar K+\bar U)$.
But the opening sentence is wrong, it is not the sum of individual kinetic and potential energies.
For the KE, as is later clarified, the sum is over the KEs in the frame of reference of the common mass centre.
For potential energy, the sum over i makes no sense since individual molecules do not have PE. Internal PE resides in the forces between the molecules and the potential of those forces to do work. Further, this is distinct from PE involving external forces.

What is the book?

Thank you for your reply @haruspex!

The textbook is the OpenStax physics volume 2. Here is a link to the section I am referring to: https://openstax.org/books/university-physics-volume-2/pages/3-2-work-heat-and-internal-energy

Many thanks!

 

[member 731016]

Sadly that is the textbook we are using for my course.

 

[haruspex]

Thank you for your reply @haruspex!

The textbook is the OpenStax physics volume 2. Here is a link to the section I am referring to: https://openstax.org/books/university-physics-volume-2/pages/3-2-work-heat-and-internal-energy

Many thanks!

Oh dear. OpenStax openly sux. I have reported hundreds of errors to them, many of which they refuse to fix.

 

[member 731016]

Oh dear. OpenStax openly sux. I have reported hundreds of errors to them, many of which they refuse to fix.

Thank you for your reply @haruspex! Yeah there is a quite a few errors :(

 

[Chestermiller]

It looks to me like a conflation of two expressions: $E_{int}=\Sigma_{i=1}^N(K_i+U_i)=N(\bar K+\bar U)$.
But the opening sentence is wrong, it is not the sum of individual kinetic and potential energies.
For the KE, as is later clarified, the sum is over the KEs in the frame of reference of the common mass centre.
For potential energy, the sum over i makes no sense since individual molecules do not have PE. Internal PE resides in the forces between the molecules and the potential of those forces to do work. Further, this is distinct from PE involving external forces.

What is the book?

Couldn't $U_i$ be considered the amount of work needed to bring a given molecule from infinity to its present location, holding the locations of all other molecules at their present positions?

 

[haruspex]

Couldn't $U_i$ be considered the amount of work needed to bring a given molecule from infinity to its present location, holding the locations of all other molecules at their present positions?

Yes, provided you make it $\frac 12\Sigma U_i$.

 

[member 731016]

Thank you for your replies @Chestermiller and @haruspex!