B Why Does a Body Experience Fictitious Force on Accelerating Objects?

[DA693]

Why does a body experience a fictitious force when placed over an object which is accelerating ? I mean why it doesn't just accelerate with an object... What's actually happening?

 

[Ibix]

Why does a body experience a fictitious force when placed over an object which is accelerating ?

It doesn't. It just keeps doing what it's doing. Howevet, if you attach yourself to the accelerating object and wish to claim that you are stationary then you need to explain why the body is accelerating - and that's what so called fictitious forces (probably better called inertial forces) do.

I mean why it doesn't just accelerate with an object

Why would it, unless it's stuck to the object or otherwise being pulled along by it?

 

[DA693]

It doesn't. It just keeps doing what it's doing. Howevet, if you attach yourself to the accelerating object and wish to claim that you are stationary then you need to explain why the body is accelerating - and that's what so called fictitious forces (probably better called inertial forces) do.

Why would it, unless it's stuck to the object or otherwise being pulled along by it?

If I am stationary and the object on which I am is moving with an acceleration then what will happen it's obvious i will experience a force against that motion and like someone pulled me backwards .. Now my question is why?

Your ans is nice but i don't get the line where you said... "wish to claim that you are stationary then you need to explain why the body is accelerating - and that's what so called fictitious forces (probably better called inertial forces) do."

 

[Ibix]

If I am stationary and the object on which I am is moving with an acceleration then what will happen it's obvious i will experience a force against that motion and like someone pulled me backwards ..

Work in an inertial frame. You are standing on a carpet and someone pulls it forwards. There is a frictional force between your feet and the carpet so your feet move as well. There is no backwards force - you fall over because your feet are no longer under you.

Now work in the non-inertial frame where the carpet is stationary. In this frame, when the carpet is pulled we want it to stay stationary, but if it had a net force on it then it would accelerate. So we need to invoke an inertial force to explain why the carpet doesn't accelerate, and that inertial force accelerates everything backwards at exactly the right rate to cancel the acceleration of the carpet. But you have no other force on you so you do accelerate backwards. The important thing to realize is that inertial forces are just a way of explaining why an accelerating reference object is stationary. You can always choose a non-accelerating reference object and they disappear.

 

[kuruman]

Your ans is nice but i don't get the line where you said... "wish to claim that you are stationary then you need to explain why the body is accelerating - and that's what so called fictitious forces (probably better called inertial forces) do."

Imagine this. Your friend is driving a car and you are on the passenger's side of the front seat. A book is on the back seat. The car's speedometer reads 30 mph and the car is traveling in a straight line. Your friend asks you if the book is moving, you look back and you answer "no it's just sitting there". Farther down the road your friend makes a sharp left turn. While the car is turning, your friend asks you again if the book is moving. You look back and you answer "sure, I see it sliding across the seat to the right."

In this scenario you have asserted that you are stationary, i.e. not moving with respect to the car. The book is also initially stationary same as you. When all of a sudden you see the book slide across the seat, you have to explain what force caused it do so. You invent a name for it and you call it "centrifugal force".

 

[Dale]

experience a fictitious force

Fictitious forces cannot be experienced. They can only be inferred from the motion. There is no experiment or measurement that depends on the fictitious force because they are not physical, they are just mathematical artifacts of using certain coordinate systems.

 

[Ibix]

Fictitious forces cannot be experienced. They can only be inferred from the motion.

This is true in technical speech, but I think it can potentially be a bit confusing. When a bus stops suddenly I would certainly say that my personal experience is that a gravity-like force suddenly pitches me onto my face (some of the bus drivers round here treat bus stops like they're dropping troops into a hot LZ). I guess I'm drawing a distinction between the technical sense of "experience a force", which you can't be experiencing if you can make it go away by a frame choice, and the more mundane sense of "experience" as "my personal impression of what happened".

 

[Dale]

This is true in technical speech, but I think it can potentially be a bit confusing. When a bus stops suddenly I would certainly say that my personal experience is that a gravity-like force suddenly pitches me onto my face (some of the bus drivers round here treat bus stops like they're dropping troops into a hot LZ). I guess I'm drawing a distinction between the technical sense of "experience a force", which you can't be experiencing if you can make it go away by a frame choice, and the more mundane sense of "experience" as "my personal impression of what happened".

That is a good point. Such experiences are just illusions, much like an optical illusion. Your brain takes an actual raw sensation and changes it into something that it isn’t.

 

[gleem]

When a bus stops suddenly I would certainly say that my personal experience is that a gravity-like force suddenly pitches me onto my face

Although you are not being accelerated.

That is a good point. Such experiences are just illusions, much like an optical illusion. Your brain takes an actual raw sensation and changes it into something that it isn’t.

This is an interesting point. Have you been sitting at a stoplight waiting for it to turn green and out of the corner of your eye you notice a car moving forward and you feel a sensation of moving backward and you automatically push on the brake pedal too?

 

[robphy]

Watch the Frame of Reference video (Ivey & Hume, 1960)

• time-stamp 00:33: the intro is a classic (a must-watch clip)
• time stamp 13:27:
  where they begin to discuss non-inertial frames of reference (but you may need to see the preceding sections for the inertial-frame)...
• punchline: 16:10
• rotating-frame: 17:04

 

[Dale]

Although you are not being accelerated.This is an interesting point. Have you been sitting at a stoplight waiting for it to turn green and out of the corner of your eye you notice a car moving forward and you feel a sensation of moving backward and you automatically push on the brake pedal too?

That is an optical illusion, as mentioned above. No actual measurement would corroborate the feeling

 

[A.T.]

...i will experience a force ...

Define "experience a force". Are you talking about your subjective interpretation?

 

[vanhees71]

What you experience when sitting in an accelerating care are not the inertial forces (I don't like 'ficticious force', because there's nothing fictitious in writing down the Newtonian equations of motion in terms of the coordinates of a non-inertial frame of reference) but the forces acting on you to accelerate you wrt. an inertial frame of reference (in this case you can simply take the restframe of the Earth as an approximate inertial frame of reference). E.g., for the care to move on a circle you are also moving in a circle, and to do so you need some centripetal force (as described in an inertial frame of reference). This is a real force due to the electromagnetic interaction between the atoms making up the seat of your care and the atoms making up you ;-)).

 

[italicus]

That is an optical illusion, as mentioned above. No actual measurement would corroborate the feeling

Optical illusion? During their training , astronauts are put into a centrifuge, and experiment accelerations many times greater than g . Centrifuges are used in chemical laboratories to separate heavier particles. Centrifuges are used in washing machines.
The problem with inertial forces is that the reference frame isn’t good to explain why inertial forces arise, the r.f. isn’t inertial. If you want to apply Newton’s law of motion : "F = ma” In an accelerated reference frame , you have to add inertial forces to the real applied force F . This gives the acceleration relative to the non inertial reference frame.

 

[vanhees71]

It's just rewriting the equation of motion "F=ma", which is valid in inertial frames of reference to the coordinates in a non-inertial frame of reference. It reads precisely the same, but a is not simplye $\ddot{x}_j'$, where $x_j'$ are the Cartesian coordinates in the non-inertial frame. To make the occurring equation more intuitive, one brings all the terms in $m a$ which are different from $m \ddot{x}_j'$ to the left-hand side. Then the EoM. look like a Newtonian equation of motion, and one calls the additional terms "inertial forces". That is just mathematics and makes the phenomena occurring in non-inertial reference frames more intuitive, i.e., you have in addition to the "true forces", due to the fundmental interactions between particles, these non-inertial forces like Coriolis and centrifugal force on the rotating Earth, "leading to" the rotation of the plane of oscillations of the Foucault pendulum or the vortex orientation in cyclones and anticyclones on the northern or western hemisphere. Seen from an inertial frame, it's clear that you just have the motion as expected from the "true forces" (and the initial conditions) of the equation of motion "F=ma". I think it becomes very clear in the above quoted movie "Frames of Reference" (posting #10).

 

[italicus]

To make the occurring equation more intuitive, one brings all the terms in ma which are different from mx¨j′ to the left-hand side. Then the EoM. look like a Newtonian equation of motion, and one calls the additional terms "inertial forces". That is just mathematics and makes the phenomena occurring in non-inertial reference frames more intuitive, i.e., you have in addition to the "true forces", due to the fundmental interactions between particles, these non-inertial forces

Intuition must be put aside. But it isn’t mathematics only, it’s physics. An astronaut experiences greater blood pressure in his brain, he can loose senses.
This is the way I teach the problem to students.

 

[vanhees71]

You can describe the astronaut as seen from the inertial frame outside the spaceship he's accelerated against this inertial frame, and then the raise of his blood pressure is clearly due to the "true forces" needed to accelerate him.

 

[italicus]

You can describe the astronaut as seen from the inertial frame outside the spaceship he's accelerated against this inertial frame, and then the raise of his blood pressure is clearly due to the "true forces" needed to accelerate him.

AS you well know, non inertial forces are considered in non-inertial r.f. only .

 

[A.T.]

An astronaut experiences greater blood pressure in his brain,

But not because of inertial forces. Inertial forces can be transformed away via a coordinate change, while the pressure gradient is frame invariant. Explaining frame invariant effects with frame dependent forces makes no sense, because they are already fully explained by the frame invariant forces.

 

[weirdoguy]

But it isn’t mathematics only, it’s physics.

And the physics is that inertial forces are not real. That is why I don't like inertial forces, because some people tend to "explain" a lot of things using those forces and because of that a lot of student don't get what those "forces" really are - a mathematical artifact. A lot of students tend to use them in inertial frames! I always try to solve problems devoted to inertial forces both in inertial and non-inertial refrence frames so that my student see what is the difference.

 

[Dale]

If you want to apply Newton’s law of motion : "F = ma” In an accelerated reference frame , you have to add inertial forces to the real applied force F . This gives the acceleration relative to the non inertial reference frame.

Yes, this is the only effect of the inertial force: to explain the coordinate acceleration in the non-inertial frame. All other effects are due exclusively to the real forces.

An astronaut experiences greater blood pressure in his brain

Yes, and this is due entirely to the real centripetal force. Consider this: where is the blood pressure greatest and hence where are vessels most likely to rupture?

In the back of the brain.

Now, suppose that we are not in a centrifuge but doing linear acceleration in an inertial frame. A forward-directed force causes blood to have high pressure in the back of the brain.

Now, going back to the centrifuge, the forward-directed force is the real centripetal force. The centrifugal force points in the wrong direction to be the cause of the increased pressure. And the amount of pressure increase is entirely explained by the centripetal force, with none leftover to be explained by the centrifugal force.

Furthermore, if we remove the centripetal force (chair breaks) and keep the centrifugal force (stay in rotating frame) then the pressure goes away. Conversely if we keep the centripetal force (chair is intact) and remove the centrifugal force (go to inertial frame) then the pressure stays.

This is the way I teach the problem to students.

You need to be careful what you say to students. It is a fine line between simplifying things to aid in learning of a concept and teaching wrong things that will have to be unlearned later. I don’t know what you are saying, but if you are attributing a real physical effect like the blood pressure to a fictitious force then you are teaching a wrong thing.

 

[pinball1970]

Watch the Frame of Reference video (Ivey & Hume, 1960)

• time-stamp 00:33: the intro is a classic (a must-watch clip)
• time stamp 13:27:
  where they begin to discuss non-inertial frames of reference (but you may need to see the preceding sections for the inertial-frame)...
• punchline: 16:10
• rotating-frame: 17:04

This is great. A fantastic illustration of these concepts (that I don't understand fully)

 

[italicus]

You need to be careful what you say to students. It is a fine line between simplifying things to aid in learning of a concept and teaching wrong things that will have to be unlearned later. I don’t know what you are saying, but if you are attributing a real physical effect like the blood pressure to a fictitious force then you are teaching a wrong thing.

Don’t worry, I am very careful. After having introduced the issue, I receive a lot of questions, to which I start answering with great patience and details, and it takes a lot to arrive at the right conclusion. I am accustomed to discussing with people.

 

[italicus]

Now, going back to the centrifuge, the forward-directed force is the real centripetal force.

The centripetal force is directed towards the axis of rotation.

 

[kuruman]

We haven't from the OP in quite a while and it is not clear whether the ongoing conversation addresses OP's original question. I think that this xkcd cartoon summarizes it well. Sorry for this touch of levity.

 

[Dale]

The centripetal force is directed towards the axis of rotation.

Yes, which is the correct (forward) direction to explain the blood pressure.

 

[vanhees71]

AS you well know, non inertial forces are considered in non-inertial r.f. only .

Exactly, and it's always easier to understand a situation wrt. an inertial reference frame!

 

[A.T.]

I think that this xkcd cartoon summarizes it well.

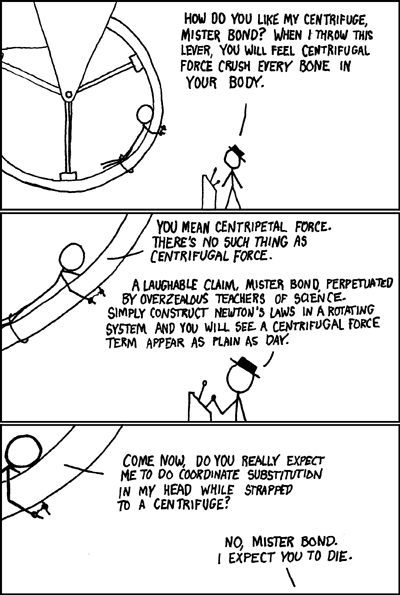
Bond is of course correct here, regarding what will crush his bones: The real centripetal contact force. Just like the real contact force of a bus hitting you is what kills you. This real contact force exists in the rotating frame as well, so no additional explanation via inertial forces is needed for Bond's demise.

The super villain's hand-waving about reconstructing Newton's Laws, applies only to the coordinate acceleration (2nd Law), which is a frame dependent quantity. But It does not apply to bone crushing, which is a frame invariant effect.

 

[italicus]

Exactly, and it's always easier to understand a situation wrt. an inertial reference frame!

Sorry but this is an idea of yours. Having well in mind that the real force is the centripetal one , consider that , more o less, we live in a rotating reference frame, the one of the Earth, and say that the acceleration of gravity , in this rotating frame to which we belong, is less at the equator than at the poles. And the Coriolis force, acting on air masses which moves from the equator to North Pole , deviates them to the right, ok ? The same deviation applies to air masses that move from the NP to the equator : to the right . This causes hurricanes ( Katia...) with a counterclockwise sense of rotation , if seen from “above” , e.g. from meteorological satellites , no ? (I have simplified the real situation, because also centrifugal ( ops!) force is to be considered, and pressure variations too...but let’s be simple). So I think, ( and you can contest of course) that the description of these phenomena is as good from the inertial point of view as from the non inertial point of view.
But consider an example taken from an exercise of mechanics : a conical pendulum , made by a rod which rotates around a vertical axis on which its upper point of suspension is attached ; you can describe motion form the point of view of an external inertial observer, introducing real centripetal forces further to gravity; but you can also describe motion from the point of view of a fly, which is attached to the rod. And in the rotating frame you have to introduce the centrifugal force, don’t you?

I am doing a lot of efforts to write in a decent English !

 

[PeroK]

We haven't from the OP in quite a while and it is not clear whether the ongoing conversation addresses OP's original question. I think that this xkcd cartoon summarizes it well. Sorry for this touch of levity.

View attachment 294423

The last lines could have been "Do you expect me to torque?", "No, Mister Bond, I expect you to die!"

 

[Dale]

you can also describe motion from the point of view of a fly, which is attached to the rod. And in the rotating frame you have to introduce the centrifugal force, don’t you?

This is correct. To explain the motion in the non-inertial frame you introduce the inertial forces. This is the only effect of the inertial forces.

The measurements of accelerometers or strain gauges or any other measurement device depend only on the real forces.

 

[PeroK]

Exactly, and it's always easier to understand a situation wrt. an inertial reference frame!

This homework problem was a lot easier to solve in the rotating reference frame using the centrifugal force:

https://www.physicsforums.com/threads/mass-m-sliding-without-friction-inside-a-rotating-tube.1006329

 

[jbriggs444]

Personally, I have no problem adopting a non-inertial frame and dealing with inertial forces. Just like the 99.9% of all first year physics students who are taught about the inertial force we call gravity.

 

[gleem]

I agree with @vanhees71, fictitious force is not a good term. How about apparent force instead?

 

[DA693]

Work in an inertial frame. You are standing on a carpet and someone pulls it forwards. There is a frictional force between your feet and the carpet so your feet move as well. There is no backwards force - you fall over because your feet are no longer under you.

Now work in the non-inertial frame where the carpet is stationary. In this frame, when the carpet is pulled we want it to stay stationary, but if it had a net force on it then it would accelerate. So we need to invoke an inertial force to explain why the carpet doesn't accelerate, and that inertial force accelerates everything backwards at exactly the right rate to cancel the acceleration of the carpet. But you have no other force on you so you do accelerate backwards. The important thing to realize is that inertial forces are just a way of explaining why an accelerating reference object is stationary. You can always choose a non-accelerating reference object and they disappear.

Work in an inertial frame. You are standing on a carpet and someone pulls it forwards. There is a frictional force between your feet and the carpet so your feet move as well. There is no backwards force - you fall over because your feet are no longer under you.

Now work in the non-inertial frame where the carpet is stationary. In this frame, when the carpet is pulled we want it to stay stationary, but if it had a net force on it then it would accelerate. So we need to invoke an inertial force to explain why the carpet doesn't accelerate, and that inertial force accelerates everything backwards at exactly the right rate to cancel the acceleration of the carpet. But you have no other force on you so you do accelerate backwards. The important thing to realize is that inertial forces are just a way of explaining why an accelerating reference object is stationary. You can always choose a non-accelerating reference object and they disappear.

Define "experience a force". Are you talking about your subjective interpretatio

Yr

Define "experience a force". Are you talking about your subjective interpretation?

 

[DA693]

I got that fictitious force are just for explanation when a person is in accelerating frame of reference or in non inertial frame. Alright but if you look into, then there are lots of scenario like in the video of Dr. hume ... A ball falling in an accelerating frame ok i got that... A puck sliding over the table , I got that too... But when am in the car or let's say I put a box over the table and I pulled a table or accelerates it towards myself then I am in which frame? If non inertial then it explains why box moves backwards if not then again WHY? If am in inertial frame then why it's moving backwards or it seems to be moving backwards because I am accelerating the table.

 

[jbriggs444]

I got that fictitious force are just for explanation when a person is in accelerating frame of reference or in non inertial frame. Alright but if you look into, then there are lots of scenario like in the video of Dr. hume ... A ball falling in an accelerating frame ok i got that... A puck sliding over the table , I got that too... But when am in the car or let's say I put a box over the table and I pulled a table or accelerates it towards myself then I am in which frame? If non inertial then it explains why box moves backwards if not then again WHY? If am in inertial frame then why it's moving backwards or it seems to be moving backwards because I am accelerating the table.

A frame is something that you write down with pencil and paper, not something that you climb into.

You can decide to treat your car as being at rest while objects accelerate across the seats. Or you can decide to treat your car as accelerating around a corner while objects on the seats continue to move in straight lines. Both descriptions of the situation work. Both lead to a correct prediction -- the objects and the door collide.

 

[Dale]

when am in the car or let's say I put a box over the table and I pulled a table or accelerates it towards myself then I am in which frame?

As @jbriggs444 said, you are in whichever frame you prefer to analyze. Or if you really like doing extra work you are in both frames. Or as many as you have patience to analyze the scenario in.

The reference frame is a part of the analysis, not the physics. The whole point of relativity is that you can do the analysis in any frame you like for whatever reason you want.

 

[DA693]

As @jbriggs444 said, you are in whichever frame you prefer to analyze. Or if you really like doing extra work you are in both frames. Or as many as you have patience to analyze the scenario in.

The reference frame is a part of the analysis, not the physics. The whole point of relativity is that you can do the analysis in any frame you like for whatever reason you want.

As @jbriggs444 said, you are in whichever frame you prefer to analyze. Or if you really like doing extra work you are in both frames. Or as many as you have patience to analyze the scenario in.

The reference frame is a part of the analysis, not the physics. The whole point of relativity is that you can do the analysis in any frame you like for whatever reason you want.

Sir i am actually trying to convince my brain that it is what it is but again I ask myself why that box move from stationary point of view or inertial. I have no answer or the answer is something which is not real...you all trying to explain and I am really thankful to all of you .But sorry to say I am not getting the actual point here.

 

[Dale]

why that box move from stationary point of view or inertial.

From the point of view of the inertial frame any acceleration of the box would be due to the friction with the table or whatever it is on. The box does not accelerate backwards.

 

[italicus]

@DA693
Be careful. First of all, a “reference frame “ isn’t a system of coordinates; a rf is made of physical bodies to which you refer you state: for example, in your room a rf can be represented by floor and walls. Only after you can choose coordinates, f.e. the distances from the walls. Math comes after physics.
Secondly: in your rf , inertial by hypothesis, there is a table at rest, and there is a bead at rest on the the table. Suppose there is no friction between bead and table, and push the table; then wrt to you the bead rests in its position, why? Because of the principle of inertia; note that there are two forces only on the bead,weight and table reaction, which equilibrate.
But wrt the table the bead accelerates backwards, why? Because the table is accelerating forward wrt to you , its acceleration is a vector A ; a little green man firmly sitting on the table says that a inertial force - mA arose, accelerating the bead backwards. The table is a rf for the little green man and the bead, that doesn’t move with rectilinear uniform motion wrt to YOU.

 

[vanhees71]

Sorry but this is an idea of yours. Having well in mind that the real force is the centripetal one , consider that , more o less, we live in a rotating reference frame, the one of the Earth, and say that the acceleration of gravity , in this rotating frame to which we belong, is less at the equator than at the poles. And the Coriolis force, acting on air masses which moves from the equator to North Pole , deviates them to the right, ok ? The same deviation applies to air masses that move from the NP to the equator : to the right . This causes hurricanes ( Katia...) with a counterclockwise sense of rotation , if seen from “above” , e.g. from meteorological satellites , no ? (I have simplified the real situation, because also centrifugal ( ops!) force is to be considered, and pressure variations too...but let’s be simple). So I think, ( and you can contest of course) that the description of these phenomena is as good from the inertial point of view as from the non inertial point of view.
But consider an example taken from an exercise of mechanics : a conical pendulum , made by a rod which rotates around a vertical axis on which its upper point of suspension is attached ; you can describe motion form the point of view of an external inertial observer, introducing real centripetal forces further to gravity; but you can also describe motion from the point of view of a fly, which is attached to the rod. And in the rotating frame you have to introduce the centrifugal force, don’t you?

I am doing a lot of efforts to write in a decent English !

Sure, if you want to treat phenomena on Earth where you have to take into account its rotation, it's convenient to use the non-inertial rest frame of the Earth (Foucault pendula, winds in meteorology and the like) and think in terms of inertial forces. That's what I said in one of the postings above too.

 

[A.T.]

a rf is made of physical bodies to which you refer you state:

You can use physical bodies to define a reference frame, but the reference frame is not "made of" physical bodies. For example: You can define a reference frame where some physical body undergoes a certain motion, but no physical body is actually at rest in that frame. So it does not make sense to say that the "reference frame is made of physical bodies"

 

[Dale]

a “reference frame “ isn’t a system of coordinates; a rf is made of physical bodies to which you refer you state

Please be aware that the professional scientific literature is inconsistent in this. While there are indeed authors who use the term as you describe, there are also authors who use it to mean either a coordinate system or a tetrad. So both usages are considered valid here on PF.

 

[italicus]

Please be aware that the professional scientific literature is inconsistent in this. While there are indeed authors who use the term as you describe, there are also authors who use it to mean either a coordinate system or a tetrad. So both usages are considered valid here on PF.

Well, I consider my reference authors, among which there is professor Elio Fabri, previous teacher at the University of Pisa. Ever heard? Look for him on the web.

 

[ergospherical]

 

[Ibix]

Well, I consider my reference authors, among which there is professor Elio Fabri, previous teacher at the University of Pisa. Ever heard? Look for him on the web.

Your construction is fine, but it is not the only one in wide use. Please can we not argue about which one is "better" again? It's literally an argument about whether we attach the lab to the reference frame or the reference frame to the lab.

 

[weirdoguy]

among which there is professor Elio Fabri, previous teacher at the University of Pisa. Ever heard? Look for him on the web.

Argument from authority is not a good argument. Again - stating that "a “reference frame “ isn’t a system of coordinates" is not consistent with literature, there are different definitions, and Elio Fabri won't change that.

 

[italicus]

Argument from authority is not a good argument. Again - stating that "a “reference frame “ isn’t a system of coordinates" is not consistent with literature, there are different definitions, and Elio Fabri won't change that.

I don’t agree with you. I have cited Fabri as my reference author, you are free to think differently. Which literature, please? Make some example!
It seems that I am fighting a battle against a lot of you, here , every moment…
I have simply given an example to DA693, to make him understand .
We should listen to ergo spherical: not again…

 

[Ibix]

don’t agree with you. I have cited Fabri as my reference author, you are free to think differently. Which literature, please?

Schutz defines a reference frame as a one form or a velocity in his text. Taylor and Wheeler simply define them by the properties of inertial bodies with respect to such a frame. The first is abstract, the second is closer to your definition

"Reference frame" is just words. Not everybody uses them in quite the same way and none is "righter" than the other. This is far from the only thing in physics where multiple conventions are in common use, so this shouldn't be any particular surprise.