Why Does Integration Matter in Solving for the Average Value of a Function?

  • Context: MHB 
  • Thread starter Thread starter nghijen
  • Start date Start date
Click For Summary

Discussion Overview

The discussion revolves around understanding the concept of the average value of a function and the role of integration in calculating it. Participants are exploring the application of definite integrals over a specified interval and addressing misunderstandings related to the integration process.

Discussion Character

  • Exploratory
  • Technical explanation
  • Debate/contested
  • Mathematical reasoning

Main Points Raised

  • One participant expresses confusion about solving for the average value of a function and seeks assistance.
  • Another participant states that the average value involves a definite integral over an interval, questioning if the interval is [0, u].
  • A similar assertion about the interval being [0, u] is repeated by another participant.
  • A participant provides a formula for the average value of a function, suggesting integration over the interval [0, u].
  • Another participant critiques the approach taken by the previous contributor, emphasizing the importance of identifying where the function equals zero and pointing out a misunderstanding in the integration process.
  • This participant also corrects a mathematical error regarding the evaluation of a specific expression related to the function.

Areas of Agreement / Disagreement

There is no consensus on the understanding of the integration process or the correct approach to finding the average value of the function. Multiple viewpoints and critiques are present, indicating ongoing disagreement and confusion.

Contextual Notes

Participants have not fully resolved the assumptions regarding the interval for integration or the steps required for proper integration. There are also unresolved mathematical steps and potential misunderstandings about the function's behavior.

nghijen
Messages
2
Reaction score
0
I have attempted to solve the question but I still do not understand. Can someone please help me?
IMG_5255.jpeg
 
Physics news on Phys.org
average value of a function involves a definite integral over an interval ... from your shading, is the given interval $[0,u]$ ?
 
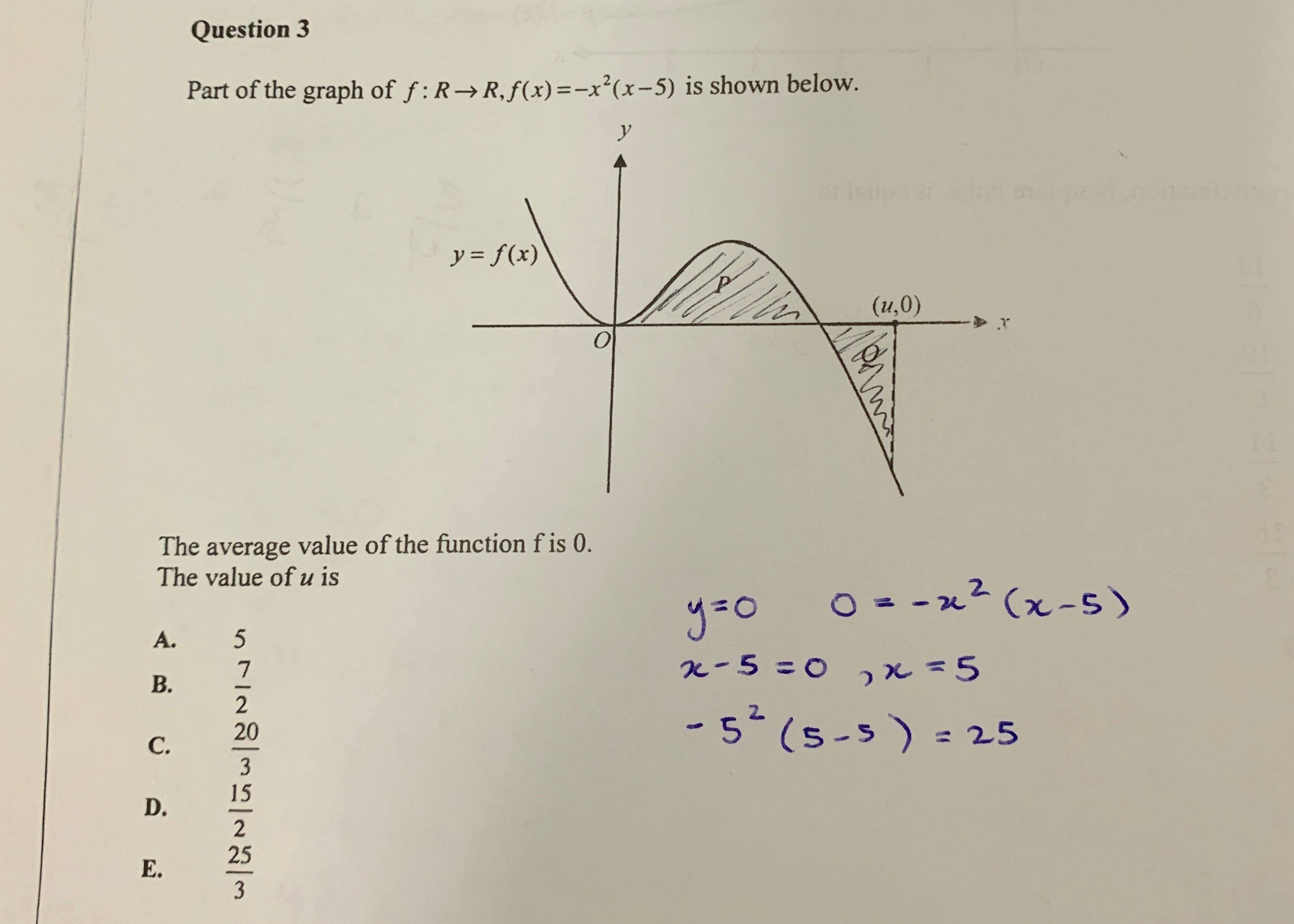
skeeter said:
average value of a function involves a definite integral over an interval ... from your shading, is the given interval $[0,u]$ ?

I am assuming it is [0, u] !
 
nghijen said:
I am assuming it is [0, u] !

$\displaystyle 0 = \dfrac{1}{u-0} \int_0^u x^2(5-x) \, dx$

work it ...
 
I frankly can't understand what you think you are doing! Much of what you are doing is determining where f(x)= 0. Okay that is at x= 5, which you have, and x= 0, which you ignore, but that is irrelevant anyway! The average value of a function, f(x), over interval a to b is the integral of f, from a to b, divided by b- a. But you show no attempt at integration!

Oh, and $-5^2(5- 5)= 0$, not -25! That is why you got x= 5 as a solution to $-x^2(x- 5)= 0$!
 
Last edited:

Similar threads

MHB Using advanced calculus for finding values
  • · Replies 2 ·
Replies
2
Views
1K
High School Some questions while I self-learn Applied Calculus
  • · Replies 49 ·
2
Replies
49
Views
7K
High School Does U-Substitution Function as the Inverse of the Chain Rule?
  • · Replies 10 ·
Replies
10
Views
3K
MHB Indefinite integral in division form
  • · Replies 1 ·
Replies
1
Views
2K
High School Explain Integration to me, please
  • · Replies 5 ·
Replies
5
Views
3K
Undergrad Infinite Series - Divergence of 1/n question
  • · Replies 5 ·
Replies
5
Views
2K
Undergrad A function with no max or min at an endpoint
  • · Replies 7 ·
Replies
7
Views
2K
High School Why don't we account for the constant in integration by parts?
  • · Replies 16 ·
Replies
16
Views
4K
Undergrad Integration with different infinitesimal intervals
  • · Replies 31 ·
2
Replies
31
Views
4K
Undergrad Ambiguity of the term "indefinite integral"
  • · Replies 11 ·
Replies
11
Views
2K