Dario56
- 289
- 48
Molecular potential energy of hydrogen in dependence with atomic distance for bonding orbital is given by picture below.
We can see that at large distances force between atoms is attractive and potential energy drops to minimum which corresponds to bond energy and length. This part of the curve looks very similar to interaction between charges of opposite sign at large enough distances (Couloumb interactions)
After that interatomic potential energy starts increasing and at some distance force becomes repulsive.
My question is why does molecular potential energy curve have that shape? Why is it that potential energy first drops to minimum and than starts increasing?
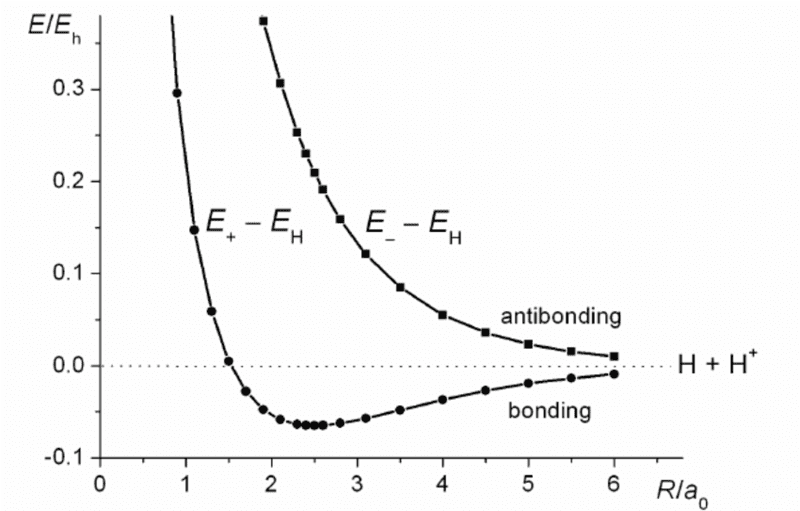
We can see that at large distances force between atoms is attractive and potential energy drops to minimum which corresponds to bond energy and length. This part of the curve looks very similar to interaction between charges of opposite sign at large enough distances (Couloumb interactions)
After that interatomic potential energy starts increasing and at some distance force becomes repulsive.
My question is why does molecular potential energy curve have that shape? Why is it that potential energy first drops to minimum and than starts increasing?