Why does the war-wolf reach its maximum speed at the vertical position?

[member 731016]

Homework Statement

Pls see below

Relevant Equations

Pls see below

For this problem,

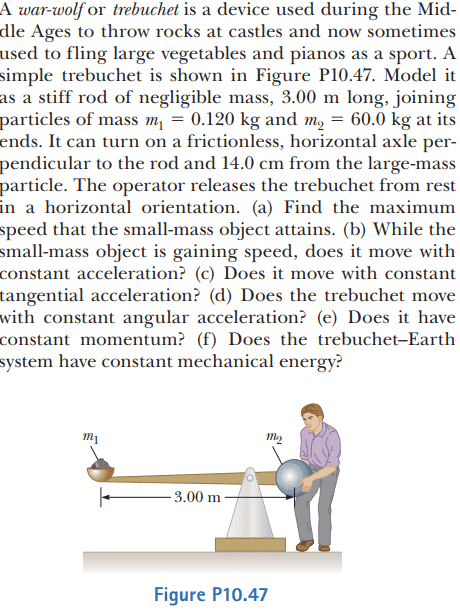
For part(a) the solution is,

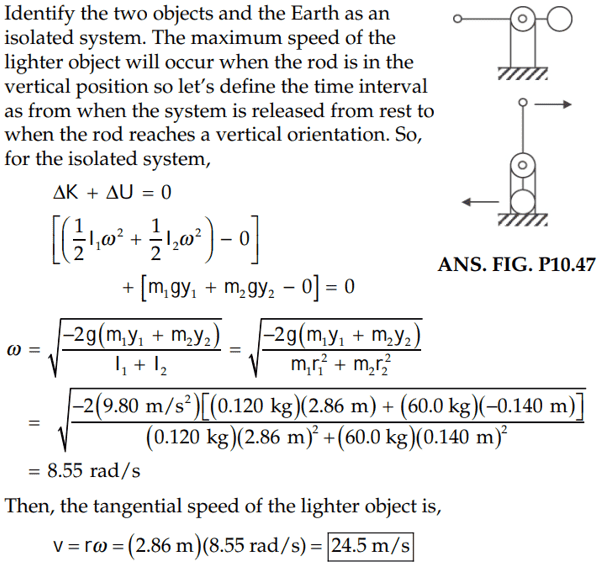
However, how did they know that the max speed is reached in the vertical position?

For part (d) the solution is,

However, I thought the solution would be because there is net gravitational torque in clockwise direction (torque due to small mass – torque due big mass) then angular momentum is not conserved.

I don’t understand why they talk only about the lever arm of the 60 kg mass, as there is also a lever arm for the smaller mass. I agree thought about their statement that the lever arm due to the masses on each end changes as the war-wolf rotates.

Many thanks!

 

[haruspex]

then angular momentum is not conserved.

Whether the acceleration is a nonzero constant or varying, angular momentum is not constant. The question is whether the acceleration is constant, so considering angular momentum is not going to help.

 

[haruspex]

I don’t understand why they talk only about the lever arm of the 60 kg mass, as there is also a lever arm for the smaller mass.

I don't see mention of a lever arm in the extracts you posted.
Yes, each weight has its lever arm.

 

[haruspex]

how did they know that the max speed is reached in the vertical position?

Before it reaches vertical, is the system getting faster or slower?
What about after vertical?

 

[member 731016]

Whether the acceleration is a nonzero constant or varying, angular momentum is not constant. The question is whether the acceleration is constant, so considering angular momentum is not going to help.

I don't see mention of a lever arm in the extracts you posted.
Yes, each weight has its lever arm.

Thank you for your replies @haruspex !

 

[member 731016]

Before it reaches vertical, is the system getting faster or slower?
What about after vertical?

Thank you for your reply @haruspex !

The system is getting faster I think because the system as a whole is losing gravitational potential energy (big mass losing GPE at a faster rate than GPE gain by small mass - even though they have same tangential speed)

Many thanks!

 

[haruspex]

Thank you for your reply @haruspex !

The system is getting faster I think because the system as a whole is losing gravitational potential energy (big mass losing GPE at a faster rate than GPE gain by small mass - even though they have same tangential speed)

Many thanks!

And what about after passing the vertical position?

 

[BvU]

even though they have same tangential speed

Actually, no! Since $v = \omega r$ and $\bf \omega$ is the same for both...

$\$

 

[Lnewqban]

... big mass losing GPE at a faster rate than GPE gain by small mass...

Could you explain that a little further?
Can you see a single imaginary mass that is equivalent to both masses and that is located at certain point between the pivot and the big ball?

 

[member 731016]

And what about after passing the vertical position?

Thank you for your reply @haruspex !

I think it slows down since the big mass starts gaining GPE

Many thanks!

 

[member 731016]

Actually, no! Since $v = \omega r$ and $\bf \omega$ is the same for both...

$\$

Thank you for your reply @BvU!

True, good you mentioned that!

 

[member 731016]

Could you explain that a little further?
Can you see a single imaginary mass that is equivalent to both masses and that is located at certain point between the pivot and the big ball?

Thank you for your reply @Lnewqban !

Do you mean the COM as an imaginary mass equivalent to both masses?

Many thanks!

 

[haruspex]

Thank you for your reply @haruspex !

I think it slows down since the big mass starts gaining GPE

Many thanks!

Right again.
If it accelerates all the while before reaching vertical and decelerates thereafter, when is it rotating fastest?

 

[member 731016]

Right again.
If it accelerates all the while before reaching vertical and decelerates thereafter, when is it rotating fastest?

Thank you for your reply @haruspex!

As the war wolf passes the top there is a moment where there is only centripetal acceleration and no tangential acceleration since the war wolf is parallel to gravity. However, since it is still rotating it makes at angle $d\theta$ with the vertical so it has a tangential acceleration again that increases. I guess I could of just said that the top is at unstable equilibrium.

If I reason from mechanical energy conservation, then the system has the least amount of potential energy when the little mass it at the top and the big mass is from the bottom. Therefore, as there is no non-conservative forces acting then the system must have the largest rotational kinetic energy.

Many thanks!

 

[haruspex]

However, since it is still rotating it makes at angle dθ with the vertical so it has a tangential acceleration again that increases.

I can't follow your reasoning there. You accepted it accelerates until vertical and decelerates thereafter. So when is it rotating fastest? It's a simple enough deduction.

the system has the least amount of potential energy when the little mass it at the top and the big mass is from the bottom. Therefore, as there is no non-conservative forces acting then the system must have the largest rotational kinetic energy.

Yes, that's another way to get there.

 

[Lnewqban]

Yes
Or just for understanding the principle on which the system works, you could disregard the little mass.
The little mass induces a CCW moment that is 24.5 times smaller than the CW moment that the big mass induces.

Then, you have a mass oscillating about a pivot after being released from a horizontal position.

 

[member 731016]

Yes
Or just for understanding the principle on which the system works, you could disregard the little mass.
The little mass induces a CCW moment that is 24.5 times smaller than the CW moment that the big mass induces.

Then, you have a mass oscillating about a pivot after being released from a horizontal position.

View attachment 322757

Thank you for your help @Lnewqban !

 

[member 731016]

I can't follow your reasoning there. You accepted it accelerates until vertical and decelerates thereafter. So when is it rotating fastest? It's a simple enough deduction.

Yes, that's another way to get there.

Thank you for your reply @haruspex!

What was the reasoning you were expecting me to give?

Sorry I think I was meant to say that before it reaches the vertical it is deaccelerating and after it reach's it is accelerating (tangentially).

Many thanks!

 

[haruspex]

Sorry I think I was meant to say that before it reaches the vertical it is deaccelerating and after it reach's it is accelerating (tangentially).

Eh? I already agreed with you it is the other way: accelerating until vertical, decelerating thereafter. I am asking what tells you about where it reaches maximum speed.

 

[member 731016]

Eh? I already agreed with you it is the other way: accelerating until vertical, decelerating thereafter. I am asking what tells you about where it reaches maximum speed.

Thank you for your reply @haruspex !

Yes sorry, I agree that it accelerates until vertical an deaccelerates thereafter. The reason it reaches max speed at the vertical is because it keeps accelerating until its reach's the vertical and deaccelerates afterwards. The acceleration = 0 at the vertical.

I think we could also integrate the acceleration graph to find that the velocity is max at the vertical.

Many thanks!