Why Doesn't a Spinning Wheel Drop Due to Its Own Weight?

[GhostLoveScore]

This is the experiment

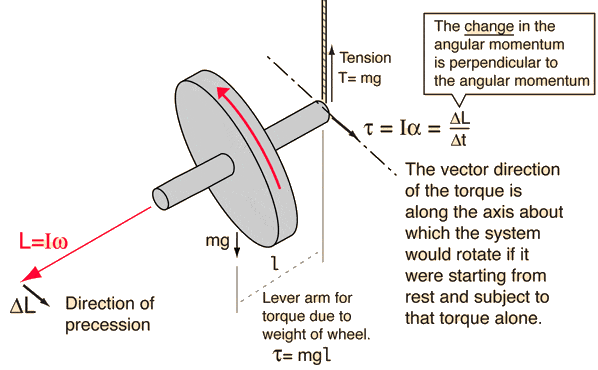
I am a bit confused about this part:
There is a torque on the wheel from its own weight. That's the τ on the picture.

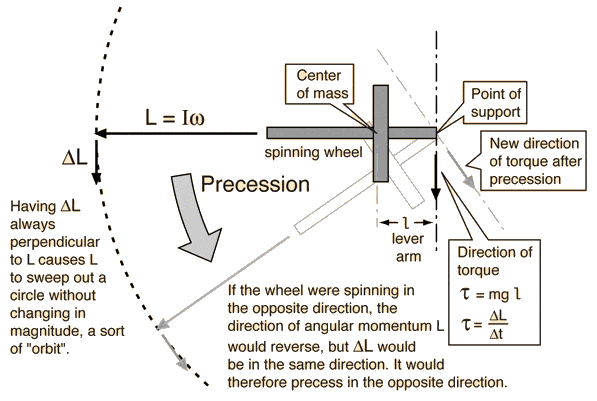
In this perspective, τ points at the bottom of the page. I understand that that torque changes angular momentum of the wheel and the wheel starts precessing because of that change.

What is bothering me is that I can't figure out what is opposing the torque τ so that the wheel doesn't drop as it would when it wouldn't rotate. There has to be a torque that has the opposite direction. Or does it?

Is it angular momentum that is preventing the wheel from dropping?
It's confusing because I would expect that the wheel would slowly be dropping (it's angle going from horizontal to vertical) as well as precessing. Maybe it does happen like that, but very slowly? And I am not talking about energy losses on friction.

EDIT: and one additional question. Since the wheel was not precessing before we let it go, and it started precessing after that, wouldn't you say that for a short time there was torque pointed up that started the precession of the wheel?

If somebody could explain that a bit better-

 

[Ivanov]

the torque opposes itself on the other side. It's just that because of the angle it is slightly greater on one end and thus the rotation. When it starts falling it goes from having to not having torque.

 

[Dale]

There has to be a torque that has the opposite direction. Or does it?

No, if there were another torque canceling out the gravitational torque then it would not precess. The precession is precisely due to the fact that there is a net torque.

 

[GhostLoveScore]

So, is any energy lost in the precession? In ideal experiment. Will is precess endlessly or will the energy be lost over time?

Also, if there is torque, it should increase wheel's angular momentum. This wheel starts precessing and then it continues to precess with constant angular speed. If there was torque after it started precessing, it would precess faster and faster, shouldn't it?

 

[jbriggs444]

So, is any energy lost in the precession? In ideal experiment. Will is precess endlessly or will the energy be lost over time?

Also, if there is torque, it should increase wheel's angular momentum. This wheel starts precessing and then it continues to precess with constant angular speed. If there was torque after it started precessing, it would precess faster and faster, shouldn't it?

Torque has direction. Is the torque from gravity constant? Or merely constant in magnitude?

 

[GhostLoveScore]

Torque from gravity is constant in magnitude, but not in the direction. Direction changes as the wheel precesses, it's always perpendicular to wheel's angular momentum from spinning.

When we let go of the wheel and leave it supported just on one side, does it drop down by a small amount? I mean, does its angular momentum changes direction from perfectly horizontal to slightly down, few degrees down from horizontal?

 

[GhostLoveScore]

the torque opposes itself on the other side. It's just that because of the angle it is slightly greater on one end and thus the rotation. When it starts falling it goes from having to not having torque.

How exactly? Why does it lose torque from gravitation when it starts precessing?

 

[Dale]

So, is any energy lost in the precession? In ideal experiment. Will is precess endlessly or will the energy be lost over time?

Energy is not lost to precession itself, just the usual dissipation and energy exchange mechanisms.

If there was torque after it started precessing, it would precess faster and faster, shouldn't it?

Only if the torque were in the same direction as the angular momentum. This is the same as in linear motion: forces parallel to momentum increase the speed but forces perpendicular to momentum change the direction of the momentum. Similarly: torques parallel to the angular momentum increase angular speed but torques perpendicular to angular momentum change the direction of the angular momentum.

 

[Dale]

When we let go of the wheel and leave it supported just on one side, does it drop down by a small amount? I mean, does its angular momentum changes direction from perfectly horizontal to slightly down, few degrees down from horizontal?

It can, depending on the details. This motion is called nutation.

 

[GhostLoveScore]

I'll try to explain the problem a bit better. What if I would hold the wheel with two hands, one hand at the point there string is attached and the other on the other end of the shaft. I will spin the wheel. Then I will move my left hand down, trying to simulate torque from gravitation.
And the wheel of course, turns counterclockwise.

And what is bothering me that I had to turn the wheel by hand for it to rotate counterclockwise - in the direction of applied torque. But torque from gravity is not turning the wheel in the direction of that torque, and yet the wheel again rotates counterclockwise. How is that possible?

On the other hand, I tried rotating the wheel counterclockwise and it produces the torque that is working against torque from gravitation. Is this what is keeping the wheel from not falling? Torque from gravitation changes angular momentum of the wheel so that it starts precessing counterclockwise. And because it's precessing counterclockwise it produces torque that is opposing torque from gravity?

 

[Dale]

I'll try to explain the problem a bit better. What if I would hold the wheel with two hands, one hand at the point there string is attached and the other on the other end of the shaft. I will spin the wheel. Then I will move my left hand down, trying to simulate torque from gravitation.
And the wheel of course, turns counterclockwise.

I am not certain that I am understanding your scenario. Let's assume a coordinate system with x horizontally to the left, y horizontally from front to back, and z vertically upwards, and with your right hand at the origin. So the axle is in both hands with the gyro spinning such that there is a large angular momentum to the left (+x). There is an upwards force at each hand and the weight at the center. The upwards force at the right hand produces no torque because the r is 0. The downwards weight produces a torque in the y direction, and the upwards force at the left hand produces a torque in the -y direction. The weight is twice the force but half the radius, so the torques cancel out.

Sound right?

 

[GhostLoveScore]

That's right.
And then with my left hand I rotate the gyro in xz plane, some 45 degrees down. The gyro will produce torque in z direction. We rotated the gyro and it produced torque.

And yet, if the gyro is instead suspended on a thread only on one side, gravity will produce torque on the wheel and the wheel will start precessing, but the wheel will not rotate as in my first example.

 

[Dale]

And then with my left hand I rotate the gyro in xz plane, some 45 degrees down. The gyro will produce torque in z direction. We rotated the gyro and it produced torque.

Yes, you are changing the direction of the angular momentum from x to x, -z. That requires a torque in the -z direction, and by Newtons 3rd law you will feel an equal and opposite torque in the z direction.

And yet, if the gyro is instead suspended on a thread only on one side, gravity will produce torque on the wheel and the wheel will start precessing, but the wheel will not rotate as in my first example

Yes, the torque from gravity is in the y direction, not in the z direction. So you would not expect it to rotate in the same direction as a z torque.

 

[GhostLoveScore]

Yes, you are changing the direction of the angular momentum from x to x, -z. That requires a torque in the -z direction, and by Newtons 3rd law you will feel an equal and opposite torque in the z direction.

Ah, that was my first misunderstanding. I thought that I applied torque in y direction.

Yes, the torque from gravity is in the y direction, not in the z direction. So you would not expect it to rotate in the same direction as a z torque.

Ok, torque from gravity is in the y direction. Than the thread by which wheel hangs feels torque in -y direction?

 

[jbriggs444]

Than the thread by which wheel hangs feels torque in -y direction?

The thread by which the wheel hangs is not accelerating nor is its angular momentum changing. It is subject to balanced forces only. Why would you think it is under a torque?

 

[GhostLoveScore]

I think I am beginning to understand something. Tell me, what would happen if I would stop the wheel from precessing, but if I would do it without the friction, not to slow wheel's spinning?

I forgot that torque in one direction doesn't mean that angular momentum will point in that direction. I totally forgot that. Angular momentum is a vector and should be added as a vector.

 

[Dale]

Ok, torque from gravity is in the y direction. Than the thread by which wheel hangs feels torque in -y direction?

What thread? The wheel has three forces on it: the force at the right hand, the force at the left hand, and the force of gravity.

There is no torque at the right hand because r=0. There is a torque in the y direction due to gravity. Depending on the force on the left hand there can be a torque in any direction.

 

[Dale]

I think I am beginning to understand something. Tell me, what would happen if I would stop the wheel from precessing, but if I would do it without the friction, not to slow wheel's spinning?

That is the situation in the beginning. The left hand applies a torque which opposes the gravitational torque. I assume there is a handle with bearings so that can be done as you say, without friction.

Angular momentum is a vector and should be added as a vector.

Yes, that is very important

 

[scottdave]

Gyroscopic precession is not an easy concept to grasp. There are many videos on Youtube which show what is happening.
Here are a couple. and

Also, you may come across some which use the new fad: Fidget Spinners. Most of their mass is near the edge, so they make decent gyroscopes, if spun fast enough.

 

[GhostLoveScore]

Actually, my cousin has one of those spinners. It's very interesting. In the meantime since I posted this, I now understand precession.