Why doesn't ΔU = 0 (U3= U1) in this problem, where pV = constant?

[grotiare]

EDIT: My bad, this is from my professors lecture, but I assume this question is more suitable for the homework section of this forum

The problem gives that pV = constant, and thus is assumed to be an isothermal process. However, the given U1 and U3 are not equal to each other. Is it because it is not specified the gas is ideal that we ignore that ΔU = 0 ?

 

[Delta2]

Yes I think that's the catch, it is not specified that the gas is ideal.

You can solve this problem, without assuming that gas is ideal and without using $PV=nRT$ (or $\Delta U=nC_V\Delta T$). Just calculate the total work done in the cycle and then use 1st law of thermodynamics for the cycle.

For the part of the cycle that $pV=constant$ you need to calculate that constant $C$ first, and then the work will be $$W_{3\to1}=\int_{V_3}^{V_1}\frac{C}{V}dV$$

P.S The solution I see to this problem , doesn't use anywhere the given values of $U_1,U_3$.

 

[grotiare]

Yes I think that's the catch, it is not specified that the gas is ideal.

You can solve this problem, without assuming that gas is ideal and without using $PV=nRT$ (or $\Delta U=nC_V\Delta T$). Just calculate the total work done in the cycle (as the total area in a PV diagram that is enclosed by the cycle) and then use 1st law of thermodynamics for the cycle.

For the part of the cycle that $pV=constant$ you need to calculate that constant $C$ first, and then the work will be $$W_{3\to1}=\int_{V_3}^{V_1}\frac{C}{V}dV$$

Okay cool thank you! So it would thus just be this process-> C = constant = PV, and thus you would integrate 1/V dV from V1 to V3 (leaving c=pv outside of integral) to get W3-1 = PV*ln(V1/V3), where PV = P1V1 or P3V3

 

[Delta2]

Okay cool thank you! So it would thus just be this process-> C = constant = PV, and thus you would integrate 1/V dV from V1 to V3 (leaving c=pv outside of integral) to get W3-1 = PV*ln(V1/V3), where PV = P1V1 or P3V3

Yes that's correct. And also very easily you can calculate the work from $1 \to 2$ (isochoric process) and $2\to 3$ (isobaric process).

 

[jack action]

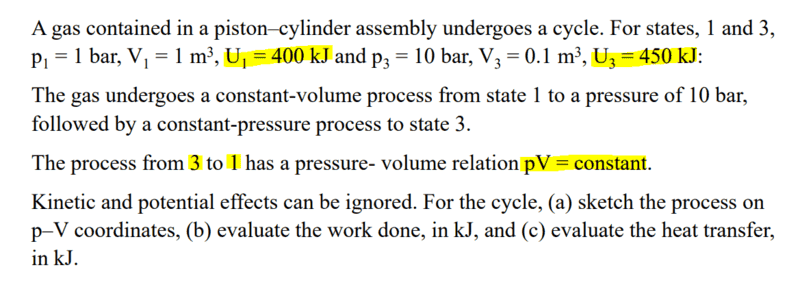
The problem gives that pV = constant, and thus is assumed to be an isothermal process.

Wrong assumption.

You have 2 processes - one constant volume, one constant pressure - for which you can calculate the pressure-volume work. (part b)

Then you use the first law to calculate the heat transfer. (part c)