rtareen
- 162
- 32
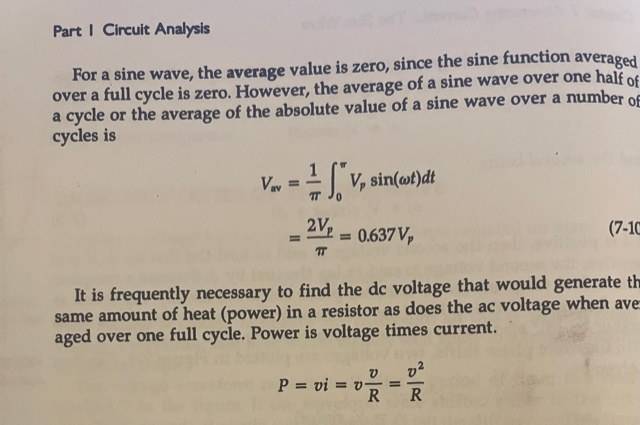
Below is the really quick derivation for average voltage. However when we do the anti-derivative a factor of ##1/\omega## should come out and the full answer should be ##\frac{2V_p}{\pi \omega}##. So why don't we include that? What's going on?