Why is φ Assumed to Be 90 in the Undamped Forced Oscillator Solution?

[member 731016]

Homework Statement

Please see below

Relevant Equations

Please see below

For this problem,

The solution is,

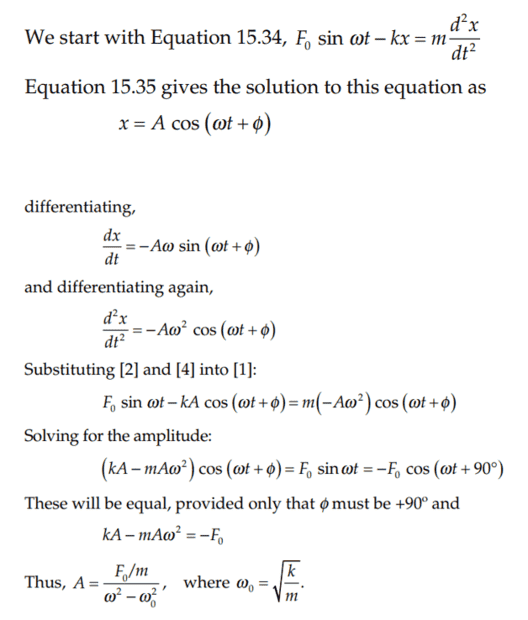
However, can someone please explain how this is showing equation 15.35 as a solution of equation 15.34? I though both sides should be equal without assuming that $\phi = 90$

Also why are they allowed to assume $\phi = 90$?

Many thanks!

 

[haruspex]

Given 15.34, we want to see whether an equation of the form 15.35 can be a solution if we plug in suitable values for $A, \omega, \phi$.
To check this, we use 15.35 to substitute for x in 15.34.
It turns out that 15.34 is satisfied by 15.35 provided $\phi=\pi/2+2n\pi$ and $A=\frac{F_0}{m\omega^2-k}$.

 

[member 731016]

Given 15.34, we want to see whether an equation of the form 15.35 can be a solution if we plug in suitable values for $A, \omega, \phi$.
To check this, we use 15.35 to substitute for x in 15.34.
It turns out that 15.34 is satisfied by 15.35 provided $\phi=\pi/2+2n\pi$ and $A=\frac{F_0}{m\omega^2-k}$.

Thank you for your reply @haruspex !

 

[hutchphd]

and any solution to the unforced (or homogeneous or $F_0=0$) system can be added to produce another solution as dictated by initial conditions.

 

[member 731016]

and any solution to the unforced (or homogeneous or $F_0=0$) system can be added to produce another solution as dictated by initial conditions.

Thank you @hutchphd , that is good to know!

 

[DaveE]

I though both sides should be equal without assuming that ϕ=90

Also why are they allowed to assume ϕ=90?

Φ and A were not defined initially. They were testing a function to see what would be required for it it satisfy the original equation. Then they derived what Φ and A had to be for that form to be correct.

 

[member 731016]

Φ and A were not defined initially. They were testing a function to see what would be required for it it satisfy the original equation. Then they derived what Φ and A had to be for that form to be correct.

Oh ok thank you @DaveE that makes more sense now!