- #1
patric44
- 308
- 40
- Homework Statement
- some questions about the derivation of Bloch theorem
- Relevant Equations
- in the attachments
hi guys
our solid state professor gave us a series of power point slides that contains the derivation of Bloch theorem , but some points is not clear to me , and when i asked him his answer was also not clear :
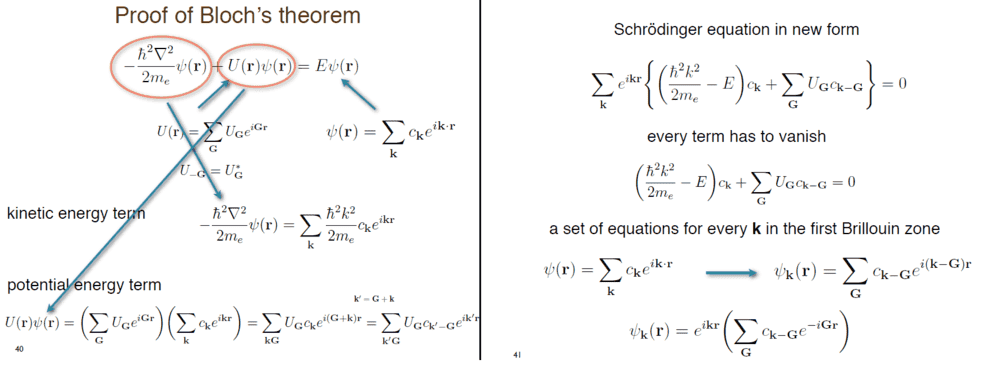
in the first part i understand the he represented both the potential energy and the electron plane wave as a Fourier series
but when he multiplied both together in the last equation he introduced k' why is that ! sinse k=k+G as it wil repreat in the next parabolic dispersion and subsequently he changed the index of Ck ⇒ Ck'-G isn't that also k ?
in the next page he set again k=k+g and took the exponential as a common factor but yet leaves the "C" coefficent as k-G
why he keep alternating between k'-G , k ...
and the jump from
$$ Ψ(r) ⇒Ψk(r) $$
is not very clear to me ?
- and how i suppose to solve this Schrodinger equation i mean its no longer a differential eq ?
our solid state professor gave us a series of power point slides that contains the derivation of Bloch theorem , but some points is not clear to me , and when i asked him his answer was also not clear :
in the first part i understand the he represented both the potential energy and the electron plane wave as a Fourier series
but when he multiplied both together in the last equation he introduced k' why is that ! sinse k=k+G as it wil repreat in the next parabolic dispersion and subsequently he changed the index of Ck ⇒ Ck'-G isn't that also k ?
in the next page he set again k=k+g and took the exponential as a common factor but yet leaves the "C" coefficent as k-G
why he keep alternating between k'-G , k ...
and the jump from
$$ Ψ(r) ⇒Ψk(r) $$
is not very clear to me ?
- and how i suppose to solve this Schrodinger equation i mean its no longer a differential eq ?