Why is my derivation of the catenary wrong?

In summary, there could be several reasons for incorrect results in a catenary derivation, such as using the wrong equation or errors in calculation. To check accuracy, compare results with known values or try different methods. The key assumptions in the derivation are a perfectly elastic, inextensible, and uniform cable under gravity with negligible weight. The catenary equation cannot be used to model other shapes and has real-world applications in structures and systems like bridges and power lines.
  • #1
phantomvommand
272
39
TL;DR Summary
I have "derived" a differential equation for the catenary, and have attached my working. It looks slightly different from the correct expression, which can be found here: https://www.math24.net/equation-catenary

Please do tell me where I made a mistake. Thank you!
Important note: I only derived the differential equation, I did not solve it.

WhatsApp Image 2021-03-04 at 1.22.37 AM.jpeg

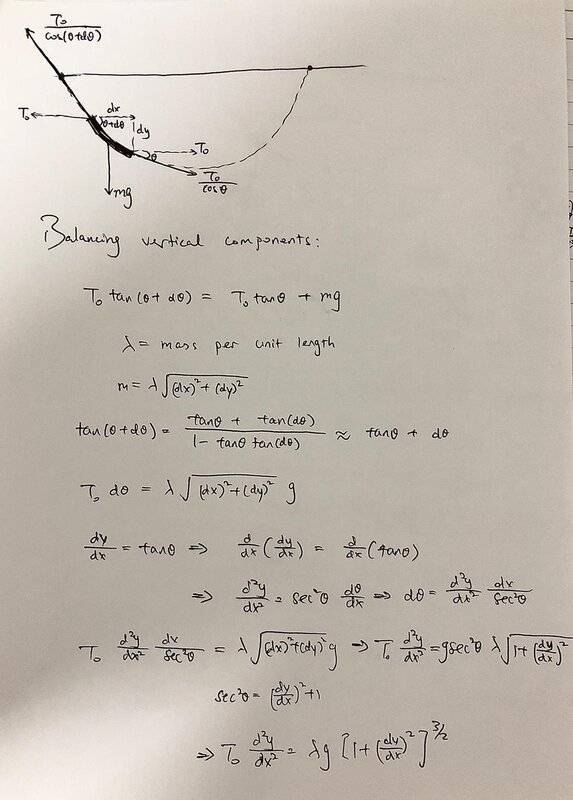
What I think caused the mistake:
- the tangent approximation (tan(theta+dtheta) ~ tan theta + d theta
 
Physics news on Phys.org
  • #2
Hi,

phantomvommand said:
What I think

That's what I think too :wink:
The proper way to differentiate ##\tan\theta## is not ##{d\tan\theta\over d\theta} = 1 ## but $${d\tan\theta\over d\theta} = {d\over d\theta}\Biggl ( {\sin\theta\over\cos\theta}\Biggr ) =\ ... $$
​
##\ ##​
 
  • Like
Likes vanhees71

FAQ: Why is my derivation of the catenary wrong?

Why does my catenary derivation not match the expected shape?

The catenary curve is a mathematical shape that is formed by a hanging chain or cable under its own weight. It is not a perfect parabola, as many people assume, but rather a hyperbolic cosine function. If your derivation does not match this shape, it is likely due to an error in your calculations or assumptions.

What are some common mistakes made in deriving the catenary?

One common mistake is assuming that the catenary is a parabolic curve. Another is neglecting the weight of the chain itself, which can significantly affect the shape of the curve. Additionally, using incorrect or incomplete equations can also lead to incorrect results.

How do I account for the weight of the chain in my derivation?

In order to accurately derive the catenary curve, you must include the weight of the chain in your calculations. This can be done by using the appropriate equations for the tension and weight of the chain at different points along the curve.

Can I use a different method to derive the catenary?

Yes, there are multiple methods for deriving the catenary curve, including using differential equations, calculus, and the principle of virtual work. However, each method requires a solid understanding of the underlying principles and equations involved.

What real-world applications does the catenary have?

The catenary curve has many practical applications, including in architecture, engineering, and physics. It is used in the design of arches, bridges, and suspension cables, as well as in understanding the shape of hanging chains and ropes. It also has applications in fields such as biology and economics.

Similar threads

I What is the Unsolvable Catenary Problem?
Replies
17
Views
3K
B Sign conventions in torque and non-uniform circular motion
Replies
1
Views
717
I Transformations for isotropy in terms of math
Replies
1
Views
799
I Why isn't the Lagrangian invariant under ##\theta## rotations?
Replies
5
Views
1K
I Mathematical disagreement with inverse square law?
Replies
33
Views
796
I What assumption is incorrect in the derivation of the catenary curve equation?
Replies
15
Views
3K
Calculation of Thomas Precession in Rindler's relativity book
Replies
6
Views
1K
I My textbook is deriving wave speed on a string under tension
Replies
19
Views
2K
I Lacking intuition with partial derivatives
Replies
18
Views
1K
I Missing exponent in "Theoretical Minimum"?
Replies
4
Views
1K

Hot Threads

Recent Insights

Back
Top