Why is My Second-Order Reaction Rate Calculation Incorrect?

[guiromero]

Homework Statement

Butadiene can be react with itself to form a dimer molecule. The reaction is second order in butadiene. The value of the rate constant is 7.7 x 10-3 M-1 s-1.

What is the initial rate consumption of butadiene in a reactor filled with butadiene to a concentration of 3.091 M? Express your answer in M/s.

Relevant Equations

r = k*C^2

Hello,

I have a doubt in an exercise about rate of reaction. The statement is quoted above and the reaction scheme is the following:

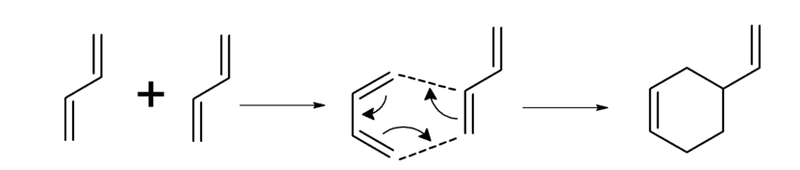
As the reaction is second order, I tried to apply the formula r = k*C^2.

Where r = rate of reaction
k = rate constant
C = concentration

So, I got:
r = (7.7e-3)*(3,091)^2 = 0,073 M

However, this not correct. I know it because when I click the "Send answer" button, it says "incorrect". Furthermore, the "Show answer" button doesn't display the answer.

Could anyone give some help?

Thanks a lot.

 

[DrClaude]

So, I got:
r = (7.7e-3)*(3,091)^2 = 0,073 M

Is this result properly rounded? (Also, careful with the units.)

 

[guiromero]

I tried to round from 0.07 to 0.075 and it didn't work.
The units are ok, as k[M^-1*s^-1] * C^2[M^2] = r[M * s^-1]

 

[DrClaude]

I tried to round from 0.07 to 0.075 and it didn't work.

In my calculator,
$$
7.7 \times 10^{-3} (3.091)^2 = 0.073567963
$$
which, rounding to two significant figures, should give 0.074. You might try also 0.0736 or 0.07357 in case the preprogrammed solution was entered with an incorrect number of significant figures.

The units are ok, as k[M^-1*s^-1] * C^2[M^2] = r[M * s^-1]

It's just that you wrote the units as M instead of M/s in the OP.

 

[guiromero]

In my calculator,
$$
7.7 \times 10^{-3} (3.091)^2 = 0.073567963
$$
which, rounding to two significant figures, should give 0.074. You might try also 0.0736 or 0.07357 in case the preprogrammed solution was entered with an incorrect number of significant figures.It's just that you wrote the units as M instead of M/s in the OP.

I tried the values that you mentioned but it didn't work.
I think what you trying to say is the units of rate. I put M*s^-1 for M/s. I don't know if this is what you mean.

 

[DrClaude]

So, I got:
r = (7.7e-3)*(3,091)^2 = 0,073 M

You wrote M instead of M s^-1.

 

[guiromero]

You wrote M instead of M s^-1.

Oh yes, thanks.