- #1
Happiness
- 695
- 31
Why is there no virtual work done by a rolling friction?
In pure rolling, is the virtual displacement parallel or perpendicular to the surface? I believe it can't be perpendicular because the object is not allowed to lose contact with the surface. But if it's parallel, then there must be a non-zero virtual work done since the rolling friction is also parallel to the surface. The book says the point of contact is momentarily at rest, does that mean that the virtual displacement must be zero (but the actual infinitesimal displacement is parallel to the surface)?
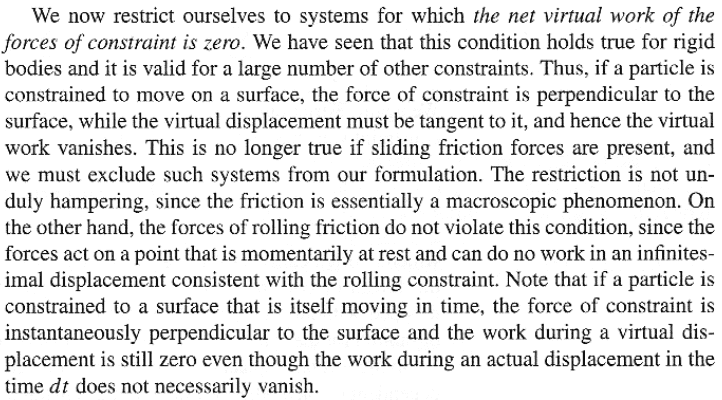
In pure rolling, is the virtual displacement parallel or perpendicular to the surface? I believe it can't be perpendicular because the object is not allowed to lose contact with the surface. But if it's parallel, then there must be a non-zero virtual work done since the rolling friction is also parallel to the surface. The book says the point of contact is momentarily at rest, does that mean that the virtual displacement must be zero (but the actual infinitesimal displacement is parallel to the surface)?
Last edited: