PainterGuy
- 938
- 73
Hi,
Could you please have a look on the attachment?
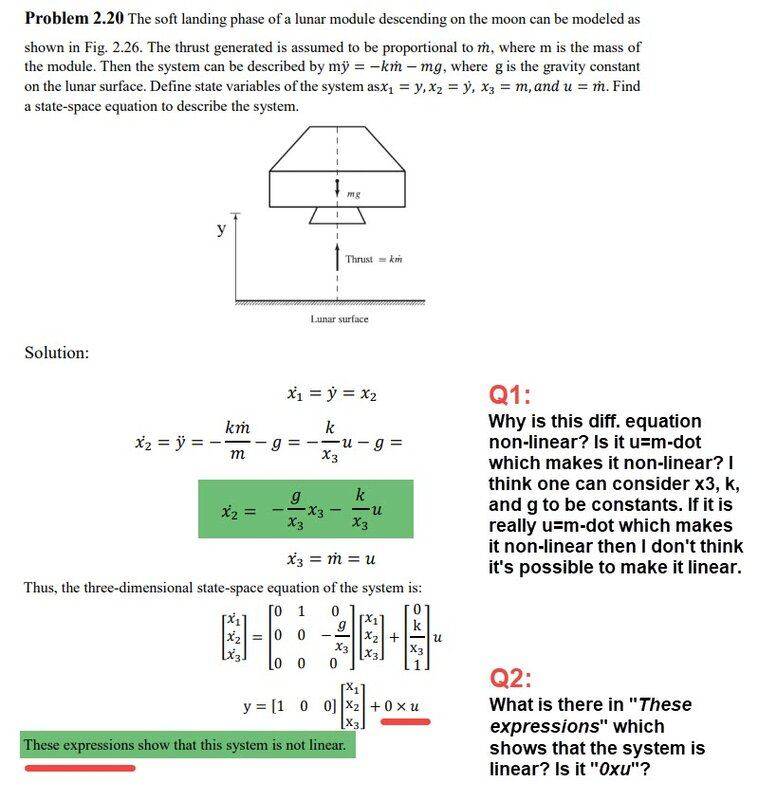
Question 1:
Why is this differential equation non-linear? Is it u=\overset{\cdot }{m} which makes it non-linear?
I think one can consider x_{3} , k, and g to be constants. If it is really u=\overset{\cdot }{m} which makes it non-linear then I don't think it's possible to make it linear. Could you please correct me?
Question 2:
What is there in "These expressions" which shows that the system is linear? Is it "0 x u"?
Could you please have a look on the attachment?
Question 1:
Why is this differential equation non-linear? Is it u=\overset{\cdot }{m} which makes it non-linear?
I think one can consider x_{3} , k, and g to be constants. If it is really u=\overset{\cdot }{m} which makes it non-linear then I don't think it's possible to make it linear. Could you please correct me?
Question 2:
What is there in "These expressions" which shows that the system is linear? Is it "0 x u"?
Last edited by a moderator: