simphys
- 327
- 46
- Homework Statement
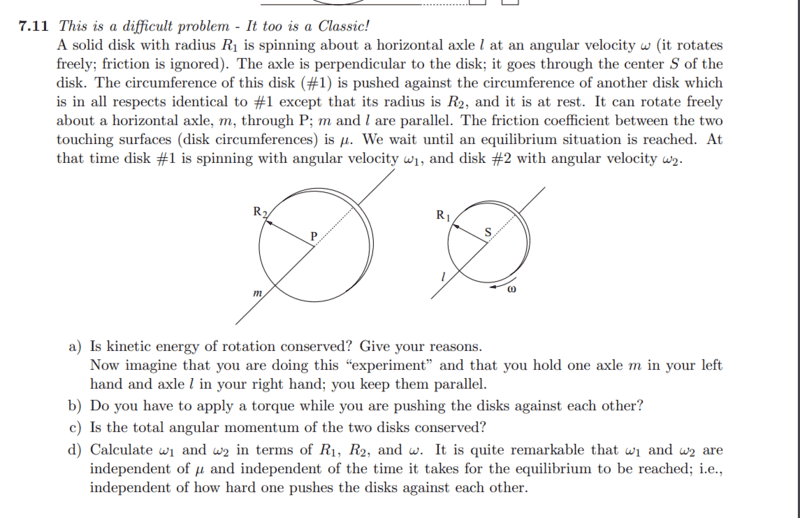
- please check the screenshot.
I have two questions: which don't need any calculation yet:
1. Why is angular momentum of the two disks not conserved?? The friction between is internal no? In the solution it said that there is ang. momentum conserved (neither kinetic of course)
2. What is actually meant with 'an equilibrium situation reached'?
- Relevant Equations
- thanks in advance.
Here is question + drawing.