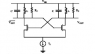
- #1
anhnha
- 181
- 1
I am wondering why LC tank only oscillates at resonant frequency not other frequencies?
Is there a physics explanation for that?
Is there a physics explanation for that?