Why must we use integral to find the resultant force?

Join the discussion
Registration is free. Start your own thread to ask a follow-up.
Sign up Log in
4 replies · 3K views · last reply
EastWindBreaks
Messages
128
Reaction score
3

Homework Statement


Hydrostatic force on a plane surface ex:
Diagram of a rotated inclined plane gate under hydrostatic pressure, showing red pressure arrows, depths h and hc, centroid C and center of pressure CP, with the resultant force equations and red-boxed result FR = gamma sin(theta) yC A = gamma hC A = PC A.


Hydrostatic force on a gate:
Plan view diagram of a lock channel 34 m wide with water on the left, showing a pair of gates meeting at a center point, Gate A hinged at the channel wall, angled 15 degrees, with hinge and width labeled.


Homework Equations



The Attempt at a Solution


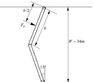
why can't we just use the formula in the red box above for problem 3.57?
instead I have to use integral
Equation F_R equals the integral from 0 to D of p dA, with the integral sign's limits labelled 0 and D.

Side view of a hinged gate in water, with a horizontal water surface line at top, a dimension b along the gate and b/2 to its upper point, a force arrow F_R acting on the upper section, a 15 degree angle marked at the lower segment, and a vertical dimension W = 34m from the surface to the bottom.

Handwritten derivation of hydrostatic force F_R as the integral from 0 to D of gamma h times W over 2 cos 15 degrees dh, reducing to gamma W D squared over 4 cos 15 degrees; W indicates channel width.

I am confused, how does this gate different from a slanted plane. if I look at the gate from front view, i feel like they are just the same thing. How does
Equation F_R = integral from 0 to D of p dA, the hydrostatic resultant force on a plane surface as an integral over its area.
a double integral with respect to area (dhdb) became a single integral with respect to dh?
 
Attachments
  • Plan view diagram of a lock channel 34 m wide with water on the left, showing a pair of gates meeting at a center point, Gate A hinged at the channel wall, angled 15 degrees, with hinge and width labeled.
    upload_2017-11-13_3-12-35.png
    28.4 KB · Views: 1,124
  • Diagram of a rotated inclined plane gate under hydrostatic pressure, showing red pressure arrows, depths h and hc, centroid C and center of pressure CP, with the resultant force equations and red-boxed result FR = gamma sin(theta) yC A = gamma hC A = PC A.
    upload_2017-11-13_3-15-19.png
    28.1 KB · Views: 1,379
  • Handwritten derivation of hydrostatic force F_R as the integral from 0 to D of gamma h times W over 2 cos 15 degrees dh, reducing to gamma W D squared over 4 cos 15 degrees; W indicates channel width.
    upload_2017-11-13_3-17-14.png
    6.3 KB · Views: 666
  • Side view of a hinged gate in water, with a horizontal water surface line at top, a dimension b along the gate and b/2 to its upper point, a force arrow F_R acting on the upper section, a 15 degree angle marked at the lower segment, and a vertical dimension W = 34m from the surface to the bottom.
    upload_2017-11-13_3-17-44.png
    15.3 KB · Views: 655
  • Equation F_R equals the integral from 0 to D of p dA, with the integral sign's limits labelled 0 and D.
    upload_2017-11-13_3-38-14.png
    932 bytes · Views: 574
  • Equation F_R = integral from 0 to D of p dA, the hydrostatic resultant force on a plane surface as an integral over its area.
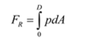    upload_2017-11-13_3-38-24.png
    932 bytes · Views: 592
Last edited:
Physics news on Phys.org

Discussion

oh! I can use it but because its the top view, we have to use 5 meter for h_c, which is the depth that affects the pressure.
 
ok, I have one question left, how does
Equation F_R = integral from 0 to D of p dA, showing the resultant force as an integral of pressure over area.
a double integral with respect to area (dhdb) became a single integral with respect to dh?
 
Attachments
  • Equation F_R = integral from 0 to D of p dA, showing the resultant force as an integral of pressure over area.
    upload_2017-11-13_3-38-24-png.png
    932 bytes · Views: 552
EastWindBreaks said:
double integral with respect to area (dhdb) became a single integral with respect to dh
Because the width (the b variable in dbdh) is constant.
EastWindBreaks said:
how does this gate different from a slanted plane.
It doesn't really. Just bear in mind that the gates are vertical, so in the equation in the red box you would put θ=π/2. On the other hand, the area is not WD. To express the answer in terms of W and D you need to make reference to the 15° angle.
With those adjustments, I think you will find the two expressions are the same.
 
  • Like
Likes   Reactions: EastWindBreaks
haruspex said:
Because the width (the b variable in dbdh) is constant.

It doesn't really. Just bear in mind that the gates are vertical, so in the equation in the red box you would put θ=π/2. On the other hand, the area is not WD. To express the answer in terms of W and D you need to make reference to the 15° angle.
With those adjustments, I think you will find the two expressions are the same.
oh yeah, I got it now, thank you!
 

Similar threads

Why must we use absolute temperature for the Ideal Gas Law?
  • member 731016
  • · Replies 10 ·
  • Introductory Physics Homework Help
Replies
10
Why Must We Use Absolute Temperature for Ideal Gas Law?
  • jack1234
  • · Replies 2 ·
  • Introductory Physics Homework Help
Replies
2
Undergrad Why Do We Use Integrating Factors in Solving First Order Linear ODEs?
  • Saladsamurai
  • · Replies 4 ·
  • Differential Equations
Replies
4