abdossamad2003
- 68
- 4
Why is this equation (red sign) written in constant volume and not in constant pressure?
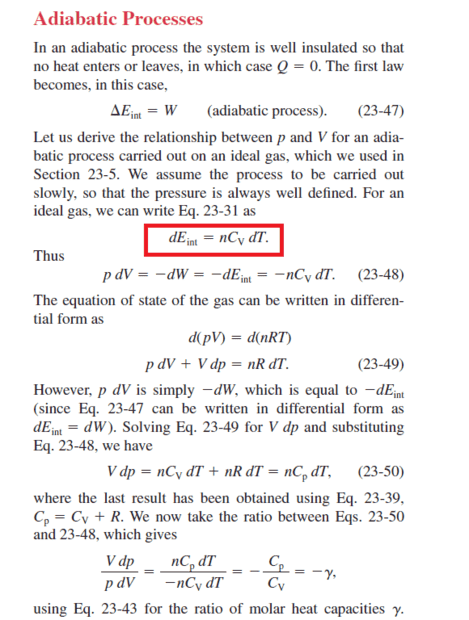
This is totally incorrect. Irrespective of the process, the internal energy of an ideal gas depends only on temperature, and not volume.abdossamad2003 said:The effect of volume on the internal energy is meaningful only in diabatic processes, for example, when heat is added to the system at a constant volume and the internal energy increases, but in adiabatic processes, when the volume is constant, the work done on the system is zero and the incoming heat is zero, as a result of the change in energy Internal is zero.
See, e.g., here: https://en.wikipedia.org/wiki/Internal_energy#Internal_energy_of_the_ideal_gas. ##C_V## is the coefficient of proportionality between internal energy on one hand and number of moles and temperature of the gas on the other hand.abdossamad2003 said:I was not convinced why internal energy should be written for heat capacity in constant volume. This process does not take place in constant volume, and if it is in constant volume, the change in internal energy must be zero.