A Wilson Line Propagator: Understanding Eqtn 5.7 & 5.8

[Elmo]

TL;DR Summary

An undefined term in the wilson line propagator

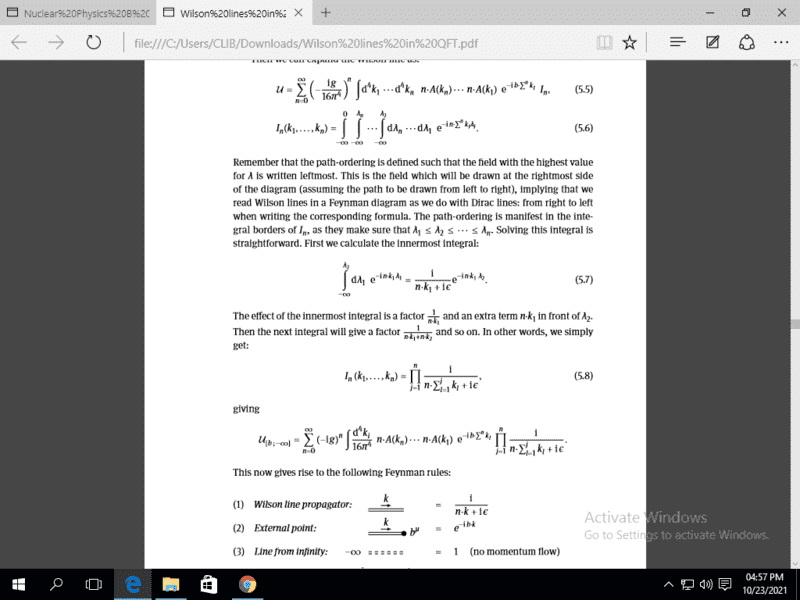
I asked this from a number of people but no one knew what to do about this exponential with iota infinity in the power,in Eqtn 5.7
The textbook seems to imply that it is zero but cos and sine are undefined at infinity.
Also,all the exponentials seem to vanish from the final result of Eqtn 5.8 whereas the integration of exponential function should still leave behind the function.
Where do all the exponentials go ?

 

[dRic2]

I think the author intoduces a small variable $\epsilon > 0$ so to write the integral in 5.7 as:

$$\lim_{\epsilon \rightarrow 0^+} \int_{-\infty}^{\lambda_2} d\lambda e^{-i(nk + i \epsilon)\lambda}$$

In this way you will have your original exponential multiplied by $e^{\epsilon \lambda}$. This will assure the convergence of the function as $\lambda$ goes to $-\infty$ because it will exponentially go to zero.
The result of the integral is to be understood with a $\lim_{\epsilon \rightarrow 0^+}$ in front.

 

[Elmo]

I think the author intoduces a small variable $\epsilon > 0$ so to write the integral in 5.7 as:

$$\lim_{\epsilon \rightarrow 0^+} \int_{-\infty}^{\lambda_2} d\lambda e^{-i(nk + i \epsilon)\lambda}$$

In this way you will have your original exponential multiplied by $e^{\epsilon \lambda}$. This will assure the convergence of the function as $\lambda$ goes to $-\infty$ because it will exponentially go to zero.
The result of the integral is to be understood with a $\lim_{\epsilon \rightarrow 0^+}$ in front.

Seems good though it introduces another undefined term as the lower limit, in the form of
$$e^{-n\epsilon\infty} $$
And as $\epsilon \rightarrow 0$ is being multiplied with infinity ,should that not again be undefined ?
Also,my other question remains that what happens to the exponentials in the upper limit ?
Why don't they show up in the final expression or the list of Feynman rules.

 

[dRic2]

should that not again be undefined

No. You take the $\lim_{\epsilon->0^+}$ at the end of all the calculations. So, first, you do the definite integral (that is first you take the limit as $\lambda \rightarrow - \infty$ which annihilates the exponential).

As for your second question, I don't see it immediately. If I have time I'll check more carefully. Sorry

 

[Dr.AbeNikIanEdL]

Also,my other question remains that what happens to the exponentials in the upper limit ?

You are performing the integrals in (5.6) one after the other. The explicit example is for the innermost one (over $\lambda_1$). Have you verified the claim about the $\lambda_2$ integral? The upper limit in the last integral (the leftmost integral symbol) is 0, giving you $e^0 = 1$.