Word Problem for slope intercept graph equation

[mhester88]

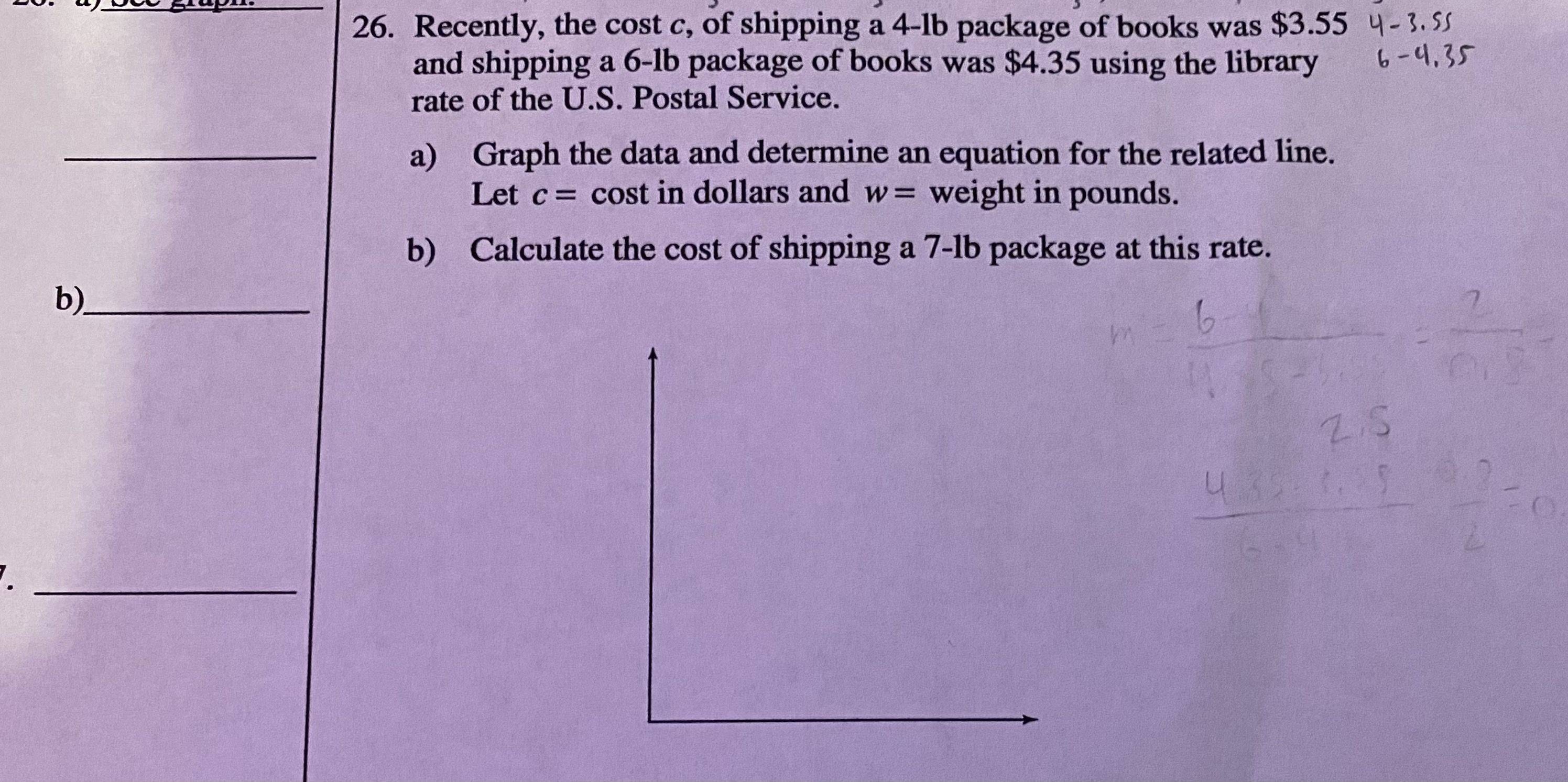
I have this word problem that is asking for two different answers, the equation for the data and to calculate the shipping rate. I'm not understanding how to address either of the questions. Will someone please help me with this answer?

 

[skeeter]

shipping cost is dependent on weight

an ordered pair for the cost function would be $(w, c)$ where $w$ is the weight (the independent variable), and $c$ is the cost (the dependent variable

you are given two such ordered pairs, $(4, 3.55)$ and $(6, 4.35)$

find the slope between those two given points, then use the point-slope form of a linear equation to get the cost function

 

[mhester88]

Thank you for your response. If I'm understanding this correctly, then the slope would be 0.4. I'm still confused on how to write the equation using c and w. I'm not sure how to even begin creating the equation.

 

[skeeter]

Thank you for your response. If I'm understanding this correctly, then the slope would be 0.4. I'm still confused on how to write the equation using c and w. I'm not sure how to even begin creating the equation.

point-slope form of a linear equation ...

$y - y_1 = m(x - x_1)$

where $(x_1,y_1)$ is a point on the line and $m$ is the slope

... requires one point (you have two), and the slope, $m$.

remember, y is the cost (you can use c instead) and x is the weight (you can use w instead)

$c - c_1 = m(w - w_1)$

 

[HOI]

Any (non-vertical) line has equation c= aw+ b for some numbers, a and b. You are told that when the weight, w, is 4 lb. the cost, c, is \$3.55 so 3.55= a(4)+ b. You are told that when the weight, w, is 6 lb. the cost, c, is \$4.35 so 4.35= a(6)+ b.

Solve the two equations, 4a+ b= 3.55 and 6a+ b= 4.35, for a and b. I recommend you subtract the first equation from the second to eliminate b.