Work done on a pendulum by gravity

[simphys]

Homework Statement

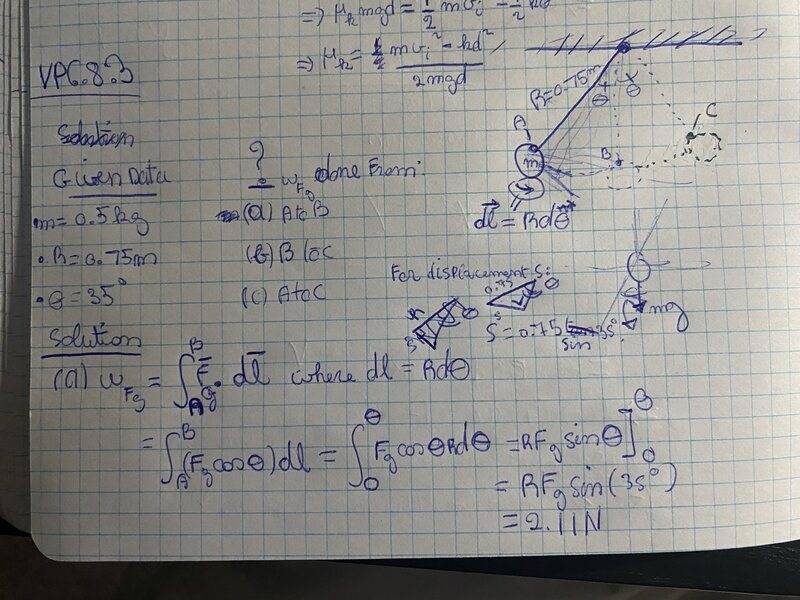
VP6.8.3
A pendulum is made up of a small sphere of mass 0.500 kg
attached to a string of length 0.750 m. The sphere is swinging back
and forth between point A, where the string is at the maximum angle of
35.0° to the left of vertical, and point C, where the string is at the maximum angle of 35.0° to the right of vertical. The string is vertical when
the sphere is at point B. Calculate how much work the force of gravity
does on the sphere (a) from A to B, (b) from B to C, and (c) from A to C.

Relevant Equations

work done: w = mgd, d=displacement or line integral of work

Hello guys, I was wondering if someone could provide me some help on this problem.
for (c), I know that it will be 0 as the amount of word done from A to B = the am of work done from B to C.

But, What I receive as seen in the picture is 2.11N Which is not correct..
In the first try I used a line integral because I thought of gravity being a variable force but it is actually the angle that varies as it is a curved path. But that doesn't matter as the force is constant and no matter the path, if there is a constant force we use W = mgd (if I'm not mistaking)

but... once again, I get 2.11N whilst the answer is 0.6 something N

Can someone tell me what I am doing wrong please?
Thanks in advance!

 

[PeroK]

You have a couple of mistakes. The component of the force along the line of motion is $\sin \theta$. And, using your diagram $dl = -R d\theta$. I.e. as the pendulum moves from left to right the angle decreases.

 

[simphys]

You have a couple of mistakes. The component of the force along the line of motion is $\sin \theta$. And, using your diagram $dl = -R d\theta$. I.e. as the pendulum moves from left to right the angle decreases.

may I ask why it is $-R d /theta$?, the angle is decreasing relative to the vertical then? (I get that at the end we get the - sign removed that is created from the integral, but don't get why we use the - sign with $d$ theta and oh yes ... thank you!

Edit:
Another question, is there a way to do this without using a line integral? as it is a constant force, or not, because the path is curved? I was kind of confused on this one as on one of the examples it only utilized$W = Fd cos/theta$

 

[PeroK]

Another question, is there a way to do this without using a line integral? as it is a constant force, or not, because the path is curved? I was kind of confused on this one as on one of the examples it only utilized$W = Fd cos/theta$

Yes, use the work-energy theorem.

 

[simphys]

Yes, use the work-energy theorem.

so for that question: only line integral is possible
and for the $-rd theta$ that's because the angle decreases relative to the vertical?

(a yes - yes is sufficient :D Thanks a lot Perok!)

 

[PeroK]

so for that question: only line integral is possible
and for the $-rd theta$ that's because the angle decreases relative to the vertical?

You chose to have the angle $\theta$ going from $+35$ degrees on the left to $-35$ degrees on the left and the pendulum bob moving from left to right (with $l$ increasing from left to right). That decision results in $\theta$ decreasing as $l$ increases, hence $d\theta = - Rdl$.

If you had chosen the opposite convention for $\theta$ or had the bob moving from right to left then you would have had $d \theta = Rdl$.

 

[kuruman]

Another question, is there a way to do this without using a line integral?

A line integral is your friend. If you do it formally, you can't go wrong. Here is what I mean using this particular problem as an example.
1. Write the position of the displaced object in Cartesian coordinates.
$\vec l=l(1-\cos\theta)~\hat x+l\sin\theta~\hat y$
Note: I chose the origin at the lowest point of the motion.

2. Find element $d\vec l$ in Cartesian coordinates.
$d \vec l=l\sin\theta ~d\theta~\hat x+l\cos\theta ~d\theta~\hat y.$

3. Write the force vector in Cartesian coordinates and take the dot product.
$\vec F=-mg~\hat y$
$\vec F \cdot d \vec l=(-mg~\hat y)\cdot (l\sin\theta~ d\theta~\hat x+l\cos\theta~ d\theta~\hat y)=-mgl\cos\theta~ d\theta.$

4. Do the integral
$$\int_{1}^{2}\vec F \cdot d \vec l=-mgl\int_{1}^{2}\cos\theta~ d\theta=-mgl(\sin\theta_2-\sin\theta_1).$$ 5. Substitute values for the starting and ending angles.

This method works with all line integrals whether the work-energy theorem is applicable or not. Note that the limits of integration take care of the sign of the final result. You don't have to worry about whether the angle is increasing or decreasing or whether $\vec F$ is parallel or antiparallel to $d\vec l$. Just be sure to start with $d\vec l=dx~\hat x+dy~\hat y+dz~\hat z$ with no negative signs anywhere; they are automatically taken care of by the limits of integration.

 

[Steve4Physics]

... is there a way to do this without using a line integral? as it is a constant force, or not, because the path is curved? I was kind of confused on this one as on one of the examples it only utilized$W = Fd cos/theta$

Work done by the force of gravity = -(change in gravitational potential energy)