- #1
otownsend
- 12
- 0
Member advised to use the formatting template for all homework help requests
My question unfortunately does not fit in the Homework template, so I hope this is okay.
I attached a question below which involves multiple parts using the concepts related to energy, work, impulse, and momentum. Under the "Simplify and Diagram" section (you will notice it is in bold text), I want to better understand why Part II cannot be understood with a work-energy bar chart. The texts reasoning for why it is not a good idea to use a work-energy bar charts is because "we don't have a way to independently determine internal energy change". Why is this the reason? Isn't the reason more that we want to find the velocity of Jane and Spiderman after the collision occurred and using a work-energy expression (in this case the work energy expression would be something like kinetic energy = change in internal energy) wouldn't allow us to determine this velocity?
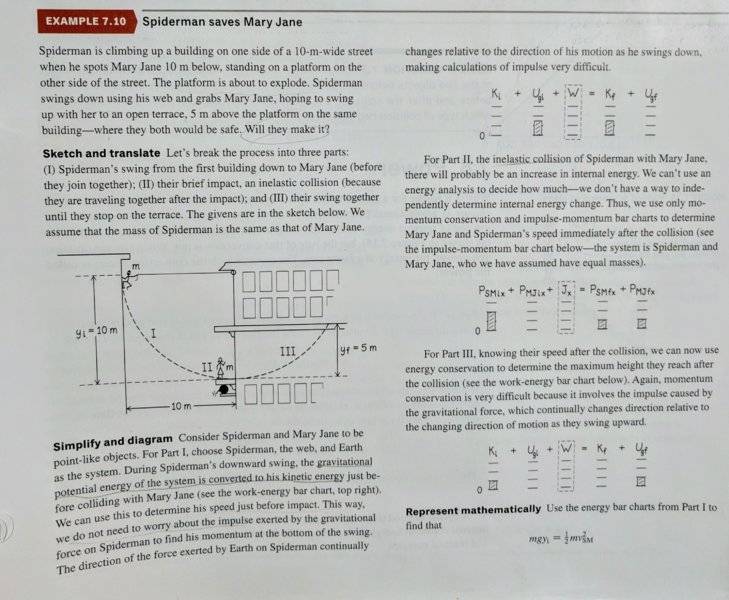

I attached a question below which involves multiple parts using the concepts related to energy, work, impulse, and momentum. Under the "Simplify and Diagram" section (you will notice it is in bold text), I want to better understand why Part II cannot be understood with a work-energy bar chart. The texts reasoning for why it is not a good idea to use a work-energy bar charts is because "we don't have a way to independently determine internal energy change". Why is this the reason? Isn't the reason more that we want to find the velocity of Jane and Spiderman after the collision occurred and using a work-energy expression (in this case the work energy expression would be something like kinetic energy = change in internal energy) wouldn't allow us to determine this velocity?
Attachments
Last edited:
