- #1
Kermit_the_Phrog
- 22
- 3
- Homework Statement
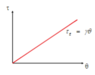
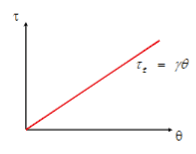
- As a nut is tightened through an angle θ , the friction between the nut and the threads on the bolt increases. This causes an increasing frictional torque, Tf = γθ as illustrated. The maximum forces, F, you can apply to the wrench gives a maximum torque Tw. What is the total work you do in tightening the nut?
A. Twθ
B. Tw (Tf/y)
C. (Tw^2)/(2y)
D. (Tw^2)/y
- Relevant Equations
- W = T θ
NOTE: THIS IS THE GRAPH PROVIDED
 At first I approached this problem attempting to solve for the total work done, using the formula
At first I approached this problem attempting to solve for the total work done, using the formula
Wtotal = Wmaxforce - Wfriction
I then subbed into the formula, representing the values of work as their torque value times theta, which gave me a longer algebraic solution that was not one of the multiple choice options listed.
ie,
Wtotal = Twθ - Tfθ
= Twθ - (Yθ)θ
= θ (Tw- yθ)
(this is very clearly wrong)
However, now that I've thought about it, the question is phrased in a specific way. It never really asks for the total work, only for the work that YOU do while tightening the nut :
So would it just be
Wmaxforce = T θ
= Tw θ
and thus option A?
Or is the real solution closer to my first approach?
NOTE: i am not really given the final answer so unfortunately I have no idea which final multiple choice answer is correct ¯\_(ツ)_/¯
Thanks in advance!
Wtotal = Wmaxforce - Wfriction
I then subbed into the formula, representing the values of work as their torque value times theta, which gave me a longer algebraic solution that was not one of the multiple choice options listed.
ie,
Wtotal = Twθ - Tfθ
= Twθ - (Yθ)θ
= θ (Tw- yθ)
(this is very clearly wrong)
However, now that I've thought about it, the question is phrased in a specific way. It never really asks for the total work, only for the work that YOU do while tightening the nut :
So would it just be
Wmaxforce = T θ
= Tw θ
and thus option A?
Or is the real solution closer to my first approach?
NOTE: i am not really given the final answer so unfortunately I have no idea which final multiple choice answer is correct ¯\_(ツ)_/¯
Thanks in advance!